vector

372 Vectors 

5 

Vectors 

5.1 VECTORS 

A vector is a quantity having both magnitude and direction such as force, velocity 

acceleration, displacement etc. 

5.2 ADDITION OF VECTORS 

Let a and b be two given vectors 

OA 

= a and AB 

= b then vector OB 

 

is called the 

sum of a and b . 

Symbolically 

— — 

OA AB = OB — 

 

a b = OB — 

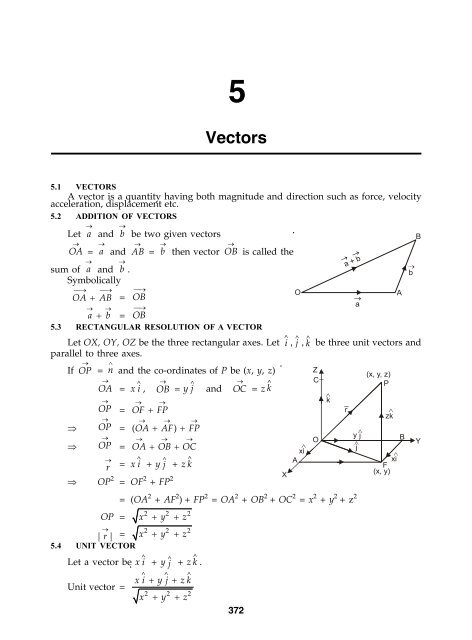
5.3 RECTANGULAR RESOLUTION OF A VECTOR 

Let OX, OY, OZ be the three rectangular axes. Let î , ^j , ^k be three unit vectors and 

parallel to three axes. 

If OP 

= n and the co-ordinates of P be (x, y, z) Z 

OA 

= x î , OB 

= y ^j and OC 

 

= z ^k 

(x, y, z) 

C 

P 

 

 

OP 

 

= OF FP 

OP 

 

= ( OA AF) 

FP 

OP 

 

= OA OB OC 

 

r 

= x î + y ^j + z ^k 

OP 2 = OF 2 + FP 2 

= (OA 2 + AF 2 ) + FP 2 = OA 2 + OB 2 + OC 2 = x 2 + y 2 + z 2 

2 2 2 

OP = x y z 

 

2 2 2 

| r | = x y z 

5.4 UNIT VECTOR 

Let a vector be x î + y ^j + z ^k . 

Unit vector = 

^ ^ ^ 

xi yj 

zk 

2 2 2 

x y z 

372 

X 

O 

 

xi 

A 

O 

 

k 

– r 

a + b 

a 

y j 

 

j 

zk 

A 

 

xi 

F 

(x, y) 

B 

b 

B 

Y

Vectors 373 

Example 1. If a and b be two unit vectors and be the angle between them, then find 

the value of such that a + b is a unit vector. (Nagpur, University, Winter 2001) 

Solution.Let 

 

OA = a be a unit vector and 

be the angle between a and b . 

If 

 

OB = c = a + b is also a unit vector then, we have 

 

| OA | = 1 

 

| OB | = 1 

 

| OB | = 1 

OAB is an equilateral triangle. 

Hence each angle of OAB is 3 

5.5 POSITION VECTOR OF A POINT 

 

AB = b is another unit vector and 

Ans. 

The position vector of a point A with respect to origin O is the vector OA which is 

used to specify the position of A w.r.t. O. 

— 

To find AB if the position vectors of the point A and point B are given. 

If the position vectors of A and B are a and b . Let the origin be O. 

Then 

 

 

= 

OA 

 

a, 

OB b 

 

OA AB = OB 

AB 

 

= OB OA 

AB 

= b 

 

O 

a 

AB 

 

= Position vector of B – Position vector of A 

Example 2. If A and B are (3, 4, 5) and (6, 8, 9), find AB 

Solution. 

AB 

 

. 

= Position vector of B – Position vector of A 

= (6 iˆ 8 ˆj 9 kˆ) (3î 4ˆj 5 kˆ) 

= 3iˆ 

4 ˆj 4 kˆ 

Ans. 

5.6 RATIO FORMULA 

To find the position vector of the point which divides the line joining two given 

points. 

Let A and B be two points and a point C divides AB in the ratio of m : n. 

Let O be the origin, then 

OA 

= 

 

A 

m C n B 

a , and OB b, OC ? (a) 

(b) 

 

 

OC 

= OA AC 

 

m 

m 

= OA AB AC 

AB 

m n m n 

m 

 

= a .( b a ) ( AB b a) 

m n 

O 

a 

A 

 

c 

a + b = c 

 

B (b) 

O 

b 

B 

A (a)

374 Vectors 

OC 

= 

 

m n 

Cor. If m = n = 1, then C will be the mid-point, and 

 

mb na 

OC 

a b 

= 

2 

5.7 PRODUCT OF TWO VECTORS 

 

 

The product of two vectors results in two different ways, the one is a number and 

the other is vector. So, there are two types of product of two vectors, namely scalar 

product and vector product. They are written as 

a . 

 

b and a 

 

b . 

5.8 SCALAR, OR DOT PRODUCT 

The scalar, or dot product of two vectors a and b is defined to be 

a 

scalar where is the angle between a and b . 

Symbolically, 

a . 

 

b = a b cos 

 

b cos i.e., 

Due to a dot between a and 

b this product is also called dot product. 

The scalar product is commutative 

To Prove. 

Proof. 

 

 

 

 

a . b = b . a 

 

 

b . a = b a 

cos ( ) 

b 

B 

= 

a b cos 

0 

A 

a 

= a . 

 

b Proved. 

Geometrical interpretation. The scalar product of two vectors is the product of one 

vector and the length of the projection of the other in the direction of the first. 

Let 

then 

 

= 

OA 

 

 

 

 

a and OB b 

a . b = (OA) . (OB) cos 

ON 

= OA . OB . OB 

= OA . ON 

= (Length of a ) (projection of b along a ) 

5.9 USEFUL RESULTS 

^ 

^ 

i . i = (1) (1) cos 0° = 1 Similarly, ^ j . 

^ 

j = 1, 

^ 

^ 

^ 

^ 

k . k = 1 

i . j = (1) (1) cos 90° = 0 Similarly, ^ j . k ^ 

= 0, k . i = 0 

Note. If the dot product of two vectors is zero then vectors are prependicular to each other. 

5.10 WORK DONE AS A SCALAR PRODUCT 

If a constant force F acting on a particle displaces it from A to B then, 

Work done = (component of F along AB). Displacement 

= F cos . AB 

= 

F . AB 

Work done = Force . Displacement 

0 

A 

 

 

^ 

b 

^ 

a 

N 

B 

F 

A 

B

Vectors 375 

5.11 VECTOR PRODUCT OR CROSS PRODUCT 

1. 

 

The vector, or cross product of two vectors a 

and b is defined to be a vector such that 

(i) Its magnitude is 

 

a 

 

b 

sin , where is the 

 

 

 

b 

angle between a and b . 

(ii) Its direction is perpendicular to both vectors 

 

a and b 

 

. 

(iii) It forms with a right handed system. 

Let ^ 

be a unit vector perpendicular to both the vectors a and b . 

 

 

a b = 

2. Useful results 

 

a 

 

b 

 

sin . 

 

Since î , ^j , ^k are three mutually perpendicular unit vectors, then 

^ 

^ 

^ ^ ^ ^ 

i i = j j k k 0 

^ 

^ 

i j = 

^ ^ ^ 

j i k 

^ ^ ^ ^ 

j i i j 

^ ^ ^ 

ĵ k ˆ = k j 

^ ^ ^ ^ 

i and k j j k 

^ ^ ^ 

kˆ 

i ˆ 

^ ^ ^ ^ 

= i k j i k k i 

5.12 VECTOR PRODUCT EXPRESSED AS A DETERMINANT 

If 

 

^ ^ ^ 

a = a i a j a k 

1 2 3 

^ ^ ^ 

b = b1 i b2 j b3 

k 

 

a b = 

= 

^ ^ ^ ^ ^ ^ 

1 2 3 1 2 3 

( a i a j a k) ( b i b j b k) 

^ ^ ^ ^ ^ ^ ^ ^ ^ ^ 

1 1( ) 1 2( ) 1 3( ) 2 1( ) 2 2 ( ) 

^ ^ ^ ^ ^ ^ ^ ^ 

ab 2 3 j k ab 3 1k i ab 3 2 k j ab 3 3 k k 

ab i i ab i j ab i k ab j i ab j j 

^ ^ ^ ^ ^ ^ 

1 2 1 3 2 1 2 3 3 1 3 2 

= ab k a b j ab k a b i ab j a b i 

= 

= 

^ ^ ^ 

2 3 3 2 1 3 3 1 1 2 2 1 

^ ^ ^ 

( ab ab) i ( a b a b ) j ( ab ab) 

k 

i j k 

a a a 

1 2 3 

b b b 

1 2 3 

5.13 AREA OF PARALLELOGRAM 

( ) ( ) ( ) ( ) 

Example 3. Find the area of a parallelogram whose adjacent sides are i – 2j + 3 k and 

2i + j – 4k. 

^ ^ ^ 

i j k 

Solution. Vector area of gm = 1 2 3 

2 1 4 

 

 

a

376 Vectors 

^ ^ ^ 

^ ^ ^ 

= (8 3) i ( 4 6) j (1 4) k = 5i 10 j 5k 

2 2 2 

Area of parallelogram = (5) (10) (5) = 5 6 Ans. 

5.14 MOMENT OF A FORCE 

O 

Let a force F ( PQ ) act at a point P. 

Moment of 

F about O 

= Product of force F and perpendicular 

distance (ON. ^ 

) 

 

= (PQ) (ON)( ^ 

) = (PQ) (OP) sin (^ 

) = 

 

M r F 

5.15 ANGULAR VELOCITY 

 

 

OP PQ 

Let a rigid body be rotating about the axis OA with the angular 

velocity which is a vector and its magnitude is radians per second 

and its direction is parallel to the axis of rotation OA. 

Let P be any point on the body such that OP = r and 

AOP = and AP OA. Let the velocity of P be V. 

Let be a unit vector perpendicular to and r . 

 

r = ( r sin ) ^ = ( AP) = (Speed of P) ^ 

r 

N P F 

A 

Axis 

 

B 

 

V 

r 

Q 

P 

= Velocity of P to and r 

 

Hence V = 

r 

5.16 SCALAR TRIPLE PRODUCT 

Let a, b, 

 

c be three vectors then their dot product is written as a .( b 

c)or[ 

a b c ]. 

If 

 

 

a = 

^ 

 

^ ^ ^ ^ ^ ^ ^ 

^ 

1 2 3 , 1 2 3 , and 1 2 3 

a i a j a k b b i b j b k c c i c j c k 

^ ^ ^ ^ ^ ^ ^ ^ ^ 

1 2 3 1 2 3 1 2 3 

a .( b c ) = ( a i a j a k).[( b i b j b k) ( c i c j c k)] 

= 

^ ^ ^ ^ ^ ^ 

1 2 3 2 3 3 2 3 1 1 3 1 2 

2 1 

( a i a j a k).[( bc bc ) i ( b c b c ) j ( bc bc) k] 

= a 1 

(b 2 

c 3 

– b 3 

c 2 

) + a 2 

(b 3 

c 1 

– b 1 

c 3 

) + a 3 

(b 1 

c 2 

– b 2 

c 1 

) 

= 

a a a 

1 2 3 

b b b 

1 2 3 

c c c 

1 2 3 

Similarly, b .( c 

a)and c .( a 

 

b ) have the same value. 

 

a .( b c ) = b .( c 

 

a ) = c .( a 

 

b ) 

The value of the product depends upon the cyclic order of the vector, but is 

independent of the position of the dot and cross. These may be interchanged. 

The value of the product changes if the order is non-cyclic. 

Note. 

 

a ( b . c) and ( a . b) 

c are meaningless. 

O

Vectors 377 

5.17 GEOMETRICAL INTERPRETATION 

The scalar triple product a .( b 

 

c ) represents the volume of the parallelopiped 

having a , b , c as its co-terminous edges. 

 

a .( b c ) = a .Area of gm OBDC 

= Area of gm OBDC × perpendicular distance 

A 

between the parallel faces OBDC and AEFG. a 

– 

= Volume of the parallelopiped 

Note. (1) If a .( b 

 

c ) = 0, then a, b, 

 

c are 

coplanar. 

1 

(2) Volume of tetrahedron ( ) 

6 a b c . 

Example 4. Find the volume of parallelopiped if 

^ 

^ ^ ^ ^ ^ ^ ^ ^ ^ 

a 3 i 7 j 5k, b 3i 7j 3k, 

and c 7 i 5 j 3k 

are the three co-terminous edges of the parallelopiped. 

Solution. 

Volume = a .( b 

 

c ) 

3 7 5 

= 

3 7 3 

7 5 3 

= 108 – 210 – 170 = – 272 

Volume = 272 cube units. 

= – 3 (–21 – 15) – 7 (9 + 21) + 5 (15 – 49) 

Example 5. Show that the volume of the tetrahedron having 

 

Ans. 

A B, B C, 

C A as 

concurrent edges is twice the volume of the tetrahendron having A , B , C 

as concurrent edges. 

1 

Solution. Volume of tetrahendron = ( ) .[( ) ( )] 

6 A B B C C A 

1 

= ( ) .[ ] 

6 A B B C B A C C C A 

[ C C 0] 

1 

= ( ) .( ) 

6 A B B C B A C A 

1 

= [ .( ) .( ) .( ) .( ) .( ) .( )] 

6 A B C A B A A C A B B C B B A B C A 

1 1 

= [ A.( BC) B.( CA)] A.( B 

C) 

6 3 

 

= 2 1 [ ] 

6 ABC 

= 2 Volume of tetrahedron having A , B , C 

, as concurrent edges. Proved. 

EXERCISE 5.1 

1. Find the volume of the parallelopiped with adjacent sides. 

 

OA = 3 i j, OB j 2 k, and OC i 5 j 4k 

extending from the origin of co-ordinates O. Ans. 20 

2. Find the volume of the tetrahedron whose vertices are the points A (2, –1, –3), B (4, 1, 3) 

C (3, 2, –1) and D (1, 4, 2). 

O 

n^ 

– 

b 

G 

B 

– 

c 

C 

E 

Ans. 

F 

D 

1 

7 3

378 Vectors 

3. Choose y in order that the vectors 

^ ^ ^ ^ ^ 

 

a 7 i yj kˆ 

, b 3 i 2 j k, 

^ ^ ^ 

c 5 i 3 j k are linearly dependent. Ans. y = 4 

4. Prove that 

 

[ a b, b c, c a] 2[ a b c] 

5.18 COPLANARITY QUESTIONS 

Example 6. Find the volume of tetrahedron having vertices 

^ ^ ^ 

^ ^ ^ 

^ ^ ^ ^ ^ 

( j k), ( 4i 5j qk), ( 3i 9j 

4k ) and 4( i j k) 

. 

Also find the value of q for which these four points are coplanar. 

(Nagpur University, Summer 2004, 2003, 2002) 

 

^ ^ ^ ^ ^ ^ ^ ^ ^ ^ ^ 

Solution. Let A = j k, B4 i 5 jqk, C 3 i 9 j4 k, D 4( i j k) 

AB = 

^ ^ ^ ^ ^ ^ ^ 

B A 4 i 5 j qk ( jkˆ 

) 4i 6 j( q 

1) k 

AC = 

^ ^ ^ ^ ^ ^ ^ 

C A (3 i 9ˆj 4 k) ( jk) 3 i 10 j5 

k 

AD = ^ ^ ^ ^ ^ ^ ^ ^ 

D A 4( i jk) ( jk) 4i 5 j 

5k 

Volume of the tetrahedron = 1 [ AB AC AD] 

6 

4 6 q 1 

1 

= 3 10 5 = 1 {4(50 25) 6(15 20) ( q 1)(15 40)} 

6 

6 

4 5 5 

= 1 {100 210 55 ( q 1)} = 1 ( 110 55 55 q) 

6 

6 

= 1 ( 5555 q) 55 ( q1) 

6 6 

If four points A, B, C and D are coplanar, then ( AB AC AD ) = 0 

i.e., Volume of the tetrahedron = 0 

55 

 

( q 1) = 0 q = 1 Ans. 

6 

Example 7. If four points whose position vectors are a, b, c, 

d are coplanar, show that 

 

[ a b c] [ a d b] [ a d c] [ d b c ] (Nagpur University, Summer 2005) 

Solution. Let A, B, C, D be four points whose position vectors are a, b, c, 

 

d . 

 

AD = d a, BD d b and CD d 

 

c 

 

If AD, BD, 

CD are coplanar, then 

 

 

AD .( BD CD ) = 0 

 

( d a) .[( d b) ( d c )] = 0 

 

 

 

( d a) .[ d d d c b d b c ] = 0 

 

( d a) .[ d c b d b c ] = 0 

 

d .( d c) d .( b d) d .( b c) a .( d c) a .( b a) a .( b c ) = 0 

 

0 0 [ dbc] [ ddc] [ dbd] [ abc ] = 0 

 

 

[ abc ] [ abd] [ adc] [ dbc] 

Proved.

Vectors 379 

EXERCISE 5.2 

1. Determine such that 

^ ^ ^ ^ ^ ^ ^ ^ 

a i j k, b 2i 4 k, and c i j 3 k are coplanar. Ans. = 5/3 

2. Show that the four points 

^ ^ ^ ^ ^ ^ ^ ^ ^ ^ ^ ^ 

6 i 3 j 2 k,3i 2 j 4 k,5i 7 j 3 k and 13 i 17 j k are coplanar. 

3. Find the constant a such that the vectors 

^ ^ ^ ^ ^ ^ ^ ^ ^ 

2 i j k, i 2 j 3 k,and 3 i a j 5 k are coplanar. Ans. – 4 

4. Prove that four points 

^ ^ ^ ^ ^ ^ ^ ^ ^ ^ ^ 

4 i 5 j k, ( j k), 3 i 9 j 4 k,4( i j k) 

are coplanar. 

 

5. If the vectors a, b and c are coplanar, show that 

 

a b c 

 

a . a a . b a . c 

 

b . a b . b b . c 

5.19 VECTOR PRODUCT OF THREE VECTORS (A.M.I.E.T.E., Summer, 2004, 2000) 

= 0 

Let a , b and c be three vectors then their vector product is written as a ( b × 

c ). 

Let 

 

^ ^ ^ 

a = a i a j a k 

1 2 3 , 

^ ^ ^ 

b = b1 i b2 j b3 k, 

^ ^ ^ 

c = c1 i c2 j c3 

k 

^ ^ ^ ^ ^ ^ ^ ^ ^ 

( a1 i a2 j a3 k) ( b1 i b2 j b3 k) ( c1 i c2 j c3 

k) 

^ ^ ^ ^ ^ ^ 

= a1 i a2 j a3 k bc 2 3 bc 3 2 i b3c1 b1c3 j bc 1 2 

bc 2 1 k 

a ( b c ) = 

= 

( ) [( ) ( ) ( ) ] 

^ 

2 1 2 2 1 3 3 1 1 3 3 2 3 3 2 1 1 2 

2 1 

[ a ( b c b c ) a ( bc bc )] i [ a ( bc bc ) a ( b c b c )] j 

[ a ( b c b c ) a ( bc bc) k] 

1 3 1 1 3 2 2 3 3 2 

^ ^ ^ ^ ^ ^ 

= ac 1 1 ac 2 2 ac 3 3 b1 i b2 j b3 k a1 b1 ab 2 2 ab 3 3 c1 i c2 j c3 

k 

= 

( )( ) ( )( ) 

( a . c) b ( a . b) c . 

Ans. 

Example 8. Prove that : 

 

a ( b c) b ( c a) c ( a b ) 0 (Nagpur University, Winter 2008) 

Solution. Here, we have 

 

a ( b c) b ( c a) c ( a b ) 

= 

[( a . c) b ( a . b) c] [( b . a) c ( b . c) a] [( c . b) b ( c . a) b] 

= 

[( b . a) c ( a . b) c] [( c . b) a ( b . c) a] [( a . c) b ( c . a) b] 

 

= [( a . b) c ( a . b) c] [( b . c) a ( b . c) a] [( c . a) b ( c . a) b] 

= 0 + 0 + 0 = 0 Proved. 

Example 9. Prove that : 

^ ^ ^ ^ ^ ^ 

i ( a i) j ( a j) k ( a k) 2 a (Nagpur University, Winter 2003) 

^ ^ ^ 

Solution. Let a = a i a j a k 

1 2 3 

^ 

^

380 Vectors 

Now, L.H.S. = 

^ ^ ^ ^ ^ ^ 

i ( a i) j ( a j) k ( a k) 

 

 

= ^ i ( a ^ ^ ^ ^ ^ ^ ^ ^ ^ 

1 i a2 j a3 k) i j ( a1 i a2 j a3 

k) 

j 

= 

 

 

^ ^ ^ ^ ^ 

k ( a1 i a2 j a3 

k) 

k 

^ ^ ^ ^ ^ ^ ^ ^ ^ ^ ^ ^ ^ ^ 

i a1( i i) a2( j i) a3( k i) j a1( i j) a2( j j) a3 

( k 

j) 

 

^ ^ ^ ^ ^ ^ ^ 

k a1( i k) a2( j k) a3( k k) 

 

0 0 0 

 

 

= ^ i a ^ ^ ^ ^ ^ ^ ^ ^ 

2 k a3 j j a1 k a3 i k a1 j a2 

i 

= 

= 

= 

^ ^ ^ ^ ^ ^ ^ ^ ^ ^ ^ ^ 

2 3 1 3 1 2 

a ( i k) a ( i j) a ( j k) a ( j i) a ( k j) a ( k i) 

^ ^ ^ ^ ^ ^ 

2 3 1 3 1 2 

^ ^ ^ 

1 2 3 

a j a k a i a k a i a j = 2a i 2a j 2a k 

^ ^ ^ 

1 2 3 

 

2( a i a j a k) 2 a Proved. 

Example 10. Show that for any scalar , the vectors 

x, 

 

y given by 

 

 

q ( a b) (1 p ) p ( a b ) 

x a , y a 

satisfy the equations 

2 2 

a 

q 

a 

 

px qy aand x y b . (Nagpur University, Winter 2004) 

Solution. The given equations are 

 

 

px qy = a ...(1) 

 

 

x y = b ...(2) 

Multiplying equation (1) vectorially by x , we get 

 

x ( px qy ) = x 

 

a 

 

p ( x x) q ( x y ) = x 

 

a 

 

q ( x y ) = x 

a, 

as x 

x 0 

 

x a = qb , 

[From (2) x 

y 

 

b ] ...(3) 

 

 

Multiplying (3) vectorially by a , we have 

 

a ( x a ) = a q 

b 

 

( a . a) x ( a . x) 

a = 

2 

 

a x ( a . x) 

a = 

 

 

q ( a b ) 

 

 

q ( a b ) 

2 

 

a x = 

 

( a . x) a q ( a b) 

x = 

2 2 

a a 

 

( a . x) a q ( a b)

Vectors 381 

 

x = 

a 

 

 

q ( a b ) 

Substituting the value of x in (1), we get 

 

 

 

q ( a b ) 

qy = a p a 

2 

a 

 

 

 

(1 p) a p ( a b ) 

y = 

2 

q a 

EXERCISE 5.3 

1. Show that a ( b a) ( a b) 

 

a 

2. Write the correct answer 

(a) 

 

A B C lies in the plane of 

( ) 

(i) 

 

A and B (ii) B and 

 

C (iii) 

(b) The value of a .( b c) ( a + b 

 

c ) is 

(i) Zero 

(ii) 

 

[ a , b , c] [ b , c , a ] (iii) 

5.20 SCALAR PRODUCT OF FOUR VECTORS 

Prove the identity 

 

( a b) .( c d ) = ( a . c) ( b . d) ( a . d) ( b . c) 

Proof. 

 

( a b) .( c d ) = 

= 

 

 

( a b) . r 

a 

2 

where = 

 

 

q ( a b ) 

p a qy 

2 

= a 

a 

 

 

 

2 

 

a . x 

a 

Ans. 

 

C and A Ans. (ii) 

 

[ a , b , c ] (iv) None of these 

Ans. (ii) 

a .( b r) 

dot and cross can be interchanged. Put c 

d 

 

r 

= 

 

 

a .[ b ( c d )] = a .[( b . d) c ( b . c) 

d ] 

= 

= 

( a . c) ( b . d) ( a . d) ( b . c) 

 

a . c a . d 

 

b . c b . d 

EXERCISE 5.4 

Proved. 

1. If a 2i 3 j k, b i 2j 4 k, c i j k,find ( a b) .( a 

c). 

Ans. –74 

2. Prove that 

 

2 

 

( a b) .( a c) a ( b . c) ( a . b)( a . c ). 

5.21 VECTOR PRODUCT OF FOUR VECTORS 

Let a, b, 

 

c and d be four vectors then their vector product is written as 

Now, 

 

( a b) ( c d) 

 

( a b) ( c d ) = r ( c 

 

d ) 

[Put a 

b 

 

r ] 

= 

( r . d) c ( r . c) 

d

382 Vectors 

 

= 

 

[( a b). d] c [( a b) . c] 

d 

= 

[ a b d] c [ a b c] 

d 

( a b) ( c d) 

lies in the plane of c and d . ...(1) 

 

Again, ( a b) ( c d ) = ( a 

b) 

 

 

s [Put = c 

d 

 

s ] 

 

 

= s ( a b ) = ( s . b) a ( s . a) 

b 

= 

 

[( c d). b] a [( c d) . a] 

b = 

 

[( b c d] a [ a c d] 

b 

 

( a b) ( c d) 

lies in the plane of a and b . ...(2) 

 

Geometrical interpretation : From (1) and (2) we conclude that ( a b) ( c d ) is 

a vector parallel to the line of intersection of the plane containing a , 

b and plane 

containing c , d . 

Example 11. Show that 

 

( B C) ( A D) ( C A) ( B D) ( A B) ( C D) 2 ( A BC) 

D 

Solution. L.H.S. = 

= 

= 

= 

= 

 

( B C) ( A D) ( C A) ( B D) ( A B) ( C D) 

 

[( BCD) A ( B CA) D] [( C AD) B ( C AB) D] [( B CD) A ( A CD) B] 

 

( BCD) A ( B CD) A ( C AD) B ( A CD) B ( B CA) D ( C AB) 

D 

 

( ACD) B ( ACD) B ( AB C) D ( ABC) 

D 

 

 

2( AB C) 

D = R.H.S. 

Show that: 

 

1. ( b c) ( c a) c ( a b c) 

when 

2. 

3. 

4. 

5. 

6. 

7. 

8. 

EXERCISE 5.5 

 

2 

[ b c, c a, a b)] [ a v c] 

 

d [ a { b ( c d)}] [( b . d)[ a . ( c d)] 

 

2 

 

a [ a [ a ( a b)] a ( b a) 

 

[( a b) ( a c)] . d ( a . d) [ a b c] 

2 2 

2 

2 ^ ^ ^ 

2a a i a j a k 

^ ^ ^ ^ ^ ^ 

a b [( i a). b] i [( j a) . b] j [( k a). b] 

k 

 

( a b c ) stands for scalar triple product. 

 

p [( a q) ( b r)] q [( a r) ( b p)] r [( a p) ( b q )] 0 

Proved.

Vectors 383 

5.22 VECTOR FUNCTION 

If vector r is a function of a scalar variable t, then we write 

 

 

r = r() 

t 

If a particle is moving along a curved path then the position vector 

r of the particle is a 

function of t. If the component of f (t) along x-axis, y-axis, z-axis are f 1 

(t), f 2 

(t), f 3 

(t) respectively. 

Then, 

— 

f() 

t 

5.23 DIFFERENTIATION OF VECTORS 

 

= f1() t i f2() t j f3() 

t k 

Let O be the origin and P be the position of a moving particle at time t. 

— 

Let 

OP = 

r 

Let Q be the position of the particle at the time t + t and 

the position vector of Q is OQ — = r r 

 

— 

 

 

PQ = — 

OQ OP 

— 

 

 

= ( r r) 

r r 

r 

is a vector. As t 0, Q tends to P and the chord 

t 

becomes the tangent at P. 

 

 

dr r 

We define 

= 

lim 

t 

0 

dt t , then 

 

dr 

dt 

 

dr 

dt 

Similarly, 

 

is a vector in the direction of the tangent at P. 

is also called the differential coefficient of r 

d 

2 

 

dt 

2 

r 

is the second order derivative of r . 

r + r 

O 

with respect to ‘t’. 

dr 

d r 

gives the velocity of the particle at P, which is along the tangent to its path. Also 

dt 

2 

dt 

gives the acceleration of the particle at P. 

5.24 FORMULAE OF DIFFERENTIATION 

 

 

(i) d 

( F G) 

d F dG (ii) d 

( F ) 

d F d 

 

F (U.P. I semester, Dec. 2005) 

dt dt dt dt dt dt 

 

 

d 

 

dG d F d 

 

dG d F 

 

(iii) ( FG . ) F. . G(iv) ( F G) 

F G 

dt dt dt dt dt dt 

 

d d a d b d c 

(v) [ abc] 

bc a c ab 

 

dt dt 

dt 

dt 

 

 

d d a d b dc 

(vi) [ a ( b c)] ( b c) 

a c a b 

 

dt dt dt dt 

The order of the functions 

F, 

G 

is not to be changed. 

 

 

 

Q 

r 

r 

Tangent 

P (r) 

2

384 Vectors 

Example 12. A particle moves along the curve 

 

3 2 2 3 

r ( t 4 t) i ( t 4) t j (8t 3 t ) k , 

where t is the time. Find the magnitude of the tangential components of its acceleration 

at t = 2. 

(Nagpur University, Summer 2005) 

Solution. We have, r = 

Velocity = 

 

3 2 2 3 

( t 4 t) i ( t 4) t j (8t 3 t ) k 

 

dr 

 

2 2 

(3t 4) i (2t 4) j (16t 9 t ) k 

dt 

 

At t = 2, Velocity = 8i 8 j 4 

k 

2 

Acceleration = d r 

a = 6ti 2 j (1618) 

t k 

2 

dt 

 

At t = 2 a 12 i 2 j 20 k 

The direction of velocity is along tangent. 

So the tangent vector is velocity. 

Unit tangent vector, 

Tangential component of acceleration, a t 

= aT . 

 

 

 

 

 

v 8i 8 j 4k 8i 8 j 4k 2i 2 j k 

T = 

| v | 64 64 16 

12 3 

 

2i 2 j k 

24 4 

20 48 

= (12i 2 j 20 k). 

= = 16 Ans. 

3 

3 3 

 

 

Example 13. If da u a and db d 

u b then prove that [ a b ] u ( a b ) 

dt 

dt 

dt 

(M.U. 2009) 

Solution. We have, 

d 

[ a b ] = 

dt 

 

 

db da 

 

a b = a ( u b) ( u a) 

b 

dt dt 

 

= a ( u b) b ( u a) 

 

= ( a. b) u ( a. u) b [( b. a) u ( b. u) a] 

(Vector triple product) 

 

= ( a. b) u ( u. a) b ( a. b) u ( u. b) 

a 

 

= ( u. b) a ( u. a) 

b 

 

= u ( a b ) 

Proved. 

Example 14. Find the angle between the surface x 2 + y 2 + z 2 = 9 and z = x 2 + y 2 – 3 at 

(2, –1, 2). (M.D.U. Dec. 2009) 


x 2 + y 2 + z 2 = 9 ...(1) 

z = x 2 + y 2 – 3 ...(2) 

Normal to (1) 1 

= (x 2 + y 2 + z 2 – 9) 

= 

 

 

 

ˆ 

 

x y z 

ˆ ˆ 2 2 2 

i j k ( x y z – 9) 

= 2 x î + 2 y ĵ + 2 z ˆk 

Normal to (1) at (2, – 1, 2), 1 

= 4î – 2 ĵ + 4 ˆk ...(3)

Vectors 385 

Normal to (2), 2 

= (z – x 2 – y 2 + 3) 

= 

 

 

 

ˆ 

 

x y z 

ˆ ˆ 2 2 

i j k ( z– x – y 3) 

= – 2 x î – 2 y ĵ + ˆk 

Normal to (2) at (2, – 1, 2), 2 

= – 4î + 2 ĵ + ˆk ...(4) 

1. 

2 = | 1|| 2|cos 

 

1. 

2 

(4 iˆ –2ˆj 4 kˆ).(– 4iˆ 2 ˆj kˆ) –16 –44 

cos = = 

= 

| 1|| 2| 

|4 iˆ –2ˆj 4 kˆ||– 4iˆ 2 ˆj kˆ| 

16 416 16 41 

= –16 –8 

= 

6 21 3 21 

–1 –8 

= cos 

3 21 

–1 –8 

Hence the angle between (1) and (2) cos 

Ans 

3 21 

EXERCISE 5.6 

2 

3 

t 

t 

1. The coordinates of a moving particle are given by x = 4t and y = 3 6 t . Find the 

2 

6 

velocity and acceleration of the particle when t = 2 secs. Ans. 4.47, 2.24 

2. A particle moves along the curve 

x = 2t 2 , y = t 2 – 4t and z = 3t – 5 

where t is the time. Find the components of its velocity and acceleration at time t = 1, in the 

 

8 14 14 

direction i 3 j 2 k. 

(Nagpur, Summer 2001) Ans. , 

7 7 

3. Find the unit tangent and unit normal vector at t = 2 on the curve x = t 2 – 1, y = 4t – 3, 

z = 2t 2 – 6t where t is any variable. 

Ans. 

1 1 

(2 i 2 j k), (2i 2 k) 

3 3 5 

 

d 

 

dG d F 

4. Prove that ( F G) 

F G 

dt dt dt 

 

5. Find the angle between the tangents to the curve 2 3 

r t i 2 t j t k, 

at the points t = ± 1. 

Ans. 1 9 

cos 

 

 

 

17 

 

6. If the surface 5x 2 – 2byz = 9x be orthogonal to the surface 4x 2 y + z 3 = 4 at the point (1, –1, 2) 

then b is equal to 

(a) 0 (b) 1 (c) 2 (d) 3 (AMIETE, Dec. 2009) Ans. (b) 

5.25 SCALAR AND VECTOR POINT FUNCTIONS 

Point function. A variable quantity whose value at any point 

N 

^ 

in a region of space depends upon the position of the point, is 

R 

Q 

called a point function. There are two types of point functions. 

n r 

(i) Scalar point function. If to each point P (x, y, z) of a 

region R in space there corresponds a unique scalar f (P), then f is 

P 

called a scalar point function. For example, the temperature 

distribution in a heated body, density of a body and potential due to gravity are the examples of 

a scalar point function. 

(ii) Vector point function. If to each point P (x, y, z) of a region R in space there corresponds 

a unique vector f (P), then f is called a vector point function. The velocity of a moving fluid, 

gravitational force are the examples of vector point function. 

(U.P., I Semester, Winter 2000) 

= c 

 

+ d = c

386 Vectors 

Vector Differential Operator Del i.e. 

The vector differential operator Del is denoted by . It is defined as 

 

= i j k 

x y z 

5.26 GRADIENT OF A SCALAR FUNCTION 

 

If (x, y, z) be a scalar function then i j k is called the gradient of the scalar 

x y z 

function . 

And is denoted by grad . 

 

Thus, grad = i j k 

x y z 

 

gard = i j k ( x, y, z) 

x y z 

gard = 

5.27 GEOMETRICAL MEANING OF GRADIENT, NORMAL 

( is read del or nebla) 

(U.P. Ist Semester, Dec 2006) 

If a surface (x, y, z) = c passes through a point P. The value of the function at each point 

on the surface is the same as at P. Then such a surface is called a level surface through P. For 

example, If (x, y, z) represents potential at the point P, then equipotential surface (x, y, z) = c 

is a level surface. 

Two level surfaces can not intersect. 

Let the level surface pass through the point P at which the value of the function is . Consider 

another level surface passing through Q, where the value of the function is + d. 

Let r and r r be the position vector of P and Q then PQ 

— 

r 

.dr = 

 

i j k 

.( idx jdy kdz 

 

) 

x y z 

= 

 

dx dy dz d 

x y z 

If Q lies on the level surface of P, then d = 0 

Equation (1) becomes . dr = 0. Then is to dr (tangent). 

Hence, is normal to the surface (x, y, z) = c 

Let = || N , where N is a unit normal vector. Let n be the perpendicular distance 

between two level surfaces through P and R. Then the rate of change of in the direction of the 

normal to the surface through P is 

 

. 

n 

...(1) 

d = lim lim 

dn n 

0 n 

n 

0 

= 

= 

| | Ndr . 

lim 

n 

n 0 

n 

0 

 

 

. 

dr 

n 

 

| | n 

lim | | 

n 

 

 

N . r | N || r 

|cos 

 

 

 

| r|cos 

n

Vectors 387 

 

|| = 

n 

Hence, gradient is a vector normal to the surface = c and has a magnitude equal to the 

rate of change of along this normal. 

5.28 NORMAL AND DIRECTIONAL DERIVATIVE 

(i) Normal. If (x, y, z) = c represents a family of surfaces for different values of the constant 

c. On differentiating , we get d = 0 

But d = . 

 

dr so . d r = 0 

The scalar product of two vectors and dr being zero, and 

each other. dr 

is in the direction of tangent to the given surface. 

Thus is a vector normal to the surface (x, y, z) = c. 

 

 

dr are perpendicular to 

(ii) Directional derivative. The component of in the direction of a vector d is equal to 

.d 

and is called the directional derivative of in the direction of d . 

 

r 

 

r 

lim 

= 

r 

0 

 

r 

where, r = PQ 

is called the directional derivative of at P in the direction of PQ. 

Let a unit vector along PQ be N . 

Now 

n 

r 

 

r 

n 

n 

= cos r = cos 

 

N. 

N 

 

lim N. 

N 

 

= r 

0 

 

 

n 

n 

 

NN 

. 

 

 

 

 

 

...(1) 

 

n 

 

From (1), r 

 

 

N. 

N 

 

 

 

 

 

= N . N | | = N . ( N | | ) 

 

Hence, 

r 

, directional derivative is the component of in the direction N . 

 

= N . | |cos | | 

r 

Hence, is the maximum rate of change of . 

Example 15. For the vector field (i) 

 

A miˆ 

and (ii) A m r . Find . 

A and 

A . 

Draw the sketch in each case. (Gujarat, I Semester, Jan. 2009) 

 

 

Solution. (i) Vector A mi is represented in the figure (i). 

(ii) A = mr is represented in the figure (ii). 

(iii) 

. 

 

 

A = i j k .( xi y j zk) 1113 

x y z 

. 

A = 3 is represented on the number line at 3. 

(iv) 

 

A = i j k ( xi y j zk) 

x y z

388 Vectors 

 

i j k 

= 

 

x y z 

x y z 

are represented in the adjoining figure. 

^ 

i 

^ 

k 

^ 

m . i 

k 

r 

 

j 

O 

O 

Number line 

j 0 1 2 3 O 

i 

i 

(i) (ii) (iii) (iv) 

m r 

Example 16. If = 3x 2 y – y 3 z 2 ; find grad at the point (1, –2, –1). 

(AMIETE, June 2009, U.P., I Semester, Dec. 2006) 

Solution. grad = 

2 3 2 

= i j k (3 x y y z ) 

x y z 

 

2 3 2 

2 3 2 2 3 2 

= i (3 x y y z ) j (3 x y yz) k (3 x y y z ) 

x y z 

= 

= 0 

 

2 2 2 3 

i (6 xy) j (3x 3 y z ) k ( 2 yz) 

 

grad at (1, –2, –1) = i (6) (1) ( 2) j[(3) (1) 3(4) (1)] k ( 2)( 8) ( 1) 

 

= 12 i 9 j 16 k 

Ans. 

Example 17. If u = x + y + z, v = x 2 + y 2 + z 2 , w = yz + zx + xy prove that grad u, 

grad v and grad w are coplanar vectors. [U.P., I Semester, 2001] 


grad u = 

 

 

i j k ( x y z) 

i j k 

x y z 

grad v = 

 

 

2 2 2 

i j k ( x y z ) 2xi 2yj 

2zk 

x y z 

grad w = 

 

 

i j k ( yz zx xy) i( z y) jz ( x) k( y x) 

x y z 

[For vectors to be coplanar, their scalar triple product is 0] 

Now, grad u.(grad v × grad w) = 

= 

= 

1 1 1 1 1 1 

2x 2y 2z 2 x y z 

z y z x y x z y z x y x 

1 1 1 

2 x y z x y z x y z 

z y z x y x 

1 1 1 

2( x y z) 1 1 1 0 

y z z x x y 

k 

[Applying R 2 

R 2 

+ R 3 

] 

j

Vectors 389 

Since the scalar product of grad u, grad v and grad w are zero, hence these vectors are 

coplanar vectors. 

Proved. 

Example 18. Find the directional derivative of x 2 y 2 z 2 at the point (1, 1, –1) in the direction 

of the tangent to the curve x = e t , y = sin 2t + 1, z = 1 – cos t at t = 0. 


Solution. Let = x 2 y 2 z 2 

Directional Derivative of 

2 2 2 

= = i j k ( x y z ) 

x y z 

 

2 2 2 2 2 2 

= 2xy z i 2yx z j 2zx y k 

Directional Derivative of at (1, 1, –1) 

Tangent vector, 

= 

Tangent(at t = 0) = 

 

2 2 2 2 2 2 

2(1)(1) ( 1) i 2(1)(1) ( 1) j 2( 1)(1) (1) k 

 

= 2 i 2 j 2k 

...(1) 

t 

 

 

r 

= xi yj zk e i (sin 2t 1) j (1 

cos t) 

k 

 

T dr 

t 

= e i 2cos 2tj 

sin tk 

dt 

 

0 

e i 2(cos 0) j (sin 0) k i 2 j 

...(2) 

( i 2 j) 

Required directional derivative along tangent = (2 i 2 j 2 k) 

1 

4 

[From (1), (2)] 

= 2 4 0 6 Ans. 

5 5 

Example 19. Find the unit normal to the surface xy 3 z 2 = 4 at (–1, –1, 2). (M.U. 2008) 

Solution. Let (x, y, z) = xy 3 z 2 = 4 

We know that is the vector normal to the surface (x, y, z) = c. 

 

Normal vector = = i 

x j 

y k 

 

 

z 

Now = 

Normal vector = 

 

i ( xyz) j ( xyz) k ( xyz) 

x y z 

 

3 2 3 2 3 2 

 

3 2 2 2 3 

yz i 3xy z j 2xyzk 

 

Normal vector at (–1, –1, 2) = 4i 12 j 4k 

Unit vector normal to the surface at (–1, –1, 2). 

 

4i 12 j 4k 

1 

= ( i 3 j k ) Ans. 

| | 16 144 16 11 

Example 20. Find the rate of change of = xyz in the direction normal to the surface 

x 2 y + y 2 x + yz 2 = 3 at the point (1, 1, 1). (Nagpur University, Summer 2001) 

Solution. Rate of change of = 

 

 

= i j k ( x yz) 

iyz jxz kxy 

x y z

390 Vectors 

 

Rate of change of at (1, 1, 1) = ( i j k) 

Normal to the surface = x 2 y + y 2 x + yz 2 – 3 is given as - 

= 

= 

() (1, 1, 1) 

= 3i 4 j 2 k 

2 2 2 

i j k ( x y y x yz 3) 

x y z 

 

2 2 2 

i(2 xy y ) jx ( 2 xy z ) k2yz 

 

 

Unit normal = 3 i 4 j 2 k 

916 

4 

 

Required rate of change of = 

(3 i 4 j 2 k) 

( i j k). 

= 3 4 2 9 

916 

4 29 29 

Ans. 

Example 21. Find the constants m and n such that the surface m x 2 – 2nyz = (m + 4)x will 

be orthogonal to the surface 4x 2 y + z 3 = 4 at the point (1, –1, 2). 

(M.D.U. Dec. 2009, Nagpur University, Summer 2002) 

Solution. The point P (1, –1, 2) lies on both surfaces. As this point lies in 

mx 2 – 2nyz = (m + 4)x, so we have 

m – 2n (–2) = (m + 4) 

m + 4n = m + 4 n = 1 

Let 1 

= mx 2 – 2yz – (m + 4)x and 2 

= 4x 2 y + z 3 – 4 

Normal to 1 

= 1 

= 

2 

i j k [ mx 2 yz ( m 

4) x] 

x y z 

 

= i(2mx m 4) 2zj2yk 

Normal to 1 

at (1, –1, 2) = 

 

 

i(2m m 4) 4 j 2k 

= ( m 4) i 4 j 2 k 

Normal to 2 

= 2 

= 

2 3 

 

i j k (4x y z 4) 

2 2 

= i8xy 4x j 3z k 

x y z 

 

Normal to 2 

at (1, –1, 2) = – 8i 4 j 12 

k 

Sinec 1 

and 2 

are orthogonal, then normals are perpendicular to each other. 

1 

. 2 

= 0 

 

[( m 4) i 4 j 2 k].[ 8i 4 j 12 k] 

= 0 

– 8 (m – 4) – 16 + 24 = 0 

m – 4 = –2 + 3 m = 5 

Hence m = 5, n = 1 

Ans. 

Example 22. Find the values of constants and so that the surfaces x 2 – yz = (+ 2) x, 

4x 2 y + z 3 = 4 intersect orthogonally at the point (1, – 1, 2). 

(AMIETE, II Sem., Dec. 2010, June 2009) 


x 2 – yz = ( + 2) x ...(1) 

4x 2 y + z 3 = 4 ...(2)

Vectors 391 

 

Normal to the surface (1), λ x yz(λ2) 

x 

 

 

 

ˆ 

 

ˆ 

ˆ 2 

i j k x yz ( 2) x 

 

x y z 

 

iˆ(2x2) ˆj ( z) kˆ 

( y) 

Normal at (1, –1, 2) = î (2 – – 2) – ĵ (–2) + ˆk ...(3) 

Normal at the surface (2) 

= î ( – 2) + ĵ z (2) + ˆk 

 

ˆ 

 

ˆ 

ˆ 2 3 

i j k (4x yz 

4) 

x y z 

= î (8 × y) + ĵ (4x2 ) + ˆk (3z 2 ) 

Normal at the point (1, –1, 2) = – 8 î + 4 ĵ + 12 ˆk ...(4) 

Since (3) and (4) are orthogonal so 

iˆ( 2) ˆj(2 ) kˆ. 8iˆ4ˆj12kˆ 

0 

 

8( 2) 4(2 ) 12 0 8 168120 

8 20160 

4( 2 54) 0 

2 540 

25 4 

...(5) 

Point (1, – 1, 2) will satisfy (1) 

 

2 

(1) ( 1)(2) ( 2)(1) + 2 = + 2 = 1 

Putting = 1 in (5), we get 

9 

25 4 

2 

9 

Hence and =1 

Ans. 

2 

Example 23. Find the angle between the surfaces x 2 + y 2 + z 2 = 9 and z = x 2 + y 2 – 3 at 

the point (2, –1, 2). (Nagpur University, Summer 2002) 

Solution. Normal on the surface (x 2 + y 2 + z 2 – 9 = 0) 

= 

 

 

2 2 2 

i j k ( x y z 9) (2xi 2yj 

2 zk) 

x y z 

 

Normal at the point (2, –1, 2) = 4i 2 j 4 k 

...(1) 

Normal on the surface (z = x 2 + y 2 2 2 

– 3) = i j k ( x y z 3) 

x y z 

 

= 2xi 2yj 

k 

 

Normal at the point (2, –1, 2) = 4i 2 

j k 

...(2) 

Let be the angle between normals (1) and (2). 

 

(4 i 2 j 4 k).(4 i 2 j k) 

= 16 416 16 41cos 

 

16 + 4 – 4 = 6 21cos 16 = 6 21cos

392 Vectors 

cos = 

8 

3 21 

= 

cos 

1 8 

3 21 

Example 24. Find the directional derivative of 1 r in the direction r where r xi y j zk . 

Solution. Here, (x, y, z) = 

Now 

= 

1 

 

 

= 

r 

 

= 

Ans. 

 

(Nagpur University, Summer 2004, U.P., I Semester, Winter 2005, 2002) 

1 

 

2 2 2 2 

1 1 

( x y z ) 

r 2 2 2 

x y z 

1 

 

 

2 2 2 2 

 

i j k ( x y z ) 

x y z 

 

 

 

1 1 1 

 

2 2 2 2 2 2 2 2 2 2 2 2 

 

( x y z ) i ( x y z ) j ( x y z ) k 

x y z 

 

3 3 

1 

 

2 2 2 1 

 

 

3 

2 2 2 2 

1 

( x y z ) 2 xi ( x y z ) 

2 

2y 

j ( 

2 2 2 ) 

2 2 

2 2 

2 x y z 

z 

 

 

 

k 

 

( xi yj 

zk) 

= 2 2 2 3/2 

( x y z ) 

 

and r = unit vector in the direction of xi yj 

zk 

 

xi yj 

zk 

= 

2 2 2 

x y z 

So, the required directional derivative 

= 

 

2 2 2 

xi yj zk xi yj zk x y z 

. r . 

 

( x y z ) ( x y z ) ( x y z ) 

1 

1 

x y z r 

= 

2 2 2 2 

2 2 2 3/2 2 2 2 1/2 2 2 2 2 

[From (1), (2)] 

Example 25. Find the direction in which the directional derivative of (x, y) = 

x 

2 2 

y 

xy 

...(1) 

...(2) 

(1, 1) is zero and hence find out component of velocity of the vector 3 2 

r ( t 1) 

i t j in 

the same direction at t = 1. (Nagpur University, Winter 2000) 

2 2 

x y 

Solution. Directional derivative = = i j k 

x y z xy 

 

2 2 2 2 

= 

xy .2 x ( x y ) y xy .2 y x ( y x 

i 

j 

) 

2 2 2 2 

x y x y 

2 3 2 3 

= 

x y y i 

j 

xy x 

2 2 2 2 

x y x y 

 

 

Directional Derivative at (1, 1) = i 0 j 0 0 

Since () (1, 1) 

= 0, the directional derivative of at (1, 1) is zero in any direction. 

Again r = 

 

3 2 

( t 1) 

i t 

j 

 

Ans. 

 

at

Vectors 393 

Velocity, v = 

dr 

2 

3t i 2tj 

dt 

 

 

Velocity at t = 1 is = 3i 

2 j 

The component of velocity in the same direction of velocity 

 

3i 

2 j 

9 

4 

= 

(3 i 2 j). 13 

9 

4 13 

Ans. 

 

Example 26. Find the directional derivative of (x, y, z) = x 2 y z + 4 x z 2 at (1, –2, 1) in 

 

the direction of 2i j 2 k . Find the greatest rate of increase of . 

(Uttarakhand, I Semester, Dec. 2006) 

Solution. Here, (x, y, z) = x 2 y z + 4xz 2 

Now, = 

= 

at (1, – 2, 1) = 

2 2 

i j k ( x yz 4 xz ) 

x y z 

 

2 2 2 

(2xyz 4 z ) i ( x z) j ( x y 8 xz) 

k 

2 

 

{2(1) ( 2)(1) 4(1) } i (11) j {1( 2) 8(1)(1)} k 

 

= ( 4 4) i j ( 2 8) k = j 6 k 

 

Let a = unit vector = 2 i j 2 k 1 

(2 i j 2 k) 

41 

4 3 

So, the required directional derivative at (1, –2, 1) 

1 

= . a ( j 6 k). (2 i j 2 k) 

= 1 

( 112) 

 

13 

3 

3 3 

Greatest rate of increase of = j 6k 

= 1 

36 

 

 

= 37 Ans. 

Example 27. Find the directional derivative of the function = x 2 – y 2 + 2z 2 at the point P 

(1, 2, 3) in the direction of the line PQ where Q is the point (5, 0, 4). 

(AMIETE, Dec. 20010, Nagpur University, Summer 2008, U.P., I Sem., Winter 2000) 

Solution. Directional derivative = 

= 

 

i j k ( x y 2 z ) 2xi 2yj 

4zk 

x y z 

 

2 2 2 

Directional Derivative at the point P (1, 2, 3) = 2i 4 j 12 k 

...(1) 

 

 

PQ = Q P = (5, 0, 4) – (1, 2, 3) = (4, –2, 1) ...(2) 

 

(4 i 2 j k) 

Directional Derivative along PQ = (2 i 4 j 12 k). 

[From (1) and (2)] 

16 41 

= 

8812 28 

 

21 21 

Ans. 

x 

Example 28. For the function (x, y) = 2 2 , find the magnitude of the directional 

x y 

derivative along a line making an angle 30° with the positive x-axis at (0, 2). 

(A.M.I.E.T.E., Winter 2002)

394 Vectors 

Solution. Directional derivative = 

 

x 

x y z 

x y 

 

= i j k 2 2 

 

2 2 

y x 2xy 

= i j 

2 2 2 2 2 2 

( x y ) ( x y ) 

Directional derivative at the point (0, 2) 

4 

0 2(0) (2) i 

 

(0 4) (0 4) 4 

 

 

= i j 

2 2 

 

 

1 x(2 x) x(2 y) 

 

 

= i 

 

j 

2 2 2 2 2 

2 2 2 

x y ( x y ) ( x y ) 

Directional derivative at the point (0, 2) in the direction CA — 3 1 

i.e. i j 

2 2 

 

 

 

CA OB BA i cos 30 j sin 30 

i 3 1 

 

 

= . i j 

 

3 1 

 

4 2 2 

 

 

i j 

 

 

2 2 

3 

= 

Ans. 

8 

 

Example 29. Find the directional derivative of V where 2 2 2 

V xy i zy j xz k, at the 

point (2, 0, 3) in the direction of the outward normal to the sphere x 2 + y 2 + z 2 = 14 at the 

point (3, 2, 1). (A.M.I.E.T.E., Dec. 2007) 

 

Solution. V 2 = VV . 

 

2 2 2 2 2 2 

2 

, 

 

= ( xy i zy j xz k).( x y i zy j xz k) 

= x 2 y 4 + z 2 y 4 + x 2 z 4 

Directional derivative = 2 

V 

= 

2 4 2 4 2 4 

i j k ( x y z y x z ) 

x y z 

= 

 

4 4 2 3 3 2 4 2 3 

(2xy 2 xz ) i (4x y 4 y z ) j (2y z 4 xz) 

k 

 

Directional derivative at (2, 0, 3) = (0 2281) i (0 0) j (0 4427) 

k 

 

= 324 i 432k 108 (3 i 4 k) 

...(1) 

Normal to x 2 + y 2 + z 2 – 14 = 

2 2 2 

= i j k ( x y z 14) 

x y z 

 

= (2xi 2yj 

2 zk) 

 

Normal vector at (3, 2, 1) = 6i 4 j 2 k 

...(2) 

Unit normal vector = 6 4 2 2(3 2 ) 3 

i j k i j k i 2 

 

j k 

 

 

36 16 4 2 14 14 

 

3i 2 j k 

Directional derivative along the normal = 108(3 i 4 k). . 

14 

108 (9 4) 1404 

= 

14 14 

j 

—2 

1 

(0, 2) 30° 

i 

C —2 

3 

i 

1 

A 

[From (1), (2)] 

j 

Ans.

Vectors 395 

Example 30. Find the directional derivative of ( f) at the point (1, – 2, 1) in the direction 

of the normal to the surface xy 2 z = 3x + z 2 , where f = 2x 3 y 2 z 4 . (U.P., I Semester, Dec 2008) 


f = 2x 3 y 2 z 4 

 

3 2 4 

f = i j k 

(2 x y z ) 2 2 4 3 4 

 

x y z 

= 3 2 3 

6 x y z i 4 xyz j 8x y z k 

 

 

 

2 2 4 3 4 3 2 3 

(f) = i j k (6x y z 

 

i 4x yz j 8 x y z k) 

x y z 

 

 

 

= 12xy 2 z 4 + 4x 3 z 4 + 24x 3 y 2 z 2 

Directional derivative of ( f ) 

 

2 4 3 4 3 2 2 

= i j k (12xy z 4xz 24 x y z ) 

x y z 

 

 

 

2 4 2 4 2 2 2 

= (12y z 12x z 72 x y z ) i (24xyz4 

48x3yz2) 

j 

+ (48xy 2 z 3 + 16x 3 z 3 + 48x 3 y 2 z) k 

Directional derivative at (1, – 2, 1) = (48 + 12 + 288) i + (– 48 – 96) j + (192 + 16 + 192) k 

= 348 i 

– 144 j 400k 

Normal to(xy 2 z – 3x – z 2 ) = (xy 2 z – 3x – z 2 ) 

 

2 2 

= i j k 

( xy z –3 x – z ) 

x y z 

 

 

 

2 

= 2 

( y z – 3) i (2 xyz) j ( xy – 2 z) 

k 

Normal at(1, – 2, 1) = i 

– 4 j 2k 

i –4j 2k 

Unit Normal Vector = 

= 1 ( – 4 2 ) 

116 

4 21 i j k 

Directional derivative in the direction of normal 

1 

= (348 i –144 j 400 k) ( i –4j 2 k) 

21 

1 

= (348 576 800) = 1724 

Ans. 

21 

21 

Example 31. If the directional derivative of = a x 2 y + b y 2 z + c z 2 x at the point 

x 1 y 3 

z 

(1, 1, 1) has maximum magnitude 15 in the direction parallel to the line , 

2 2 1 

find the values of a, b and c. (U.P. I semester, Winter 2001) 

Solution. Given = a x 2 y + b y 2 z + c z 2 x 

 

= i j k 

x y z (a x2 y + b y 2 z + c z 2 x) 

= 

 

2 2 2 

i(2 axy cz ) jax ( 2 byz) kby ( 2 czx) 

 

at the point (1, 1, 1) = i(2 a c) j( a 2 b) kb ( 2 c) 

...(1) 

We know that the maximum value of the directional derivative is in the direction of . 

i.e. || = 15 (2a + c) 2 + (2b + a) 2 + (2c + b) 2 = (15) 2 

But, the directional derivative is given to be maximum parallel to the line

396 Vectors 

x 1 y 3 z 

= 

 

i.e., parallel to the vector 

2 2 1 

2i 2 j k . 

On comparing the coefficients of (1) and (2) 

...(2) 

 

2a 

c 

= 2 b a 2 c b 

 

2 2 1 

2a + c = – 2b – a 3a + 2b + c = 0 ...(3) 

and 2b + a = – 2(2c + b) 

2b + a = – 4c – 2b a + 4b + 4c = 0 ...(4) 

Rewriting (3) and (4), we have 

3a 2b c 

0 

 

 

b 

c 

a 4b 4c 

0 

4 11 10 

= k (say) 

a = 4k, b = –11k and c = 10k. 

Now, we have 

(2a + c) 2 + (2b + a) 2 + (2c + b) 2 = (15) 2 

(8k + 10k) 2 + (–22k + 4k) 2 + (20k – 11k) 2 = (15) 2 

k = 

a = 

 

5 

 

9 

20 

, b = 

9 

Example 32. If r xi y j zk, 

show that : 

 

r 

1 

(i) grad r = 

 

r 

r 

 

Solution. (i) r = xi yj zk r = 

r 

2r 

x 

r 

Similarly, 

y 

= 2x 

= y r 

grad r = r = 

and 

r 

(ii) grad . 

3 

r 

r 

x 

x 

r 

r 

z 

z 

r 

55 

and c = 

9 

 

50 

 

9 

Ans. 


2 2 2 

x y z r 2 = x 2 + y 2 + z 2 

r r r 

i j k r i j k 

x y z 

x y z 

 

x y z xi yj 

zk r 

= i j k Proved. 

r r r r r 

1 

 

(ii) grad 

r 

= 1 1 

 

1 1 1 

i j k = i 

j k 

r x y zr 

xr yr zr 

 

= 1 r 1 r 1 r 

 

i j k 

2 2 

2 

r x r y 

r z 

 

= 

 

1 x 1 y 1 z 

i j k 

2 2 

2 

r r r r r r = xi yj 

zk r 

Proved. 

3 3 

r r 

2 2 

Example 33. Prove that f () r f ´´ () r f´( r) 

. (K. University, Dec. 2008) 

r 

Solution.

Vectors 397 

f () r 

 

i j k f () r 

x y z 

 

2 2 2 2 r r x r y r z 

r x y z 2r 2 x , and 

 

x x r y r z r 

r r r 

x y z 

if´( r) j f´() r k f´( r) 

f´( r) 

i j k 

x y z 

 

r r r 

 

 

 

xi 

yj 

zk 

f´( r) r 

2 

f () r [ f ( r)] 

 

xi yj zk 

i j k f´( r) x y z 

r 

 

 

 

x y z 

f´( r) 

f´() r 

f´( r) 

x 

r 

 

y 

r 

 

z 

r 

 

 

r 

r 

r1 

x 

r.1 

y 

r x r 

f´´( r) f´( r) x 

y 

y 

f´´( r) 

2 f´( r) 

 

xr r y 

r 

2 

 

r 

z 

r.1 

z 

r z f´´( r) f´( r) 

r 

2 

z r 

 

r 

2 

2 

2 

x 

y 

z 

r 

r 

r 

= x x f´´( r) f´( r) 

r y y 

 

f´´( r) f´( r) 

r z z 

 

2 

´´ () ´( ) 

r 

2 f r 

f r 

2 

r r r r r r r r 

r 

2 2 2 2 

2 2 

x x r x y 

r y z z 

r z 

= f´´( r) f´( r) f´´( r) f´() 

r 

3 

 

3 f´´ () r 

f´( r) 

r r r r 

3 

r r r r 

2 2 2 2 2 2 2 2 2 

x y z y x z z x y 

f´´( r) f´( r) f´´( r) f´() r f´´( r) f´() 

r 

2 3 2 3 2 3 

r r r r r r 

 

2 2 2 2 2 2 2 2 2 

x y z y z z x x y 

= f´´( r) f´( r) 

2 2 2 

3 3 3 

r r r r r r 

2 2 2 2 2 2 

2 2 

x y x 2( x y z 

) r 2r 

f´´ () r f´( r) 

2 3 

= f´´( r) f´( r) 

2 3 

r 

r 

r r 

2 

= f´´() r f´( r) 

r 

Ans. 

EXERCISE 5.7 

1. Evaluate grad if = log (x 2 + y 2 + z 2 ) Ans. 

 

2( xi yj 

zk) 

2 2 2 

x y z 

2. Find a unit normal vector to the surface x 2 + y 2 + z 2 1 

= 5 at the point (0, 1, 2). Ans. ( ˆ 2 ˆ) 

5 j k 

(AMIETE, June 2010) 

3. Calculate the directional derivative of the function (x, y, z) = xy 2 + yz 3 at the point 

5 

(1, –1, 1) in the direction of (3, 1, –1) (A.M.I.E.T.E. Winter 2009, 2000) Ans. 

11 

4. Find the direction in which the directional derivative of f (x, y) = (x 2 – y 2 )/xy at (1, 1) is zero. 

(Nagpur Winter 2000) 

 

i j 

Ans. 

2

398 Vectors 

5. Find the directional derivative of the scalar function of (x, y, z) = xyz in the direction of the outer 

normal to the surface z = xy at the point (3, 1, 3). 

Ans. 

27 

11 

6. The temperature of the points in space is given by T(x, y, z) = x 2 + y 2 – z. A mosquito located at 

(1, 1, 2) desires to fly in such a direction that it will get warm as soon as possible. In what direction 

should it move? 

Ans. 

1 (2 i 

2 j 

 

k ) 

3 

7. If (x, y, z) = 3xz 2 y – y 3 z 2 , find grad at the point (1, –2, –1) Ans. 

 

(16i 9j 4 k) 

8. Find a unit vector normal to the surface x 2 y + 2xz = 4 at the point (2, –2, 3). 

1 

Ans. ( i 2 j 2 k 

 

) 

3 

9. What is the greatest rate of increase of the function u = xyz 2 at the point (1, 0, 3)? Ans. 9 

10. If is the acute angle between the surfaces xyz 2 = 3x + z 2 and 3x 2 – y 2 + 2z = 1 at the point 

(1, –2, 1) show that cos = 3/7 6 . 

11. Find the values of constants a, b, c so that the maximum value of the directional directive of 

= axy 2 + byz + cz 2 x 3 at (1, 2, –1) has a maximum magnitude 64 in the direction parallel to the 

axis of z. Ans. a = b, b = 24, c = –8 

12. Find the values of and µ so that surfaces x 2 – µ y z = ( + 2)x and 4 x 2 y + z 3 = 4 intersect 

orthogonally at the point (1, –1, 2). Ans. = 9 , 1 

2 

13. The position vector of a particle at time t is R = cos (t – 1) i + sinh (t – 1) j + at 2 k. If at t = 1, 

the acceleration of the particle be perpendicular to its position vector, then a is equal to 

1 

(a) 0 (b) 1 (c) 

2 

5.29 DIVERGENCE OF A VECTOR FUNCTION 

The divergence of a vector point function F 

 

(d) 

1 

2 

(AMIETE, Dec. 2009) Ans. (d) 

is denoted by div F and is defined as below. 

Let F = Fi 1 F 2 j Fk 3 

div F1 F2 F F = . F i j k ( iF1 jF2 kF3) 

= 3 

x y z 

x y z 

It is evident that div F is scalar function. 

5.30 PHYSICAL INTERPRETATION OF DIVERGENCE 

Let us consider the case of a fluid flow. Consider a small rectangular parallelopiped of 

dimensions dx, dy, dz parallel to x,y and z axes respectively. 

 

Let V Vx i Vy j Vz 

k 

be the velocity of the 

fluid at P(x, y, z). 

Mass of fluid flowing in through the face ABCD in unit time 

= Velocity × Area of the face = V x 

(dy dz ) 

Mass of fluid flowing out across the face PQRS per unit time 

= V x 

(x + dx) (dy dz) 

Vx 

 

= Vx 

dx ( dydz) 

x 

 

 

Y 

Net decrease in mass of fluid in the parallelopiped 

corresponding to the flow along x-axis per unit time 

O 

Z 

A 

C 

D 

dz 

V x B 

S 

P 

R 

Q 

X

Vectors 399 

Vx 

 

= Vx 

dydz Vx 

dx dy dz 

x 

 

 

 

Vx 

= dx dy dz 

(Minus sign shows decrease) 

x 

Vy 

Similarly, the decrease in mass of fluid to the flow along y-axis = dx dy dz 

y 

Vz 

and the decrease in mass of fluid to the flow along z-axis = dx dy dz 

z 

V V 

x y Vz 

 

Total decrease of the amount of fluid per unit time = dx dy dz 

x y z 

 

V 

V 

x y Vz 

Thus the rate of loss of fluid per unit volume = 

x y z 

= i j k 

.( iV 

x jV y kV 

 

z) 

= . V div V 

x y z 

If the fluid is compressible, there can be no gain or loss in the volume element. Hence 

div V = 0 ...(1) 

and V is called a Solenoidal vector function. 

Equation (1) is also called the equation of continuity or conservation of mass. 

 

xi yj 

zk 

Example 34. If v 

, 

2 2 2 

x y z 

find the value of div v . 


Solution. We have, v = 

 

xi yj 

zk 

2 2 2 

x y z 

div v = 

 

xi yj zk 

. v = i j k . 

 

x y z 2 2 2 1/2 

( x y z ) 

 

= 

x y z 

 

 

2 2 2 1/2 2 2 2 1/2 2 2 2 1/2 

x ( x y z ) y ( x y z ) z 

( x y z ) 

 

1 

2 2 2 1/2 1 

 

2 2 2 

( x y z ) x. ( x y z ) 

2 

.2x 

 

2 

 

= 

2 2 2 

( x y z ) 

 

1 1 

2 2 2 2 

1 

2 2 2 1/2 1 2 2 2 1/2 

 

2 2 2 

( x y z ) y. ( x y z ) 2 2y 

 

( x y z ) z. ( x y z ) .2z 

 

 

2 

 

2 

 

 

 

 

2 2 2 

2 2 2 

( x y z ) 

( x y z ) 

= 

= 

2 2 2 2 2 2 2 2 2 2 2 2 

( x y z ) x ( x y z ) y ( x y z ) z 

 

 

2 2 2 3/2 2 2 2 3/2 2 2 2 3/2 

( x y z ) ( x y z ) ( x y z ) 

2 2 2 2 2 2 

y z x z x y 

2 2 2 3/2 

( x y z ) 

= 

2 2 2 

2( x y z ) 2 

 

2 2 2 3/2 

( x y z ) 2 2 2 

( x y z ) 

 

Example 35. If u = x 2 + y 2 + z 2 , and r xi y j zk, 

then find div ( ur ) in terms of u. 

(A.M.I.E.T.E., Summer 2004) 

Ans.

400 Vectors 

Solution. div ( ur ) = 

 

 

2 2 2 

i j k .[( x y z )( xi yj 

zk)] 

x y z 

= 

 

 

2 2 2 2 2 2 2 2 2 

i j k .[( x y z ) xi( x y z ) yj( x y z ) zk] 

x y z 

= 

3 2 2 2 3 2 2 2 3 

( x xy xz ) ( x y y yz ) ( x z y z z ) 

x y z 

= (3x 2 + y 2 + z 2 ) + (x 2 + 3y 2 + z 2 ) + (x 2 + y 2 + 3z 2 ) = 5 (x 2 + y 2 + z 2 ) = 5 u Ans. 

Example 36. Find the value of n for which the vector 

 

r xi y j zk . 

Solution. Divergence F = 

= 

n 

r r is solenoidal, where 

n 2 2 2 n/2 

 

. F . r r .( x y z ) ( xi yj 

zk) 

 

 

2 2 2 n/2 2 2 2 n/2 2 2 2 n/2 

i j k .[( x y z ) xi( x y z ) yj( x y z ) zk] 

x y z 

= 2 

n (x 2 + y 2 + z 2 ) n/2 – 1 (2x 2 ) + (x 2 + y 2 + z 2 ) n/2 + 2 

n (x 2 + y 2 + z 2 ) n/2 – 1 (2y 2 ) 

+ (x 2 + y 2 + z 2 ) n/2 + 2 

n (x 2 + y 2 + z 2 ) n/2 – 1 (2z 2 ) + (x 2 + y 2 + z 2 ) n/2 

= n(x 2 + y 2 + z 2 ) n/2 – 1 (x 2 + y 2 + z 2 ) + 3 (x 2 + y 2 + z 2 ) n/2 

= n(x 2 + y 2 + z 2 ) n/2 + 3(x 2 + y 2 + z 2 ) n/2 = (n + 3) (x 2 + y 2 + z 2 ) n/2 

n 

If r r is solenoidal, then (n + 3) (x 2 + y 2 + z 2 ) n/2 = 0 or n + 3 = 0 or n = –3. Ans. 

 

 

Example 37. Show that 

( a. r) a n( a. r) 

r 

 

. (M.U. 2005) 

n n n 2 

r 

r r 


Let = 

 

 

x 

 

a. 

r 

r 

= 

n 

= 

 

But r 2 = x 2 + y 2 + z 2 r 

2r 

x 

 

( a i a j a k).( xi yj 

zk) 

1 2 3 

a. 

r ax 1 ay 2 az 

3 

 

n 

n 

r r 

n 

n1 

r . a ( ax a y az) nr ( r/ x) 

1 1 2 3 

2n 

n 

r 

r 

= 2x 

r x 

x 

r 

n 

= 

axa y az 

1 2 3 

n 

 

ar 1 ( ax 1 a2y az 3 ). nr . x a1 

nax ( 1 a2y a3zx 

) 

= 

2n 

= 

x 

r 

n 

n 2 

r r 

= 

 

i j k 

x y z 

= 

1 n 

 

( a1 i a 

n 

2 j a3k ) 

n 2 [( ax 1 a2y az 3 )( xi yj 

zk 

 

)] 

r 

r 

= 

a n 

( ar . ) r 

n n 2 

r r 

n 

2 

r

Vectors 401 

 

 

 

Example 38. Let r xi y j zk, r | r | and a is a constant vector. Find the value of 

 

 

a r 

div 

n 

r 

 

 

 

Solution. Let a = a 1 i a 2 j a 3 k 

= 

 

 

 

a r = ( a1i a2 j a3 

k) ( xi yj 

zk) 

 

 

a r 

 

| r | n 

 

a r 

div 

 

| r | n 

 

 

 

i j k 

a a a 

= 1 2 3 

= 

x y z 

 

= ( az 2 ayi 3 ) ( a1z a3x) j ( ay 1 axk 2 ) 

 

( az ayi ) ( a z a x) j ( ay 

axk ) 

2 3 1 3 1 2 

2 2 2 /2 

 

 

a r 

= . 

 

| r | n 

( x y z ) n 

 

( az 2 ayi 3 ) ( a1z a3x) j ( ay 1 axk 2 ) 

= i j k . 

x y z 

2 2 2 /2 

 

( x y z ) n 

az 2 ay 3 az 1 ax 3 ( ay 1 ax 2 ) 

 

 

2 2 2 n/2 2 2 2 n/2 2 2 2 n/2 

x ( x y z ) y ( x y z ) z 

( x y z ) 

n ( azay)2 x n ( a z a x)2 y n ( ay 

ax)2z 

 

n2 n2 n2 

2 2 2 

2 2 2 2 2 2 2 2 2 2 2 

( x y z ) ( x y z ) ( x y z ) 

2 

n 

[( azayx ) ( azaxy ) ( a y a xz ) ] 

= 

2 3 1 3 1 2 

= 

n 2 2 3 1 3 1 2 

2 2 2 2 

( x y z ) 

n 

[ azxa xy ayz a xy ayz a zx] 

= 0 Ans. 

= 

n 2 2 3 1 3 1 2 

2 2 2 2 

( x y z ) 

Example 39. Find the directional derivative of div ( u ) at the point (1, 2, 2) in the direction 

of the outer normal of the sphere x 2 + y 2 + z 2 

4 4 4 

= 9 for u x i y j z k 

. 

 

Solution. div ( u ) = . u 

 

4 4 4 3 3 3 

= i j k .( x i y j z k) 4x 4y 4z 

x y z 

Outer normal of the sphere = (x 2 + y 2 + z 2 – 9) 

 

 

2 2 2 

= i j k ( x y z 9) 2xi 2yj 

2zk 

x y z 

Outer normal of the sphere at (1, 2, 2) = 2i 4 j 4 k 

...(1) 

Directional derivative = 

= 

 

3 3 3 

(4x 4y 4 z ) 

 

 

 

i j k (4x 4y 4 z ) 12x i 12y j 12z k 

x y z 

 

3 3 3 2 2 2 

 

Directional derivative at (1, 2, 2) = 12 i 48 j 48 k 

...(2)

402 Vectors 

 

2i 4 j 4k 

Directional derivative along the outer normal = (12i 48 j 48 k). 

416 16 

[From (1), (2)] 

24 192 192 

= = 68 Ans. 

6 

Example 40. Show that div (grad r n ) = n (n + 1)r n – 2 , where 

2 2 2 

r = x y z 

2 1 

 

Hence, show that = 0. (U.P. I Semester, Dec. 2004, Winter 2002) 

r 

 

Solution. grad (r n 

n 

n n 

) = i r j r k r by definition 

x y z 

 

n 1 r 

n 1 r 

n 

1r 

 

n 1 r r 

r 

= inr j nr knr . = nr i j k 

x y z 

 

x y z 

 

n 1 x y z 

 

 

n2 n 2 

= nr i j k nr ( xi y j zk) nr r. 

r r r 

 

 

2 2 2 2 r r x 

 

r x y z 2r 2x etc. 

x x r 

 

 

 

 

Thus, grad (r n ) = n 2 n 2 n 2 

nr xi nr yj nr zk 

...(1) 

 

div grad r n = div [ n2 n2 n 2 

nr xi nr yj nr zk ] 

= 

= 

= 

 

 

n2 n 2 n 2 

i j k .( nr xi nr y j nr zk) 

x y z 

n 2 n 2 n 2 

( nr 

x) ( nr 

y) ( nr z) 

x y z 

n2 n3 r 

n2 n 3 

r 

 

nr nx ( n 2) r nr ny ( n 2) r 

x y 

 

nr nz ( n 

2) r 

 

n n r r r 

3 nr nn ( 2) r 

x y z 

 

 

x y z 

 

= 2 3 

[From (1)] 

(By definition) 

 

 

z 

 

n2 n3 

r 

n 2 n 3 x y z 

= 3 nr nn ( 2) r 

x y 

z 

 

r r r 

 

 

2 2 2 2 r r x 

 

r x y z 2r 2x etc. 

x x r 

 

 

 

= 3nr n – 2 + n (n – 2)r n – 4 [x 2 + y 2 + z 2 ] 

= 3nr n – 2 + n (n – 2) r n – 4 .r 2 ( r 2 = x 2 + y 2 + z 2 ) 

= r n – 2 [3n + n 2 – 2n] = r n – 2 (n 2 + n) = n(n + 1) r n – 2 

If we put n = –1 

div grad (r – 1 ) = –1 (–1 + 1) r – 1 – 2 

2 1 

 

 

r 

= 0 

 

r 

1 

Ques. If r xi y j zk, 

and r = |r| find div . 

2 (U.P. I Sem., Dec. 2006) Ans. 2 

r 

 

r

Vectors 403 

EXERCISE 5.8 

 

 

 

1. If r = xi yj zk 

r 

and r = | r | , show that (i) div 

3 

| r | 

 

= 0, 

 

(ii) div (grad r n ) = n (n + 1) r n – 2 (AMIETE, June 2010) (iii) div (r ) = 3 + r grad . 

 

2. Show that the vector V = ( x3 y) i ( y 3 z) j ( x 2 z) 

k is solenoidal. 

(R.G.P.V., Bhopal, Dec. 2003) 

3. Show that .( A) = .A + (.A) 

4. If , , z are cylindrical coordinates, show that grad (log ) and grad are solenoidal vectors. 

5. Obtain the expression for 2 f in spherical coordinates from their corresponding expression in 

orthogonal curvilinear coordinates. 

Prove the following: 

 

6. .( F) ( ). F ( . F ) 

 

7. (a) .() = 2 

(b) 

( A 

R) (2 n) A n( A. R) 

R 

, | | 

n n n 2 r R 

r r r 

8. div ( f g) – div (g f) = f 2 g – g 2 f 

5.31 CURL (U.P., I semester, Dec. 2006) 

The curl of a vector point function F is defined as below 

Curl F 

 

 

curl F = F 

 

= i j k ( F1 i F2 j F3 

k) 

x y z 

 

i j k 

= 

1 2 3 

is a vector quantity. 

5.32 PHYSICAL MEANING OF CURL 

 

 

 

( F F i F j F k) 

1 2 3 

F 

3 F2 

F 

3 F1 

F2 F1 

i j k 

x y z 

y z 

 

x z 

 

x y 

 

F F F 

(M.D.U., Dec. 2009, U.P. I Semester, Winter 2009, 2000) 

We know that V r, 

where is the angular velocity, V is the linear velocity and 

r 

is the position vector of a point on the rotating body. 

 

Curl V 1 i 2 j 3 

k 

 

= V 

 

r x i y j zk 

 

 

= ( r ) = [( 1i 2 j 3 

k) ( xi y j zk)] 

= 

 

i j k 

 

= 

[( 2z 3y) i ( 1z 3x) j ( 1y 2x) k] 

 

1 2 3 

x y z 

 

x y z 

 

= i j k 2z 3y i 1z 3x j 1y 2x k 

 

[( ) ( ) ( ) ]

404 Vectors 

= 

 

i j k 

 

x y z 

z y xz y x 

2 3 3 1 1 2 

 

= ( 1 2) i ( 2 2) j ( 3 3) 

k 

 

= 2( 1 i 2 j 3 

k) 2 

Curl V = 2 which shows that curl of a vector field is connected with rotational properties 

of the vector field and justifies the name rotation used for curl. 

If Curl F = 0, the field F is termed as irrotational. 

 

 

2 2 2 

Example 41. Find the divergence and curl of v ( xyz) i (3 x y) j ( xz y z) 

k at 

(2, –1, 1) (Nagpur University, Summer 2003) 


 

2 2 2 

 

v = ( xyz) i (3 x y) j ( xz y z) 

k 

Div. 

v = 

Div 2 2 2 

v = ( xyz) (3 x y) ( xz y z) 

x y z 

= yz + 3x 2 + 2x z – y 2 = –1 + 12 + 4 – 1 = 14 at (2, –1, 1) 

Curl v = 

 

i j k 

 

x y z 

2 2 2 

xyz 3x y xz y z 

 

2 

= 2 yz i ( xy z ) j (6 xy xz) 

k 

Curl at (2, –1, 1) 

 

= 2( 1)(1) i {(2) ( 1) 1} j {6(2)( 1) 2(1)} k 

Example 42. If 

 

= 

 

2 

2 yz i ( z xy) j (6 xy xz) 

k 

= 2i 3 j 14k 

Ans. 

 

xi yj 

zk 

V 

x y z 

2 2 2 , 

 

find the value of curl V . 

Solution. Curl V = V 

 

xi yj 

zk 

= i j k 

x y z 2 2 2 1/2 

( x y z ) 

 

= 

 

 

i j k 

 

x y z 

x y z 


2 2 2 1/2 2 2 2 1/2 2 2 2 1/2 

( x y z ) ( x y z ) ( x y z )

Vectors 405 

= 

z y 

z 

i 

j 

2 2 2 1/2 2 2 2 1/2 2 2 2 1/2 

y 

( x y z ) z 

 

 

 

 

 

( x y z ) 

 

x 

 

( x y z ) 

 

 

x y x 

k 

 

2 2 2 1/2 2 2 2 1/2 2 2 2 1/2 

z 

( x y z ) x 

 

( x y z ) y 

 

 

( x y z ) 

yz yz . zx zx 

 

( x y z ) ( x y z ) ( x y z ) ( x y z 

) 

xy 

xy 

k 

0 

2 2 2 3/2 2 2 2 3/2 

( x y z ) ( x y z 

) 

 

 

= i 

j 

 

2 2 2 3/2 2 2 2 3/2 2 2 2 3/2 2 2 2 3/2 

Example 43. Prove that 

2 2 

 

 

y z yz x i xz xy j xy xz z k 

Ans. 

( – 3 –2 ) (3 2 ) (3 –2 2 ) is both 

solenoidal and irrotational. (U.P., I Sem, Dec. 2008) 

Solution. Let F = 

2 2 

 

 

y z yz x i xz xy j xy xz z k 

( – 3 –2 ) (3 2 ) (3 –2 2) 

 

For solenoidal, we have to prove .F = 0. 

 

 

2 2 

 

Now, .F = i j k ( y – z 3 yz –2 x) i (3xz 2 xy) j (3 xy –2xz 2 z) 

k 

x y z 

 

 

 

= – 2 + 2x – 2x + 2 = 0 

Thus, F is solenoidal. For irrotational, we have to prove Curl F = 0. 

Now, Curl F = 

Thus, F is irrotational. 

 

i j k 

 

x y z 

2 2 

y – z 3 yz –2x 3xz 2xy 3 xy – 2xz 2z 

 

= (3z 2 y – 2y 3 z) i – (– 2z 3 y –3y 2 z) 

j 

 

= 0i 0 j 0 k = 0 

(3z 2 y – 2 y – 3) z k 

Hence, F is both solenoidal and irrotational. 

Proved. 

Example 44. Determine the constants a and b such that the curl of vector 

2 2 

 

A = (2xy 3 yz) i ( x axz –4 z ) j –(3 xy byz) 

k is zero. 

(U.P. I Semester, Dec 2008) 

Solution. Curl A = 

 

 

 

 

2 2 

i j k [(2xy 3 yz) i ( x axz – 4 z ) j 

x y z 

 

 

 

= 

 

i j k 

 

x y z 

2 2 

2xy 3 yz x axz –4 z –3 xy – byz 

 

 

 

(3 xy byz) k]

406 Vectors 

 

= [– 3 x – bz – ax 8 z] i –[– 3 y –3 y] j [2 x az –2 x –3 zk ] 

 

= [– x(3 a) z(8– b)] i 6 y j z(–3 a) 

k 

= 0 (given) 

i.e., 3 + a = 0 and 8 – b = 0, – 3 + a = 0 

a = – 3, 3 b = 8 a = 3 Ans. 

Example 45. If a vector field is given by 

 

2 2 

F ( x – y x) i – (2 xy y) 

j . Is this field irrotational ? If so, find its scalar potential. 


 

= 

2 2 

F 

Curl F = 

= 

= 

 

( x – y x) i – (2 xy y) 

j 

F 

 

 

 

 

 

 

2 2 

i j k ( x – y x) i – (2 xy y) 

j 

x y z 

 

i j k 

 

x y z 

2 2 

x – y x – 2 xy – y 0 

Hence, vector field F is irrotational. 

To find the scalar potential function 

F 

= 

 

 

(U.P. I Semester, Dec 2009) 

= i(0 –0)– j(0 –0) k(–2y 2 y) 

= 0 

d = dx dy 

 

 

dz = i j k ( i dx jdy kdz) 

x y z 

x y z 

 

 

 

 

 

= i j k ( d r ) 

x y z 

 

 

= dr = Fdr 

 

2 2 

= [( x – y x) i 

–(2 xy y) j]( i dx jdy kdz) 

= (x 2 – y 2 + x)dx – (2xy + y)dy. 

2 2 

= [( x – y x) dx –(2 xy ydy ) ] c 

= 

Hence, the scalar potential is 

 

 

2 2 

x d x xdx ydy y dx 2 xy dy 

c = 

 

Example 46. Find the scalar potential function f for 

Solution. We have, A = 

3 2 2 

x 2 

 

 

x – y – xy c 

3 2 2 

3 2 2 

x x y 2 

– – xy c 

Ans. 

3 2 2 

Curl A = A 

= 

 

2 2 

y i 2xy j z k 

 

2 2 

A y i 2xyj z k . 

(Gujarat, I Semester, Jan. 2009) 

 

2 2 

i j k ( y i 2 xyj 

z k) 

x y z

Vectors 407 

= 

 

i j k 

 

x y z 

2 2 

y 2xy z 

 

= i(0) j(0) k(2y 2 y) 

= 0 

Hence, A is irrotational. To find the scalar potential function f. 

 

A 

= f 

df = f dx 

f dy 

f 

dz 

x y z 

 

= i j k f. 

dr 

x y z 

 

= Adr . 

f f f 

 

= i j k .( i dx jdy kdz) 

x y z 

 

= f. 

dr 

 

2 2 

= ( y i 2 xy j z k).( i dx jdy kdz) 

= y 2 dx + 2xy dy – z 2 dz = d (xy 2 ) – z 2 dz 

 

= 

f = d 2 2 

( xy ) z dz 

xy 

2 

3 

(A = f) 

z 

C 

Ans. 

3 

Example 47. A vector field is given by 

A = (x 2 + xy 2 ) i + (y 2 + x 2 y) j . Show that the field 

is irrotational and find the scalar potential.(Nagpur Univeristy, Summer 2003, Winter 2002) 

Solution. A is irrotational if curl A = 0 

 

i j k 

Curl A = 

Hence, A 

 

A 

 

 

A 

x y z 

2 2 2 2 

x xy y x y 

is irrotational. If is the scalar potential, then 

= grad 

d = dx 

dy 

dz 

x y z 

 


= grad . dr 

x y z 

 

 

0 

 

2 2 2 2 

 

= i(0 0) j(0 0) k(2xy 2 xy) 0 

[Total differential coefficient] 

= Adr . = [( x xy ) i ( y x y) j].( idx jdy kdz) 

= (x 2 + xy 2 ) dx + (y 2 + x 2 y) dy = x 2 dx + y 2 dy + (x dx)y 2 + (x 2 ) (y dy) 

= 

2 2 2 2 

xdx y dy [( xdx ) y ( x )( y dy )] = 

 

2 2 

3 3 2 2 

x y x y 

c Ans. 

3 3 2 

Example 48. Show that V( x, y, z) 2 x yzi ( x z 2 y) 

j x yk is irrotational and find a 

scalar function u(x, y, z) such that V 

= grad (u). 

Solution. V (x, y, z) = 

 

2 2 

2 xyzi ( x z 2 y) 

j x yk

408 Vectors 

Curl V = 

= 

= 

 

 

2 2 

i j k [2 x yzi ( x z 2 y) j x yk] 

x y z 

 

i j k 

 

x y z 

2 2 

2xyz x z 2y x y 

2 2 

 

( x x ) i (2xy 2 xy) j (2xz 2 xz) k 0 

Hence, V (x, y, z) is irrotational. 

To find corresponding scalar function u, consider the following relations given 

V 

= grad (u) 

 

or V = ( u) 

...(1) 

u u u 

du = 

dx 

dy 

dz (Total differential coefficient) 

x y z 

u u u 

 

= i j k .( i dx jdy k dz) 

x y z 

 

 

= udr 

. V. 

d r 

[From (1)] 

 

2 2 

= [2 xyzi ( xz 2 y) j x yk].( i dx jdy kdz) 

= 2 x y z dx + (x 2 z + 2y) dy + x 2 y dz 

= y(2x z dx + x 2 dz) + (x 2 z) dy + 2y dy 

= [yd (x 2 z) + (x 2 z) dy] + 2y dy = d(x 2 yz) + 2y dy 

Integrating, we get u = x 2 yz + y 2 Ans. 

 

 

Example 49. A fluid motion is given by v ( y z) i ( z x) j ( x y) k. 

Show that the 

motion is irrotational and hence find the velocity potential. 

(Uttarakhand, I Semester 2006; U.P., I Semester, Winter 2003) 

Solution. Curl v = v 

 

 

 

= i j k [( y z) i ( z x) j ( x y) k] 

x y z 

= 

 

i j k 

 

x y z 

y z z x x y 

 

= (1 1) i (1 1) j (1 1) k 0 

Hence, 

v is irrotational. 

To find the corresponding velocity potential , consider the following relation. 

v = 

d = dx 

dy 

dz 

[Total Differential coefficient] 

x y z

Vectors 409 

 

 

= i j k .( idx jdykdz) 

= i j k . d r . 

d r = vdr . 

x y z 

 

x y z 

 

= [( y z) i ( z x) j ( x y) k].( i dx jdy kdz) 

= (y + z) dx + (z + x) dy + (x + y) dz 

= y dx + z dx + z dy + x dy + x dz + y dz 

 

= xy + yz + zx + c 

= ( ydx x dy) ( zdy y dz) ( zdx x dz) 

Velocity potential = xy + yz + zx + c 

Example 50. A fluid motion is given by 

 

v = (y sin z – sin x) i + (x sin z + 2yz) j + (xy cos z + y 2 ) k 

is the motion irrotational? If so, find the velocity potential. 

Solution. Curl v = v 

= 

= 

 

 

 

i j k ( ysin zsin x) i +( xsin z+2 yz) j+( xy cos z + y ) k 

x y z 

 

i j k 

 

2 

 

x y z 

ysin z sin x xsin z 2yz xy cos z y 

= (x cos z + 2y – x cos z – 2y) i – [y cos z – y cos z] j + (sin z – sin z) k = 0 

Hence, the motion is irrotational. 

So, 

2 

Ans. 

v = where is called velocity potential. 

d = dx 

dy 

dz 


x y z 

 


= .d r = v. 

dr 

x y z 

 

= [(y sin z – sin x) i + (x sin z + 2yz) j + (xy cos z + y 2 ) k 

].[ idx jdy kdz] 

= (y sin z – sin x) dx + (x sin z + 2 y z) dy + (x y cos z + y 2 ) dz 

= (y sin z dx + x dy sin z + x y cos z dz) – sin x dx + (2 y z dy + y 2 dz) 

= d (x y sin z) + d (cos x) + d (y 2 z) 

 

2 

= d ( xy sin z) d (cos x) d( y z) 

= xy sin z + cos x + y 2 z + c 

Hence, Velocity potential = xy sin z + cos x + y 2 z + c. Ans. 


 

Solution. Given F = 

Consider 

 

 

F = 

 

2 

F r r is conservative and find the scalar potential such that 

F = . (Nagpur University, Summer 2004) 

 

i j k 

 

x y z 

2 2 2 

r x r y r z 

2 

r r = 

 

2 2 2 

 

r 2 ( xi y j zk) 

= r xi r yj 

r zk

410 Vectors 

 

F 

= 0 

2 2 

2 2 

2 2 

= i r z r y j r z r x k r y r x 

y z 

x z 

 

 

x y 

 

 

r r r r r r 

= i 2rz 2ry j 2rz 2rx k 2ry 2rx 

y z 

x z 

 

 

x y 

 

 

2 2 2 2 r x r y r z 

But r x y z 

, , , 

x r y r z r 

 

 

 

y z x z x y 

= i 

 

2rz 2ry j 2rz 2rx k 2ry 2rx 

r r r r r r 

 

 

= i(2yz 2 yz) j(2zx 2 zx) k(2xy 2 xy) 

= 0i 0 j 0k 

0 

F is irrotational F is conservative. 

Consider scalar potential such that F = . 

d = dx 

dy 

dz 

x y z 

 


x y z 

 

 


x y z 

 

= F.( idx jdy kdz) 

= 

= 2 2 2 


 

= .( idx jdy kdz) 

 

2 

 

r r.( idx jdy kdz) 

( = F ) 

( x y z )( ix jy kz).( idx jdy kdz) 

= (x 2 + y 2 + z 2 ) (x dx + y dy + z dz) 

= x 3 dx + y 3 dy + z 3 dz + (x dx) y 2 + (x 2 ) (y dy) 

+ (x dx)z 2 + z 2 (y dy) + x 2 (z dz) + y 2 (z dz) 

3 3 3 2 2 

= x dx y dy z dz [( x dx) y ( y dy) x ] 

= 

 

4 4 4 

x y z 1 2 2 1 2 2 1 2 2 

x y xz y z c 

4 4 4 2 2 2 

 

2 2 2 2 

[( xdxz ) ( zdzx ) ] [( ydyz ) ( zdz) y ] 

= 1 4 (x4 + y 4 + z 4 + 2x 2 y 2 + 2x 2 z 2 + 2y 2 z 2 ) + c Ans. 

r 

Example 52. Show that the vector field F is irrotational as well as solenoidal. Find 

3 

| r | 

the scalar potential. 

(Nagpur University, Summer 2008, 2001, U.P. I Semester Dec. 2005, 2001) 

 

 

r xi y j zk 

Solution. F = 

2 2 2 3/2 

3 

| r | 

( x y z ) 

 

Curl F xi yj 

zk 

= F = i j k 

x y z 2 2 2 3/2 

( x y z )

Vectors 411 

= 

 

i j k 

 

x y z 

x y z 

2 2 2 3/2 2 2 2 3/2 2 2 2 3/2 

( x y z ) ( x y z ) ( x y z ) 

3 2yz 

3 2yz 

 

2 ( x y z ) 2 ( x y z ) 

 

= i 

 

2 2 2 5/2 2 2 2 5/2 

= 0 

Hence, F is irrotational. 

F = , where is called scalar potential 

d = dx 

dy 

dz 

x y z 

 


x y z 

 

 

xi yj 

zk 

= .( 

idx jdy kdz ) 

2 2 2 3/2 

( x y z ) 

1 2xdx 2y dy 2zdz 

= 

2 2 2 3/2 

2 

 

( x y z ) 

= 

Now, Div F 

= .F 

= 

3 2xz 

3 

2xz 

j 

 

2 

( x y z ) 2 

( x y z ) 

3 2xy 

3 

2xy 

k 

2 

( x y z ) 2 

( x y z ) 

1 

 

2 2 2 2 

 

 

 

2 2 2 5/2 2 2 2 5/2 

2 2 2 5/2 2 2 2 5/2 

 

 

 

 

 

 


 

= . dr Fdr . 

xdx y dy zdz 

= 2 2 2 3/2 

( x y z ) 

1 

2 

1 1 

( x y z ) 

1 

2 

1 

| r | 

2 2 2 

( x y z ) 2 

 

xi yj 

zk 

= i j k . ( 

2 2 2 ) 

3/2 

x y z 

x y z 

x y z 

 

 

x ( x y z ) y ( x y z ) z 

( x y z ) 

2 2 2 3/2 3 

2 2 2 1/2 

( x y z ) (1) x 

( x y z ) (2 x) 

2 

 

2 2 2 3 

( x y z ) 

= 

2 2 2 3/2 2 2 2 3/2 2 2 2 3/2 

Ans. 

2 2 2 3/2 3 

2 2 2 1/2 

( x y z ) (1) y 

( x y z ) (2 y) 

 

2 

 

2 2 2 3 

( x y z ) 

2 2 2 3/2 3 

2 2 2 1/2 

( x y z ) (1) z 

( x y z ) (2 z) 

 

2 

 

2 2 2 3 

( x y z )

412 Vectors 

= 

= 0 

2 2 2 1/2 

( x y z ) 

2 2 2 3 

( x y z ) 

[x 2 + y 2 + z 2 – 3x 2 + x 2 + y 2 + z 2 – 3y 2 + x 2 + y 2 + z 2 – 3z 2 ] 

Hence, F is solenoidal. 

Proved. 

 

2 2 2 2 

Example 53. Given the vector field V ( x y 2 xz) i ( xz xy yz) j ( z x ) k find 

curl V. Show that the vectors given by curl V at P 0 

(1, 2, –3) and P 1 

(2, 3, 12) are orthogonal. 

 

 

Solution. Curl V = V 

 

 

2 2 2 2 

= i j k [( x y 2 xz) i( xz xy yz) j( z x ) k] 

x y z 

curl V = 

 

i j k 

 

x y z 

2 2 2 2 

x y 2xz xz xy yz z x 

 

= ( x y) i (2x2 x) j ( z y 2 yk ) = ( x y) i ( y z) 

k 

 

curl V at P 0 

(1, 2, –3) = (1 2) i (23) k 3 

i k 

 

curl V at P 1 

(2, 3, 12) = (2 3) i (312) k 5i 15k 

The curl V 

at (1, 2, –3) and (2, 3, 12) are perpendicular since 

 

( 3 i k).( 5i 15 k) 

= +15 – 15 = 0 Proved. 

Example 54. Find the constants a, b, c, so that 

 

F = ( x2 y az) i ( bx 3 y z) j (4xcy 2 z) 

k 

...(1) 

is irrotational and hence find function such that F 

= . 

(Nagpur University, Summer 2005, Winter 2000; R.G.P.V., Bhopal 2009) 


 

F 

= 

As F is irrotational, 0 

F 

 

i j k 

 

x y z 

( x 2 y az) ( bx 3 y z) (4x cy 2) z 

 

= ( c 1) i (4 a) j ( b 2) k 

 

 

i.e., ( c 1) i (4 a) j ( b 2) k 0i 0 j 0k 

c + 1 = 0, 4 – a = 0 and b – 2 = 0 

i.e., a = 4, b = 2, c = –1 

Putting the values of a, b, c in (1), we get 

 

F = ( x 2y 4 z) i (2x 3 y z) j(4x y 2 z) 

k

Vectors 413 

Now we have to find such that F 

= 

We know that 

d = dx 

dy 

dz 

x y z 


= 

 

i j k .( i dx jdy kdz) 

x y z 

 

= 

 

 

i j k .( i dx jdy kdz) 

= .( idx jdy kdz) 

x y z 

 

= F.( i dx jdy kdz) 

 

= [( x 2y 4 z) i (2x3 y z) j (4x y 2 z) k)].( i dx jdy kdz) 

= (x + 2y + 4z) dx + (2x – 3y – z) dy + (4x – y + 2z) dz 

= x dx – 3y dy + 2z dz + (2y dx + 2x dy) + (4z dx + 4x dz) + (–z dy – y dz) 

( zdy 

ydz) 

= xdx3 ydy 2 zdz (2ydx 2 xdy) (4zdx 4 xdz) 

 

= 

2 2 

x 3y 

+ z 2 + 2xy + 4zx – yz + c Ans. 

2 2 

Example 55. Let V (x, y, z) be a differentiable vector function and (x, y, z) be a scalar 

function. Derive an expression for div ( V 

) in terms of .V , div V and . 

(U.P. I Semester, Winter 2003) 

 

Solution. Let V = V 1 i V 2 j V 3 k 

div ( V 

) = .( F) 

 

 

= i j k .[ V1 i V2 j V3 

k] 

= ( V1) ( V2) ( V3) 

x y z 

x y z 

V1 V2 

V3 

 

= V1 V2 V3 

x x y y 

z z 

 

 

 

V1 V2 

V3 

 

= V1 V2 V3 

x y z 

x y z 

 

 

 

= i j k .( V1 i V2 j V3 

k) 

i j k .( V1 i V2 j V3 

k) 

x y z 

x y z 

 

 

= ( . V) ( ). V (div V) (grad ). 

V 

Ans. 

Example 56. If A is a constant vector and R = x î + y ĵ + z ˆk , then prove that 

 

 

 

 

Curl A. 

R 

A A R 

(K. University, Dec. 2009) 

 

 

 

Solution. Let A = A1 î + A 2 

ĵ + A 3 

ˆk , R = xî + y ĵ + z ˆk 

 

A. 

R (A 1 î + A 2 

ĵ + A 3 

ˆk ) . (x î + y ĵ + z ˆk ) = A 1 

x + A 2 

y + A 3 

z 

 

[ A. R] 

R = (A1 x + A 2 

y + A 3 

z) (x î + y ĵ + z ˆk ) 

= (A 1 

x 2 + A 2 

xy + A 3 

zx) î + (A 1 

xy + A 2 

y 2 + A 3 

yz) ĵ + (A 1 xz + A 2 yz + A 3 z2 ) ˆk

414 Vectors 

iˆ 

ˆj kˆ 

 

Curl ( A. R) 

R 

= 

x y z 

2 2 2 

Ax Axy A zx Axy A y A yz Axz A yz Az 

L.H.S. = A 

R 

1 2 3 2 2 3 1 2 3 

= (A 2 

z – A 3 

y) î – [A 1 

z – A 3 

x) ĵ [A 1 y – A 2 x] ˆk ... (1) 

 

 

= (A 1 î + A 2 

ĵ + A 3 

k) ×(x î + y ĵ + z ˆk ) 

iˆ 

ˆj k 

A A A 

= 1 2 3 

x y z 

Example 57. Suppose that UV , 

= (A 2 

z – A 3 

y) î – (A 1 

z – A 3 

x) ĵ + (A y – A x) 1 2 

ˆk 

= R.H.S. [From (1)] 

 

 

and f are continuously differentiable fields then 

Prove that, div ( U V) Vcurl . U U. 

curl V . (M.U. 2003, 2005) 

 

Solution. Let U = ui 1 u2 ju3k, 

V v1i v2 j 

v3k 

 

 

U V = 

 

i j k 

u u u 

1 2 3 

v v v 

1 2 3 

 

= ( uv 2 3 uv 3 2) i ( u1v3 u3v1) j + ( uv 1 2 uv 2 1) 

k 

 

 

div ( U V) 

= i j k .[( u2v3u3v2) i( uv 1 3 uv 3 1) j+ ( u1v2u2v1) k] 

x y z 

 

= ( uv 2 3uv 3 2) ( uv 1 3uv 3 1) ( uv 1 2uv 

2 1) 

x y z 

v3 u2 v2 u3 

v3 

u1 

v1 

u3 

 

= u2 v3 u3 v2 u1 v3 

x x x x 

u3 v1 

y y 

y 

y 

 

 

v2 u1 v1 u2 

u1 v2 u2 v1 

z z z z 

 

 

 

u3 u2 u3 

u1 u2 u1 

 

= v1 v2 v3 

 

y z 

x z 

 

x y 

 

 

v3 v2 v3 

v1 v1 v2 

 

u1 u2 

u3 

 

y z 

x z 

 

y x 

 

 

u3 u2 u 1 u3 

 

u2 u1 

= ( v1i v2 j v3 

k). 

i j 

k 

 

y z z x 

 

x y 

 

 

 

v2 v3 v3 

v1 v1 v2 

 

( u1 i u2 j u3 

k). 

i j k 

z y x z 

 

y x 

 

 

 

 

= V.( U) U.( V) V.curl U U.curl 

V 

Proved.

Vectors 415 


 

 

( F G) 

= F( . G) G( . F) ( G. ) F ( F. ) G (M.U. 2004, 2005) 

Solution. 

 

( F G) 

= i ( F G) 

x 

= 

F G F G 

 

i G F i G i F 

 

x x x x 

 

= 

F F G 

G 

( iG . ) i G i F ( i. F) 

 

x x x x 

 

= 

F F G 

G 

( Gi . ) Gi. Fi. ( F. i) 

x x x x 

= 

G 

F 

F G 

F i Gi. ( G. i) ( F. i) 

x x x x 

 

= F ( G) G ( . F) ( G. ) F ( F. ) 

G 

Proved. 

Questions for practice: 

Prove that 

 

( FG . ) = ( G. ) F ( F. ) G G( F) F ( G) 

Example 59. Prove that, for every field V ; div curl V = 0. 

(Nagpur University, Summer 2004; AMIETE, Sem II, June 2010) 

 

Solution. Let V = V1 i V2 j V3 

k 

= 

 

div (curl V ) = .( V 

) 

 

i j k 

 

. 

x y z 

V V V 

1 2 3 

V3 V2 V 

3 V1 V 2 V1 

 

= i j k . 

i j 

k 

x y z y z x z x y 

 

V3 V2 V3 

V1 V2 V1 

 

= 

x y z y 

 

x z 

 

z x y 

 

2 2 2 2 2 2 

V3 V2 V3 

V1 V2 V1 

= 

xy xz yx yz zx zy 

 

2 2 2 2 

2 2 

V1 V 

1 V2 V 

2 V3 V 

3 

= 

 

yz zy zx xz xy yx 

 

= 0 Ans. 

Example 60. If a 

is a constant vector, show that 

 

 

 

a ( r) 

= ( a. r) ( a. ) r. 

(U.P., Ist Semester, Dec. 2007) 

 

Solution. a = a1 i a2 j a3k, 

r r1 i r2 j r3k

416 Vectors 

 

 

r = 

 

 

i j k 

 

x y z 

r r r 

= 

1 2 3 

 

i j k 

a ( r) 

= 

a1 a2 a3 

r3 r2 r3 

r1 r2 r1 

 

y z x z x y 

r 

r r 

r r r 

 

i j k 

y z 

 

x z 

 

x y 

 

 

3 2 3 1 2 1 

r2 r1 r3 r1 r2 r1 r3 

r2 

 

a2 a2 a3 a3 i a1 a1 a3 a3 

j 

= 

x y 

x z 

x y y z 

 

 

 

 

r3 r1 r3 

r2 

 

a1 a1 a2 a2 

k 

x z y z 

 

 

r 

1 r 

2 r3 r 

1 r 

 

2 r3 

 

= a1i a2 i a3 i a1 j a2 j a3 

j 

x x x y y y 

 

r 

1 r 

2 r3 

r 

1 r 

 

2 r3 

 

ak 1 a2k a3k 

a1 i a1 j ak 1 

z z z 

x x x 

 

r 

1 r 

2 r3 r 

1 r 

 

2 r3 

 

a2 i a2 j a2k a3 i a3 j a3k 

 

y y y z z z 

 

 

= i j k ( a11 r a2r2 a33 r ) a1 a2 a3 ( r1 i r2 j 

r3 

k) 

x y z x y z 

 

 

= ( a. r) ( a. ) 

r 

Proved. 

Example 61. If r is the distance of a point (x, y, z) from the origin, prove that 

1 1 

Curl k grad 

grad kgrad 

. 

= 0, where k is the unit vector in the direction OZ. 

r r (U.P., I Semester, Winter 2000) 

Solution. r 2 = (x – 0) 2 + (y – 0) 2 + (z – 0) 2 = x 2 + y 2 + z 2 

 

1 

r 

= (x 2 + y 2 + z 2 ) – 1/2 

grad 1 1 2 2 2 1/2 

= = i j k ( x y z ) 

r 

 

r x y z 

= 

1 ( 2 2 2 3/2 

x y z (2 xi 2 yj 2 zk 

) 

2 

k × grad 1 r 

2 2 2 3/2 

 

= – ( x y z ) ( xi yj 

zk) 

= 

2 2 2 3/2 

 

k [ ( x y z ) ( xi yj 

zk)] 

curl 

1 

k 

grad 

r 

= 

= 

2 2 2 3/2 

( x y z ) ( xj 

yi) 

1 

k 

grad 

r

Vectors 417 

 

= i j k 

x y z × [–(x2 + y 2 + z 2 ) –3/2 

( xj yi) 

] 

 

i j k 

 

= x y z 

y 

x 

0 

2 2 2 3/2 2 2 2 3/2 

( x y z ) ( x y z ) 

3 ( x)(2) z 3 y (2 z) 3 ( x)(2 x) 

= 

i j 

2 2 2 5/2 2 2 2 5/2 

2 2 2 5/2 

2 ( x y z ) 2( x y z ) 2 ( x y z ) 

1 ( 3/2) ( y)(2 y) 1 

k 

2 2 2 3/2 2 2 2 5/2 2 2 2 3/2 

( x y z ) ( x y z ) ( x y z ) 

2 2 2 2 2 2 2 2 

3xz 3 yz (3x x y z 3 y x y z 

) 

= 

i 

j 

k 

2 2 2 5/2 2 2 2 5/2 2 2 2 5/2 

( x y z ) ( x y z ) ( x y z ) 

 

2 2 2 

 

3xz i 3 yzj ( x y 2 z ) k 

= 

2 2 2 5/2 

( x y z ) 

...(1) 

k . grad 1 r = 

2 2 2 3/2 

z 

k.[ ( x y z ) ( xi yj zk)] 

 

2 2 2 3/2 

( x y z ) 

1 z 

grad k.grad 

= i j k 2 2 2 3/2 

r x y z 

( x y z ) 

= 

 

 

3 i( z)(2 x) 3 j( z)(2 y) 

 

 

2 

2 2 2 5/2 

2 

2 2 2 5/2 

( x y z ) ( x y z 

) 

3 ( z)(2 z) 1 

 

 

k 

2 2 2 5/2 2 2 2 3/2 

2 

 

( x y z ) ( x y z 

) 

 

2 2 2 2 2 2 2 

= 3xz i 3 yzj(3 z x y z ) k 3xz i 3 yzj( x y 2 z ) k 

 

2 2 2 5/2 2 2 2 5/2 

( x y z ) ( x y z ) 

...(2) 

Adding (1) and (2), we get 

1 1 

Curl kgrad 

grad k.grad 

 

 

r r = 0 Proved. 

 

 

a r (2 n) a n( a. r) 

r 

Example 62. Prove that 

. 

n n n 2 

r r r 

 

(M.U. 2009, 2005, 2003, 2002; AMIETE, II Sem. June 2010) 


 

a r 

r 

n 

 

1 

i j k 

a a a 

= 

n 1 2 3 

r 

 

x y z 

1 1 1 

 

= ( az 2 ayi 3 ) ( a3x a1z) j ( ay 1 axk 2 ) 

n n n 

r r r

418 Vectors 

 

i j k 

 

 

( a r ) 

n 

= x y z 

r 

azay ax az ay 

ax 

2 3 3 1 1 2 

n n n 

r r r 

 

ay 1 ax 2 ax 3 az 1 

ay 1 ax 

2 az 2 ay 

3 

= i j 

n 

n n n 

y r z 

 

 

r x r z 

 

 

r 

ax 3 az 1 az 2 ay 3 

k 

n 

n 

x 

 

 

r y 

 

 

r 

Now, r 2 = x 2 + y 2 + z 2 

r 

r 

x 

2r 

= 2x 

x 

x 

r 

Similarly, 

r 

y 

= , 

r 

z 

y 

r 

z 

r 

 

a r 

 

n 

1 

y 

1 

= i . ( n 

r 

nr a1y a2x) 

a 

n 1 

r 

r 

 

n 

1 

z 

1 

nr ( ax 3 az 1 ) ( a1 

) 

r 

n + two similar terms 

 

r 

= 

 

n 2 a1 n 

2 a1 

i ( a 

2 1y a2xy) ( axz 

2 3 a1z 

) 

n 

n n 

n 

r r r r 

+ two similar terms 

= 

 

2a1 

n 2 2 n 

 

i a 

2 1( y z ) ( axy 

2 2 a3xz) 

n n 

n 

 


r r r 

 

Adding and subtracting 

n 2 

ax to third and from second term, we get 

n 2 

r 

1 

 

a r 

 

 

2a1 na1 

2 2 2 n 2 

 

n 

r 

= i ( x y z ) ( ax 

2 2 1 axy 2 a3xz) 

n n 

n 

 

r r r 

 

 


2a1 na1 

2 n 

 

= i r xax ( 

2 2 1 a2y a3 

z) 

n n 

n 


r r r 

 

2a1 na1 

n 

 

 

2a2 na2 

n 

 

= i x( ax 

2 1 ay 2 az 3 ) 

n n n 

 

j 

y( a 

2 2 y az 3 ax 1 ) 

n n n 

 

r r r 

r r r 

 

2a3 na3 

n 

 

k 

z( az 

2 3 ax 1 a2 

y) 

n n n 

 

r r r 

 

2 n n 

= ( a 

n 1 i a2 j a3k ) 

n ( a1 i a2 j a3k 

) ( 2 1 2 3 )( 

ax a y az xi yj 

zk ) 

n 

r 

r 

r 

2 n n 

 

= ( a1 i a2 j a3k) ( ax 

2 1 a2y az 3 )( xi yj 

zk) 

n 

n 

r 

r 

2 n n 

= a ( a. r) 

r 

n n 

2 

Proved. 

r r 

Example 63. If f and g are two scalar point functions, prove that 

div (f g) = f 2 g + f g. (U.P., I Semester, compartment, Winter 2001)

Vectors 419 

g g g 

 

Solution. We have, g = i j k 

x y z 

g g g 

 

f g = f i f j f k 

x y z 

g g g 

 

div (f g) = f 

f 

f 

x x y y z z 

 

 

2 2 2 

g g g f g f g f g 

= f 

 

 

2 2 2 

x y z 

 

 

x x y y z z 

 

 

2 2 2 

f f f g g g 

 

= f 

g i j k . i j k 

2 2 2 

x y z 

 

 

x y z x y z 

 

= f 2 g + f.g Proved. 

Example 64. For a solenoidol vector F , show that curl curl curl curl F = 4 

F . 

(M.D.U., Dec. 2009) 

Solution. Since vector F is solenoidal, so div F = 0 ... (1) 

We know that curl curl F = grad div ( F – 2 

F ) ... (2) 

Using (1) in (2), grad div F = grad (0) = 0 ... (3) 

On putting the value of grad div F in (2), we get 

curl curl F = – 2 

F ... (4) 

Now, curl curl curl curl F = curl curl (– 2 

F ) [Using (4)] 

= – curl curl ( 2 

F ) = – [grad div ( 2 

F ) – 2 ( 2 

F ) ] [Using (2)] 

= – grad ( . 2 

F ) + 2 

( 2 

F ) = – grad ( 2 

. F 

 

) + 4 

F [ . F = 0] 

= 0 + 4 F = 4 F [Using (1)] Proved. 

EXERCISE 5.9 

1. Find the divergence and curl of the vector field V = (x 2 – y 2 ) i + 2xy j + (y 2 – xy) k . 

Ans. Divergence = 4x, Curl = (2y – x) i + y j + 4y k 

2. If a is constant vector and r is the radius vector, prove that 

 

 

(i) ( a. r) 

a (ii) div ( r a) 0 (iii) curl ( r 

a) 2 

a 

where r 

 

= xi yj zk and a a1i a2 j a3k 

. 

3. Prove that: 

(i) .(A) = .A + (.A) 

(ii) (A.B) = (A.)B + (B.)A + A × ( × B) + B × ( × A) (R.G.P.V. Bhopal, June 2004) 

(iii) × (A × B) = (B.)A – B(.A) – (A.)B + A(.B) 

4. If F = (x + y + 1) i + j – (x + y) k , show that F.curl F = 0. 

(R.G.P.V. Bhopal, Feb. 2006, June 2004) 

Prove that 

 

 

5. ( F) ( ) F ( F ) 

6. .( F G) G.( F) F.( G) 

 

7. Evaluate div ( A r) 

if curl A 

= 0. 8. Prove that curl ( a r) 

= 2a

420 Vectors 

9. Find div F and curl F where F = grad (x 3 + y 3 + z 3 – 3xyz). (R.G.P.V. Bhopal Dec. 2003) 

Ans. div F = 6(x + y + z), curl F = 0 

10. Find out values of a, b, c for which v = (x + y + az) i + (bx + 3y – z) j + (3x + cy + z) k 

is irrotational. 

Ans. a = 3, b = 1, c = –1 

11. Determine the constants a, b, c, so that F = (x + 2y + az) i + (bx – 3y – z) j + (4x + cy + 2z) k is 

irrotational. Hence find the scalar potential such that F = grad . 

(R.G.P.V. Bhopal, Feb. 2005) Ans. a = 4, b = 2, c = 1 

Choose the correct alternative: 

Potential = 

12. The magnitude of the vector drawn in a direction perpendicular to the surface 

x 2 + 2y 2 + z 2 = 7 at the point (1, –1, 2) is 

2 2 

x 3y 

2 

 

z 2xy yz 4zx 

 

2 2 

 

2 

3 

(i) 

(ii) (iii) 3 (iv) 6 (A.M.I.E.T.E., Summer 2000) Ans. (iv) 

3 

2 

13.If u = x 2 – y 2 + z 2 and V xi yj zk 

then ( uV ) is equal to 

(i) 5u (ii) 5| V | (iii) 5( u | |) (iv) 5( u | |) (A.M.I.E.T.E., June 2007) 

14.A unit normal to x 2 + y 2 + z 2 = 5 at (0, 1, 2) is equal to 

1 

(i) ( ) 

5 i j k 

1 

(ii) ( ) 

5 i j k 

1 

(iii) ( 2 ) 

5 j 

k 1 

(iv) ( ) 

5 i j k 

 

 

V 

V 

(A.M.I.E.T.E., Dec. 2008) 

15.The directional derivative of = x y z at the point (1, 1, 1) in the direction i is: 

1 

(i) –1 (ii) 

1 

(iii) 1 (iv) 

Ans. (iii) 

3 

3 

(R.G.P.V. Bhopal, II Sem., June 2007) 

 

 

16.If r xi y j zk and r = | r | then (r) is: 

(i) (r) r 

(ii) 

 

() 

r r 

r 

(iii) 

 

() 

r r 

r 

(iv) None of these 

Ans. (iii) 

(R.G.P.V. Bhopal, II Semester, Feb. 2006) 

17. If 

r = xi yj zk is position vector, then value of (log r) is (U.P., I Sem, Dec 2008) 

 

 

 

r 

r 

(i) 

(ii) 

(iii) – r (iv) none of the above. Ans. (ii) 

2 

3 

r 

r 

r 

 

18. If r xi y j zk and | r | 

= r, then div r is: 

(i) 2 (ii) 3 (iii) –3 (iv) –2 Ans. (ii) 

(R.G.P.V. Bhopal, II Semester, Feb. 2006) 

 

2 

 

2 

 

2 

 

19. If V xy i 2yx zj 3yz k then curl V at point (1, –1, 1) is 

 

 

 

 

(i) ( j 2 k) 

(ii) ( i 3 k) 

(iii) ( i 2 k) 

(iv) ( i 2 j k) 

(R.G.P.V. Bhopal, II Semester, Feb 2006) 

Ans. (iii) 

20. If A is such that A = 0 then A is called 

(i) Irrotational (ii) Solenoidal (iii) Rotational (iv) None of these 

(A.M.I.E.T.E., Dec. 2008) 

21. If F is a conservative force field, then the value of curl F is 

(i) 0 (ii) 1 (iii) F (iv) –1 (A.M.I.E.T.E., June 2007)

Vectors 421 

5.33 LINE INTEGRAL 

 

Let y, 

F ( x, z) 

be a vector function and a curve AB. 

Line integral of a vector function F along the curve AB is defined as integral of the component 

of F along the tangent to the curve AB. 

Component of F along a tangent PT at P 

= Dot product of F 

 

and unit vector along PT 

 

 

 

dr dr 

= F is aunit vector along tangent PT 

ds ds 

 

 

 

 

dr 

Line integral = F from A to B along the curve 

ds 

 

 

 

 

dr 

Line integral = F 

ds 

c 

ds 

= 

 

F 

 

dr 

 

 

c 

 

Note (1) Work. If F represents the variable force acting on a particle along arc AB, then the 

total work done = 

 

B 

F 

 

A 

 

dr 

(2) Circulation. If V represents the velocity of a liquid then 

V 

 

c 

 

dr is called the circulation 

of V round the closed curve c. 

If the circulation of V round every closed curve is zero then V is said to be irrotational there. 

(3) When the path of integration is a closed curve then notation of integration is in place 

 

of . 

22.If 2 

[(1 – x) (1 – 2x)] is equal to 

(i) 2 (ii) 3 (iii) 4 (iv) 6 (A.M.I.E.T.E., Dec. 2009) Ans. (iii) 

23.If R = xi + yj + zk and A 

is a constant vector, curl ( A R ) is equal to 

(i) R (ii) 2 R (iii) A (iv) 2 A (A.M.I.E.T.E., Dec. 2009) Ans. (iv) 

1 

24. If r is the distance of a point (x, y, z) from the origin, the value of the expression ˆj grad 2 

equals 

(i) 2 2 2 

3 

 

2 ˆ 

( x y z ) ( ˆjz kx) 

(ii) 

(iii) zero (iv) 

 

2 

Example 65. If a force F 2x yiˆ 3xyj 

ˆ displaces a particle in the xy-plane from (0, 0) to 

(1, 4) along a curve y = 4 x 2 . Find the work done. 

Solution. Work done = F 

. dr 

 

 

c r xiˆ yj ˆ 

 

 

2 

 

= 

ˆ ˆ ˆ ˆ 

(2 x yi 3 xyj).( dx i dyj) 

dr dxiˆdyj 

ˆ 

 

= 

 

c 

c 

2 

(2 x y dx 3 xy dy) 

3 

 

2 2 2 

( x y z ) 2 ( ˆjz 

iz ˆ ) 

3 

 

2 2 2 2 ˆ 

( x y z ) ( ˆjy 

kx) 

(AMIETE, Dec. 2010) Ans. (ii)

422 Vectors 

Putting the values of y and dy, we get 

 

1 2 2 2 

= [2 x (4 x ) dx 3 x (4 x )8 xdx] 

0 

5 

1 

1 4 

x 104 

= 104 x dx 104 

 

0 

5 

5 

0 

 

2 

y 4 x 

 

dy 8 xdx 

 

Ans. 

Example 66. Evaluate 

 

C 

 

F. 

drwhere F x 

2ˆ i xyj ând C is the boundary of the square in the 

plane z = 0 and bounded by the lines x = 0, y = 0, x = a and y = a. 


 

 

Solution. F. dr F. dr F. dr F. dr F. 

dr 

C OA AB BC CO 

 

Here r xiˆ yj ˆ, dr dxiˆ dyj ˆ, F x 

2ˆ i xyj ˆ 

On OA, y = 0 2 

F. 

dr x dx 

 

F. 

dr = x 2 dx + xydy ...(1) 

 

 

F . dr = 

OA 

On AB, x = a dx = 0 

(1) becomes 

 

F. 

dr = aydy 

2 

a 

3 

F 

. dr 

 

= a y a 

aydy a 

 

 

Ab 

0 

2 2 

...(3) 

0 

On BC, y = a dy = 0 

(1) becomes 2 

F. 

dr x dx 

 

 

 

F . dr = 

BC 

On CO, x = 0, F. dr 0 

(1) becomes 

 

 

 

 

 

3 

0 

3 

0 2 

x – a 

xdx 

a 

3 3 

...(4) 

 

 

a 

F . dr = 0 ...(5) 

CO 

3 3 3 3 

On adding (2), (3), (4) and (5), we get F 

. dr 

 

= 

a a – a 0 a 

Ans. 

C 3 2 3 2 

Example 67. A vector field is given by 

 

F = (2y 3) iˆ 

xzj ˆ ( yz – x) kˆ 

. Evaluate F 

. dr 

 

along the path c is x = 2t, 

C 

y = t, z = t 3 from t = 0 to t = 1. (Nagpur University, Winter 2003) 

 

 

 

C 

 

 

a 

3 3 

a 2 

x a 

xdx 

0 

3 3 

...(2) 

0 

Solution. F . dr = (2y 3) dx ( xz) dy ( yz – x) 

dz 

C C 

 

3 

Since x 2t y t z t 

 

 

dx dy dz 2 

2 1 3t 

 

 

dt dt dt 

Y 

O 

B 

A 

X

Vectors 423 

1 3 4 2 

= (2 t 3) (2 dt ) (2)( t t ) dt ( t – 2)(3 t t dt ) = 

0 

 

2 

t 2 5 3 7 6 4 

= 4 6 t t t – t 

2 5 7 4 

1 

0 

 

1 4 6 3 

(4t 6 2t 3 t – 6 t ) dt 

0 

2 2 5 3 7 3 4 

= 2t 6 t t t – t 

5 7 2 

 

 

 

2 3 3 

= 2 6 – = 7.32857. Ans. 

5 7 2 

Example 68. The acceleration of a particle at time t is given by 

a 

= 18 cos 3tiˆ 

8sin 2tj ˆ 6 tk ˆ . 

If the velocity v and displacement r be zero at t = 0, find v and r at any point t. 

2 

Solution. Here, a d r 

= 

2 

dt 

On integrating, we have 

 

= 18 cos 3tiˆ 

8sin 2tj ˆ 6 tkˆ 

. 

 

v = dr 

iˆ 

18 cos 3t dt ˆj 8sin 2t dt k ˆ 6t dt 

dt 

 

2 

v = 6sin 3tiˆ 4cos 2tj ˆ 3t kˆ 

 

c ...(1) 

At t = 0, v = 0 

Putting t = 0 and 

v = 0 in (1), we get 

 

0 = 4ˆj 

c c 4ˆj 

 

dr 

2 

v = 6sin 3tiˆ 4(cos 2t 1) ˆj 3tkˆ 

dt 

Again integrating, we have 

2 

r 

= iˆ 

6sin 3 ˆ 4(cos 2 1) ˆ 

t dt j t dt k3t dt 

3 

r = 2cos 3 tiˆ(2sin 2t 4 t) 

ˆj t kˆ 

c 

...(2) 

At, t = 0, r = 0 

Putting t = 0 and r = 0 in (2), we get 

 

 

0 = 2iˆC ˆ 

1 C1 

2i 

Hence, r 

3 

= 2(1cos 3) t iˆ 

2(sin 2t 2 t) 

ˆj t kˆ 

Ans. 

Example 69. If A 2 2 

(3x 6 y) iˆ 

–14yzj ˆ 20 xz kˆ 

, evaluate the line integral Adr . from 

(0, 0, 0) to (1, 1, 1) along the curve C. 

x = t, y = t 2 , z = t 3 . (Uttarakhand, I Semester, Dec. 2006) 


 

 

A . dr = 

C 

= 

 

 

C 

C 

2 2 

[(3x 6 y) iˆ –14yzj ˆ 20 xz kˆ].[ iˆ dx ˆjdy kˆ 

dz] 

2 2 

[(3x 6 y) dx –14yzdy 20 xz dz] 

If x = t, y = t 2 , z = t 3 , then points (0, 0, 0) and (1, 1, 1) correspond to t = 0 and t = 1 respectively. 

 

 

Now, A . dr = 

C 

= 

 

t 1 2 2 2 3 2 3 2 3 

t 0 

[(3t 6 t ) d ( t)–14 t t d ( t ) 20 t ( t ) d ( t )] 

t 1 2 5 7 2 

[9 t dt –14 t .2tdt 20 t .3 t dt] 

= 

t 0 

 

1 

1 2 6 9 

0 

1 

0 

 

 

(9 t –28 t 60 t ) dt

424 Vectors 

= 

 

3 7 10 

t t t 

 

9 – 28 60 

3 7 10 

 

 

 

1 

0 

= 3 – 4 + 6 = 5 Ans. 


 

S 

 

2 

A. nˆ 

ds where A ( x y ) iˆ 

– 2xj ˆ 2yzkˆ 

and S is the surface of 

the plane 2x + y + 2z = 6 in the first octant. (Nagpur University, Summer 2000) 

Solution. A vector normal to the surface “S” is given by 

 

(2x y 2) z = ˆ 

 

ˆ 

ˆ 

i j k (2x y 2) z 2iˆ ˆj 2kˆ 

 

x y z 

And ˆn = a unit vector normal to surface S 

Z 

2iˆ 

ˆj 2kˆ 

2 1 2 N 

= 

i ˆ ˆ j k ˆ 

41 

4 3 3 3 

K 

kˆ 

2 1 2 2 

n 

. n ˆ = ˆ 

k. 

iˆ 

ˆj kˆ 

– 

 

3 3 3 3 

O 

M Y 

3 

A . nds 

dx dy 

R 

ˆ = A. 

nˆ 

S 

R 

k ˆ L 

. n 

Where R is the projection of S. 

X 

Now, A . nˆ 

= [( x y 2 ) iˆ – 2xj ˆ 2 yzkˆ]. 

2 

iˆ 1 ˆj 2 kˆ 

 

3 3 3 

2 2 2 4 2 2 4 

= ( x y ) – x yz y yz 

...(1) 

3 3 3 3 3 

Putting the value of z in (1), we get 

on the plane 2x y 2z 

6, 

 

A. 

nˆ 

2 2 4 6 

2 x y 

 

 

= y y 

(6 2 x y) 

3 3 2 z 

 

2 

 

 

A. 

nˆ 

= 2 y ( y 6 –2 x – y) 4 y (3 – x) 

...(2) 

3 3 

M 

Hence, 

A 

. nds 

dx dy 

ˆ = A. 

n 

S 

R 

| k ˆ 

...(3) 

. n | 

 

Putting the value of A. 

nˆ 

from (2) in (3), we get 

A 

 

. nds 4 3 

3 62x 

ˆ = (3 – ). 2 (3 ) 

S y x dx dy y x dydx 

R 3 2 

 

0 0 

= 

 

3 

0 

 

2 

y 

2(3– x) 

 

2 

6–2x 

0 

dx 

= 

3 (3 – )(6 –2 ) 2 4 3 

(3 – ) 

3 

0 0 

4 

3 

x x dx x dx 

(3 – x) 

 

= 4. –(0–81) 81 

4(–1) 

0 

 

iy ˆ ˆjx 

Example 71. Compute F. dr, 

where F 

c 

2 2 

and c is the circle x 2 + y 2 = 1 traversed 

x y 

counter clockwise. 

O 

2x + 3y = 6 

L 

Ans. 

X

Vectors 425 

Solution. r = ix ˆ ˆjy kz ˆ , dr idx ˆ ˆjdy kdz ˆ 

 

F . dr 

iy ˆ ˆjx 

= ( ˆ ˆ ˆ ) 

c idx jdy kdz 

c 2 2 

x y 

ydx xdy 

= ( ydx xdy) 

c 2 2 

x y 

c 

Parametric equation of the circle are x = cos , y = sin . 

Putting x = cos , y = sin , dx = – sin d , dy = cos d in (1), we get 

Fd 

 

r 

2 

= sin ( sin d ) cos (cos d ) 

C 

= 

...(1) [ x 2 + y 2 = 1] 

0 

2 

2 2 

2 

= 2 0 

(sin cos ) d d 

0 0 

 

2 Ans. 

 

2 3 2 2 2 

Example 72. Show that the vector field F 2 x( y z ) iˆ 

2x yj ˆ 3xzkˆ 

is conservative. 

Find its scalar potential and the work done in moving a particle from (–1, 2, 1) to (2, 3, 4). 

(A.M.I.E.T.E. June 2010, 2009) 


 

2 3 ˆ 2 ˆ 2 2 

F 2 x( y z ) i 2x yj 3x z kˆ 

 

 

Curl F F 

iˆ 

ˆj kˆ 

 

 

x y z 

2 3 2 2 2 

2 xy ( z ) 2x y 3x z 

(00) i(6xz 6 xz ) ˆj(4xy 4 xy) 

k = 0 

Hence, vector field F is irrotational. 

To find the scalar potential function 

 

F 

 

d dx dy 

dz ˆ 

 

ˆ 

ˆ 

 

i j k . idx ˆ ˆjdy kdz ˆ 

 

x y z x y z 

 

 

ˆ 

 

ˆ 

 

i j kˆ 

 

 

 

. d r . d r F. 

dr 

x y z 

2 3 2 2 2 

2 xy ( z ) iˆ2x yj ˆ3 x z kˆ 

( idx ˆ ˆjdy kdz 

ˆ ) 

 

 

= 

2 2 ˆ 

 

 

2 3 2 2 2 

2 x( y z ) dx2x ydy3x z dz 

2 3 2 2 2 

2 ( ) 2 3 

 

xy z dx x ydy x z dz C 

2 2 3 2 2 

(2 xy dx 2 x ydy ) (2 xz dx 3 x z dz ) + C = x 2 y 2 + x 2 z 3 + C 

Hence, the scalar potential is x 2 y 2 + x 2 z 3 + C 

Now, for conservative field 

Work done = 

 

(2,3, 4) (2,3, 4) 

 

F. 

d r d 

( 1,2,1) ( 1, 2,1) 

 

 

(2,3,4) (2,3,4) 

2 2 2 3 

x y x z c 

( 1,2,1) 

( 1,2,1) 

 

= (36 + 256) – (2 – 1) = 291 Ans.

426 Vectors 

 

Example 73. A vector field is given by F (sin y) iˆ x(1 cos y) ˆj. 

Evaluate the line integral 

over a circular path x 2 + y 2 = a 2 , z = 0. `(Nagpur University, Winter 2001) 


F 

 

C 

Work done = . dr 

F 

 

C 

 

= [(sin ) ˆ (1 cos ) ˆ].[ ˆ ˆ 

y i x y j dxi dyj] 

( z = 0 hence dz = 0) 

 

C 

. d r = sin ydx x (1 cos y ) dy (sin y dx x cos y dy xdy ) 

C 

C 

 

= d ( xsin y) 

x dy 

C 

(where d is differential operator). 

The parametric equations of given path 

x 2 + y 2 = a 2 are x = a cos , y = a sin , 

Where varies form 0 to 2 

F 

 

C 

. d r = 

= 

 

 

 

C 

2 

2 

d [ a cos sin ( a sin )] a cos . a cos d 

0 0 

2 

2 

2 2 

d [ a cos sin ( a sin )] a cos . 

d 

0 0 

= [ a cos sin ( asin )] a cos d 

 

 

 

 

2 

2 

2 2 

0 0 

2 

2 21cos 2 a sin 2 

= 0 a 

d 

0 

 

2 2 

 

2 

 

0 

2 

a 

2 

= .2a 

2 

Example 74. Determine whether the line integral 

Ans. 

2 2 2 2 

(2 xyz ) dx ( x z z cos yz) dy (2x yz y cos yz) 

dz is independent of the path of 

 

integration ? If so, then evaluate it from (1, 0, 1) to 

 

0, ,1 . 

2 

2 2 2 2 

Solution. (2 xy z ) dx ( x z z cos yz) dy (2x yz y cos yz) 

dz 

= 

 

c 

 

c 

2 2 2 2 

[(2 xy ziˆ) ( x z z cos yz) ˆj (2x yz y cos yz) kˆ].( idx ˆ ˆjdy kdz ˆ ) 

= Fdr 

 

 

c 

This integral is independent of path of integration if 

F 

= 0 

iˆ 

ˆj kˆ 

F = 

F 

 

 

x y z 

2 2 2 2 

2xyz x z z cos yz 2x yz y cos yz 

= (2x 2 z + cos yz – yz sin yz – 2x 2 z – cos yz + yz sin yz) = iˆ 

–(4 xyz – 4 xyz) ˆj (2 xz –2 xz ) k 

= 0 

Hence, the line integral is independent of path. 

d = dx 

dy 

dz 

x y z 

(Total differentiation) 

2 

2 2 ˆ

Vectors 427 

 

B A 

 

ˆ 

ˆ 

= 

ˆ 

 

ˆ 

 

 

i j k ( idx ˆ ˆjdy kdz) 

= dr F 

d r 

x y z 

2 2 2 2 

= [(2 xyz ) iˆ( x z z cos yz) ˆj (2x yz ycos yz) kˆ]. ( idx ˆ ˆjdy kdz ˆ ) 

= 2xyz 2 dx + (x 2 z 2 + z cos y z) dy + (2x 2 yz + y cos yz) dz 

= [(2x dx) yz 2 + x 2 (dy) z 2 + x 2 y (2z dz)] + [(cos yz dy) z + (cos yz dz) y] 

= d (x 2 yz 2 ) + d (sin yz) 

= 

 

 

2 2 2 2 

d ( x yz ) d (sin yz) x yz sin yz 

= (B) – (A) 

2 2 2 2 

= [ x yz sin yz] [ x yz sin yz] 

(1, 0,1) = 

 

0 sin ( 1) [0 0] 

(0, ,1) 

 

2 

2 

 

 

 

= 1 Ans. 

 

Example 75. Evaluate A. nˆ 

dS, where A 18 ziˆ 

–12ˆj 3ykˆ 

 

and S is the part of the 

S 

plane 2x + 3y + 6z = 12 included in the first octant. (Uttarakhand, I semester, Dec. 2006) 

Solution. Here, A = 18 ziˆ 

–12ˆj 3ykˆ 

Given surface f (x, y, z) = 2x + 3y + 6z – 12 

 

ˆ 

Normal vector = f = 

ˆ 

 

ˆ 

 

i j k (2x 3y 6 z –12) = 2iˆ3ˆj 6kˆ 

x y z 

ˆn = unit normal vector at any point (x, y, z) of 2x + 3y + 6z = 12 

2iˆ3ˆj 6kˆ 

1 

= 

(2iˆ3ˆj 6 kˆ 

) 

4936 

7 

dx dy dx dy dxdy 7 

dS = dx dy 

nˆ . kˆ 1 

ˆ ˆ ˆ ˆ 6 6 

(2i 3 j 6 k). 

k 

7 7 

Now, A 

. ndS 

1 7 

 

ˆ = (18 ˆ –12 ˆ 3 ˆ). (2ˆ 3ˆ 

6 ˆ 

zi j yk i j k) 

dx dy 

7 6 

dx dy 

= (36 z –36 

18 y) 

= (6 z –6 

3 y) 

dx dy 

6 

Putting the value of 6z = 12 – 2x – 3y, we get 

Y 

= 

= 

= 

= 

1 

6 (12 –2 x) 

3 

0 0 

 

 

(12–2 x –3 y –6 

3 y) 

dxdy 

1 

6 (12 –2 x) 

3 

0 0 

1 

6 (12 –2 x) 

3 

(6 –2 x) 

dx 

0 0 

1 

6 

(12–2 x ) 

(6 –2 x) dx( y) 

3 

0 0 

 

(6 –2 x) 

dxdy 

dy 

6 1 

1 6 

2 

= (6 –2 x) (12 –2 x) 

dx = (4 x –36x 72) dx 

0 

0 

3 

3 

 

3 

6 

14x 

2 

 

–18x 

72x 

3 

3 

= 1 [4 36 2–18 36 72 6] = 72 [4 –9 6] 24 

Ans. 

3 

3 

0 

O 

B 

2x + 3y = 12 

A 

X

428 Vectors 

EXERCISE 5.10 

1. Find the work done by a force yiˆ xj ˆ which displaces a particle from origin to a point ( iˆ ˆj). 

Ans. 1 

2. Find the work done when a force 2 2 

F ( x – y x) iˆ(2 xy y) 

ˆj 

moves a particle from origin to 

(1, 1) along a parabola y 2 = x. Ans. 2 3 

3. Show that 

 

 

3 ˆ 

2 ˆ 

2 ˆ is a conservative field. Find its scalar potential such that 

V (2 xy z ) i x j 3xz k 

V = grad . Find the work done by the force V in moving a particle from (1, – 2, 1) to (3, 1, 4). 

Ans. x 2 y + xz 3 , 202 

4. Show that the line integral 2 

(2xy 3) dx ( x 4 z) dy 4ydz 

 

c 

where c is any path joining (0, 0, 0) to (1, – 1, 3) does not depend on the path c and evaluate the line 

integral. Ans. 14 

2 2 

x y 

5. Find the work done in moving a particle once round the ellipse 1 , z = 0, under the field of 

25 16 

force given by F = (2x – y + z) î + (x + y – z2 ) ĵ + (3x – 2y + 4z) k ˆ. Is the field of force conservative? 

(A.M.I.E.T.E., Winter 2000) Ans. 40 

6. 

If 4 

= (y 2 – 2xyz 3 ) î + (3 + 2xy – x 2 z 3 ) ĵ + (z 3 – 3x 2 yz 2 z 2 2 3 

) k ˆ, find . Ans. 3y xy x yz 

 

4 

R . dR 

7. is independent of the path joining any two point if it is. (A.M.I.E.T.E., June 2010) 

C 

Ans. (i) 

(i) irrotational field (ii) solenoidal field (iii) rotational field (iv) vector field. 

5.34 SURFACE INTEGRAL 

A surface r = f(u, v) is called smooth if f (u, v) posses continous 

first order partial derivative. 

Let F be a vector function and S be the given surface. 

Surface integral of a vector function F over the surface S is defined 

as the integral of the components of F 

along the normal to the 

surface. 

Component of F along the normal 

= F . ˆn , where n is the unit normal vector to an element ds and 

grad f 

ˆn = 

|grad f | 

Surface integral of F over S 

Note. (1) Flux = 

 

ds = 

dx dy 

( nˆ 

kˆ 

) 

= Fn ˆ 

= ( Fnˆ 

) ds 

 

S 

 

 

( F nˆ 

) ds where, F represents the velocity of a liquid. 

S 

 

 

If 

( Fn ˆ) 

ds = 0, then F is said to be a solenoidal vector point function. 

S 

 

 


( ˆ ˆ ˆ 

yzi zxj xyk) 

ds where S is the surface of the sphere 

S 

x 2 + y 2 + z 2 = a 2 in the first octant. (U.P., I Semester, Dec. 2004) 

Solution. Here, = x 2 + y 2 + z 2 – a 2

Vectors 429 

Vector normal to the surface = = 

iˆ 

ˆj kˆ 

 

x y z 

 

ˆ 2 2 2 2 

ˆ 

= 

ˆ 

 

ˆ 

 

i j k ( x y z a ) 2xiˆ 2yj ˆ 2zk 

x y z 

ˆn 

2xiˆ 2yj ˆ 2zkˆ 

xiˆ yj ˆ zkˆ 

= 

 

| | 2 2 2 2 2 2 

4x 4y 4z x y z 

xiˆ 

yj ˆ zkˆ 

= 

[ x 2 + y 2 + z 2 = a 2 ] 

a 

Here, F = yz iˆ 

zxj ˆ xy kˆ 

 

ˆ ˆ ˆ 3 

Fn ˆ = ( ˆ ˆ ˆ 

xi yj zk xyz 

yz i zxj xy k) 

 

 

a 

 

a 

Now, F 

n ˆ ds 

 

2 2 

dx dy a a x 3xyz dx dy 

S 

= ( Fn ˆ) 

 

S 

| kˆ 

. nˆ 

 

| 

00 

z 

a 

a 

a 

2 

x 

2 

2 

a a 

2 

x 

2 

a y 

= 3 xy dy dx 3 x dx 

0 

0 0 2 

 

= 

0 

2 2 4 

a 

4 4 4 

3 a 2 2 3 a x x 3 a a 3a 

2 

x a x dx 0 

2 

2 4 

 

2 2 4 

8 

0 

( ) . 

3 

Example 77. Show that Fnds ˆ , 

S 

where 

2 

F = 4 xz î – y 2 ĵ + yz ˆk 

and S is the surface of the cube bounded by the planes, 

x= 0, x = 1, y = 0, y = 1, z = 0, z = 1. 

Solution. 

 

 

 

= 

ˆ 

Fnds ˆ 

S 

Fnds 

 

 

OABC 

Fnds ˆ 

Fnds 

ˆ 

 

DEFG 

 

 

OAGF 

 

Fnds ˆ 

Fnds 

ˆ 

BCED 

 

Fnds 

 

OCEF 

 

ABDG 

ˆ 

...(1) 

Now, 

 

DEFG 

Fnds 

 

 

OABC 

 

2 

= (4 ˆ ˆ ˆ 

xzi y j yz k)( k) 

dx dy = 1 1 

OABC 

2 

(4 xziˆ 

y ˆj yz kˆ) 

kˆ 

dx dy 

= 

 

DEFG 

2 

1 

1 y 

 

1 

= dx [ x] 

0 

0 

0 

2 ˆ 

1 1 

 

yz dx dy y (1) dxdy 

0 0 

1 1 

 

2 2 2 

(4 ˆ ˆ 

xz i y j yzk) ( j) 

dxdz = 

OAGF 

0 0 

Ans. 

S.No. Surface Outward ds 

normal 

1 OABC – k dx dy z = 0 

2 DEFG k dx dy z = 1 

3 OAGF – j dx dz y = 0 

4 BCED j dx dz y = 1 

5 ABDG i dy dz x = 1 

6 OCEF – i dy dz x = 0 

yz dx dy 0 

(as z = 0) 

2 

y dxdz 0 

(as y = 0) 

OAGF

430 Vectors 

(4 xz iˆ y ˆj yzk) 

ˆ 

jdxdz = 

BCED 

2 ˆ 

= 

2 ˆ 

 

BCED 

1 dx 1 dz x 1 1 

0 0 

0 z 0 

(4 xziˆ y ˆj yzk) 

idydz 

ˆ 

 

= 

ABDG 

= 

2 

( y ) dxdz 

(as y = 1) 

( ) ( ) 1 

 

2 

1 

1 

z 1 

 

0 

4( y) 

4(1) 

2 

2 

2 

(4 ˆ ˆ )( ˆ 

xz i y j yzk i) 

dydz = 

OCEF 

On putting these values in (1), we get 

= 

F 

nds ˆ 

S 

1. Evaluate A 

. n ˆ ds , 

S 

2 ˆ 

0 

1 1 

 

4xz dy dz 4 (1) zdydz 

1 1 

0 0 

1 

0 01 2 0 = 3 2 

2 

EXERCISE 5.11 

0 0 

where A = 2 

( x y ) iˆ 

2xj ˆ 2yzkˆ 

4xz dy dz 0 

(as x = 0) 

Proved. 

and S is the surface of the plane 

2x + y + 2z = 6 in the first octant. Ans. 81 

2. Evaluate A 

. n ˆ ds , 

S 

where A = 2 

ziˆ xj ˆ 3y zkˆ 

and S is the surface of the cylinder x 2 + y 2 = 16 

included in the first octant between z = 0 and z = 5. Ans. 90 

3. If r = 

tiˆ t ˆ j ( t 1) k 

2 

ˆ 

and S = 

2ˆ 

tkˆ 

evaluate 

2ti 

6 , 

2 

r S dt. 

Ans. 12 

0 

4. Evaluate FndS 

 

ˆ , where, F = 18 ziˆ12 ˆj 3ykˆ 

and S is the surface of the plane 2x + 3y + 6z = 12 

S 

in the first octant. Ans. 24 

5. Evaluate Fnds 

 

ˆ , 

2 

where, F = 2yxiˆ yzj ˆ xkˆ 

over the surface S of the cube bounded by the 

S 

coordinate planes and planes x = a, y = a and z = a. 

 

2 

6. If F 2yiˆ 

3ˆj xkˆ 

and S is the surface of the parabolic cylinder y 

2 

= 8x in the first octant bounded 

 

by the planes y = 4, and z = 6, then evaluate FndS ˆ . 

Ans. 132 

S 

5.35 VOLUME INTEGRAL 

Let F be a vector point function and volume V enclosed by a closed surface. 

The volume integral = 

F 

dv 

V 

Example 78. If F = 2 z î – x ĵ + y ˆk , evaluate 

the surfaces 

x = 0, y = 0, x = 2, y = 4, z = x 2 , z = 2. 

Solution. 

(2 ziˆ 

xj ˆ yk) 

dx dy dz 

 

= ˆ 

 

= 

= 

F 

dv 

V 

2 4 2 

0 0 x 

2 

dx dy (2 ziˆ 

xj ˆ ykˆ 

) dz 

= 

 

2 4 4 3 2 

dx dy [4iˆ 2xj ˆ 2 ykˆ 

x iˆ x ˆj x ykˆ] 

 

0 0 

Ans. 

1 4 

2 a 

Fdv 

 

where, v is the region bounded by 

V 

 

2 4 2 

2 

dx ˆ ˆ ˆ 

0 dy [ z i xzj yzk] 0 

x 

2

Vectors 431 

= 

 

2 

0 

 

2 2 

ˆ ˆ 2 ˆ 4 ˆ 3 

4 2 

ˆ 

x y 

dx yi xyj yk x yi x yj kˆ 

 

 

 

2 

2 4 3 2 

= ˆ ˆ ˆ ˆ ˆ ˆ 

(16i 8xj 16k 4xi 4x j 8 xk) 

dx 

0 

2 

 

5 3 

2 4 4 8 

= ˆ ˆ ˆ x 

16 4 16 ˆ ˆ 

x 

xi x j xk i x j kˆ 

 

 

5 3 0 

128 64 

= 32 ˆ 16 ˆ 32 ˆ ˆ 16 ˆ ˆ 32 iˆ 

32kˆ 

i j k i j k = = 32 (3iˆ 

5 kˆ 

) 

5 3 5 3 15 

EXERCISE 5.12 

1. If F 

= 2 

(2x 3) z iˆ2xyj ˆ 4 xkˆ 

, then evaluate FdV , where V is bounded by the plane 

V 

4 

0 

Ans. 

x = 0, y = 0, z = 0 and 2x + 2y + z = 4. Ans. 8 3 

2. Evaluate dV , 

V where = 45 x 2 y and V is the closed region bounded by the planes 

4x + 2y + z = 8, x = 0, y = 0, z = 0 Ans. 128 

3. If F = (2x 2 

– 3z) iˆ 

2xyj ˆ 4 xkˆ 

, then evaluate FdV 

, where V is the closed region bounded 

V 

8 

by the planes x = 0, y = 0, z = 0 and 2x + 2y + z = 4. 

Ans. ( ˆ ˆ) 

3 j k 

4. Evaluate (2 x 

y) dV , where V is closed region bounded by the cylinder z = 4 – x 2 and the planes 

V 

x = 0, y = 0, y = 2 and z = 0. Ans. 80 3 

5. If F 2 

= 2 ˆ ˆ ˆ 

 

xz i xj y k, 

evaluate 

F dV over the region bounded by the surfaces x = 0, y = 0, 

y = 6 and z = x 2 , z = 4. Ans. (16iˆ3ˆj 48 kˆ 

) 

5.36 GREEN’S THEOREM (For a plane) 

 

Statement. If (x, y), (x, y), and be continuous functions over a region R bounded 

y 

x 

by simple closed curve C in x – y plane, then 

( dx 

dy) 

= 

 

dx dy 

C 

R 

 

x 

y 

(AMIETE, June 2010, U.P., I Semester, Dec. 2007) 

Proof. Let the curve C be divided into two curves C 1 

(ABC) and C 1 

(CDA). 

Let the equation of the curve C 1 

(ABC) be y = y 1 

(x) and equation of the curve C 2 

(CDA) be 

y = y 2 

(x). 

Let us see the value of 

 

dx dy = xc 

y 

y 

2 

( x) 

 

dy dx 

R y 

xa y y ( x) 

 

1 y 

 

= c 2 ( ) 

( , ) 

y 

x y 

y x 

dx 

a 

y y ( x) 

1 

c 

a 

c 

= ( x, y2) ( x, y1) 

dx = ( x, y2) dx ( x, y1) 

dx 

a 

a 

c 

= 

 

( x, y2) dx ( x, y1) 

dx 

 

c 

a 

 

= 

 

( x , y ) dx ( x , y ) dx 

 

 

c2 c1 

 

= – ( , ) 

 

c 

c 

 

a 

x y dx

432 Vectors 

Thus, 

dx 

c 

= 

Similarly, it can be shown that 

 

dx dy 

...(1) 

R y 

 

dy 

c 

= dx dy ...(2) 

x 

On adding (1) and (2), we get 

 

 

( dx 

dy) 

= 

dx dy 

R 

Proved. 

x 

y 

 

Note. Green’s Theorem in vector form 

where, 

 

 

Fdr 

 

= ( F ) ˆ 

c 

R 

k dR 

 

F iˆ ˆj, r xiˆ yj ˆ, 

kˆ 

is a unit vector along z-axis and dR = dx dy. 

 

Example 79. A vector field F is given by F sin yiˆ x (1 cos y) ˆj. 

Evaluate the line integral Fdr 

 

where C is the circular path given by x 2 + y 2 = a 2 . 

C 

 

Solution. F sin yiˆ x(1 cos y) 

ˆj 

Fdr 

 

 

C 

= [sin ˆ (1 cos ) ˆ] ( ˆ ˆ 

yi x y j idx jdy) 

C 

= sin ydx x (1 cos y) 

dy 

C 

On applying Green’s Theorem, we have 

 

 

( dx dy) 

c 

= 

dx dy 

s 

 

x 

y 

 

= [(1 

cos y) cos y] 

dx dy 

s 

where s is the circular plane surface of radius a. 

= dx dy 

s 

= Area of circle = a 2 . Ans. 

2 2 

Example 80. Using Green’s Theorem, evaluate ( x ydx x dy), 

where c is the boundary 

described counter clockwise of the triangle with vertices (0, 0), (1, 0), (1, 1). 


Solution. By Green’s Theorem, we have 

Y 

A 

 

 

(1, 1) 

= 

( dx dy) 

c 

= dx dy 

R 

 

x 

y 

 

2 2 

( x y dx 

2 

x dy) 

= (2 x x ) dxdy 

c 

1 2 

(2 x x ) dx dy = 

0 0 

x 

= 

 

 

R 

1 2 

0 

(2 x x ) dx[ y]x 

1 2 

(2 x x )( x ) dx = 

0 

2 1 

= 

3 4 = 5 

12 

Example 81. State and verify Green’s Theorem in the plane for 

c 

0 

1 2 3 

(2 x x ) dx = 

0 

 

2 2 

(0, 0) 

(1, 0) 

 

3 4 

2 x x 

 

 

3 4 

 

1 

0 

Ans. 

(3 x –8 y ) dx (4 y – 6 xy) 

dy where C is the boundary of the region bounded by x 0, y 0 and 2x – 3y = 6. 

(Uttarakhand, I Semester, Dec. 2006) 

O 

y = x 

X

Vectors 433 

Solution. Statement: See Article 24.4 on page 576. 

Here the closed curve C consists of straight lines OB, BA and AO, where coordinates of A and 

B are (3, 0) and (0, – 2) respectively. Let R be the region bounded by C. 

Then by Green’s Theorem in plane, we have 

 

2 2 

[(3 x –8 y ) dx (4 y –6 xy) dy ] 

= 

 

 

x 

 

y 

2 2 

(4 y –6 xy)– (3 x –8 y ) dxdy 

R 

 

 

...(1) 

 

= (– 6y 16 y) dx dy 10 ydxdy 

R 

2 

3 0 3 y 

= 10 dx 1 ydy 10 dx 

 

x 

2 

0 (2 –6) 0 

3 1 

(2 x –6) 

3 

 

= 

3 

5 (2 x – 6) 5 3 

= – – (0 6) 

9 3 

2 54 

0 

Now we evaluate L.H.S. of (1) along OB, BA and AO. 

Along OB, x = 0, dx = 0 and y varies form 0 to –2. 

Along BA, x = 1 (6 3 y), 

dx 3 dy and y varies from –2 to 0. 

2 2 

and along AO, y = 0, dy = 0 and x varies from 3 to 0. 

L.H.S. of (1) = 

= 

 

OB 

 

2 2 

[(3 x – 8 y ) dx (4 y –6 xy) dy] 

3 

2 2 2 2 

BA 

R 

 

0 

 

 

= 

5 3 

– (2 – 6) 

9 

dx x 

0 

5 

– (216) –20 ...(2) 

54 

[(3 x – 8 y ) dx (4 y –6 xy) dy] [(3 x – 8 y ) dx (4 x –6 xy) dy ] 

 

 

AO 

2 2 

[(3 x – 8 y ) dx (4 y –6 xy) dy ] 

–2 0 3 2 23 

 

0 

2 

= 4 ydy (6 3 y) –8 y dy [4 y –3(6 3 y) y] dy 3x dx 

0 –2 

 

4 

 

2 

 

 

3 

= 

2 –2 0 9 

2 2 2 3 0 

[2 y ] 

 

0 

 

(63 y) –12y 4 y –18 y –9 y dy ( x ) 

3 

–2 

8 

 

 

 

0 9 

2 2 

= 2[4] 

(6 3 y) –21 y –14 y dy (0 –27) 

–2 

8 

 

 

 

0 

 

3 

9(6 3 y) 3 2 

216 

3 2 

= 

8 – 7 y – 7 y –27 –19 7(– 2) 7(–2) 

8 3 3 8 

 

 

 

–2 

= – 19 + 27 – 56 + 28 = – 20 ...(3) 

With the help of (2) and (3), we find that (1) is true and so Green’s Theorem is verified. 

2 2 2 2 

Example 82. Apply Green’s Theorem to evaluate [(2 x y ) dx ( x y ) dy], 

where C 

is the boundary of the area enclosed by the x-axis and the upper half of circle x 2 + y 2 = a 2 . 

(M.D.U. Dec. 2009, U.P., I Sem., Dec. 2004) 

2 2 2 2 

Solution. [(2 x y ) dx ( x y ) dy] 

 

C 

By Green’s Theorem, we’ve ( dx 

dy) 

= 

C 

 

S 

C 

 

 

dx dy 

x 

y 

2

434 Vectors 

= 

a a 

2 

x 

2 

 

a 

0 

a a 

2 

x 

2 

a 

0 

2 2 2 2 

( x y ) (2 x y ) dx dy 

x 

y 

 

a a 

2 

x 

2 

= (2x 

2 y) 

dx dy = 2 dx ( x y ) dy 

a 

2 

x 

2 

2 

= 2 a y 

dx 

xy 

a 

2 = 2 

 

= 

 

a 

0 

 

a 

a 

2 2 a 2 2 

a 2 x a x dx ( a x ) dx 

a 

 

a 

0 

 

2 2 

2 2 a x 

 

x a x 

dx 

2 

 

 

 

 

 

 

3 

a 

3 

2 x 

3 a 

2 2 

= 0 2 a ( a x ) dx = 2 a x 

2 

a 

3 

 

0 

3 3 

= 4a 

3 

0 

 

a 

a 

( ) 2 ( ) , is even 

 

 

 

 

0, is odd 

f x dx f x dx f 

a 0 

 

f 

Example 83. Evaluate y x 

dx dy, 

where C C 

C 2 2 2 2 

1UC2 

x y x y 

with C : x2 + y2 = 1 

1 

and C 2 

: x = 2, y = 2. (Gujarat, I Semester, Jan 2009) 

y 

x 

Solution. dx 

C 2 2 2 2 

x y x y 

dy 

Y 

x y 

= 

 

dx dy 

x 

2 2 

y 

2 2 

x y x y 

 

 

 

2 2 2 2 

( x y )1–2 x( x) ( x y )1–2 y ( y) 

 

X 

 

 

= 

 

dx dy 

2 2 2 2 2 2 x = – 2 

( x y ) ( x y ) 

 

2 2 2 2 2 2 

x y –2 x x y –2y 

 

= 

 

dx dy 

2 2 2 2 2 2 

( x y ) ( x y ) 

2 2 2 2 

= y – x x – y 

 

dx dy 

2 2 2 2 2 2 

( x y ) ( x y ) 

= 0 

dx dy 0 

( x 

2 y 

2 ) 

2 

5.37 AREA OF THE PLANE REGION BY GREEN’S THEOREM 

Proof. We know that 

Mdx Ndy = 

N M 

– dx dy 

A 

 

x y 

C 

 

...(1) 

 

N 

 

M 

On putting N = x 1 

and M = – y 1 

in (1), we get 

x 

y 

– ydx xdy = [1 –(–1)] dx dy 

A 

= 2 dx dy = 2 A 

C 

Area = 1 ( – ) 

2 

xdy ydx 

C 

Example 84. Using Green’s theorem, find the area of the region in the first quadrant bounded 

by the curves 

y = x, y = 1 x 

, y = (U.P. I, Semester, Dec. 2008) 

x 4 

Solution. By Green’s Theorem Area A of the region bounded by a closed curve C is given by 

O 

Y 

y = 2 

x 2 + y 2 = 1 

y = – 2 

Ans. 

X 

x = 2 

Ans.

Vectors 435 

A = 1 Y 

( xdy – ydx) 

2 

C 

x 

Here, C consists of the curves C 1 

: y = , C 

4 2 

: y = 1 x 

and C 3 

: y = x So 

1 1 1 

A 

 

 

 

( I1 I2 I3 

) 

C 

 

2C 2 C 

1 C 

2 C3 

 

2 

 

2 

 

 

C 

x 1 

3 C 1 

Along C 1 

: y = , dy dx , x :0to 2 

x 

y = — 

4 4 

4 

1 x 

I 1 

= ( xdy – ydx) x dx – dx 0 

C 

1 C 

 

 

1 

4 4 

Along C 2 

: y = 1 , dy – 1 

O (0, 0) 

dx, x :2to1 

2 

x x 

1 

1 1 

I 2 

= ( xdy – ydx) 

x – 

dx – 

dx 

C 

2 

2 

2 

x 2 

 

= 

1 

[– 2log x] 2log 2 

2 

Along C 3 

: y = x, dy = dx ; x : 1 to 0 ; 

I 3 

= 

 

C3 

 

( xdy – ydx) ( xdx – xdx) 0 

A = 1 ( I 1 

1 I2 I3) (0 2log 2+0) log 2 

Ans. 

2 2 

EXERCISE 5.13 

2 2 

1. Evaluate 

[(3x 6 yz) dx(2y 3 xz) dy (1 4 xyz ) dz] 

from (0, 0, 0) to (1, 1, 1) along the path c 

c 

given by the straight line from (0, 0, 0) to (0, 0, 1) then to (0, 1, 1) and then to (1, 1, 1). 

2 2 3 

2. Verify Green’s Theorem in plane for ( x 2 xy) dx ( y x y) dy, 

where c is a square with the 

C 

1 

vertices P (0, 0), Q (1, 0), R (1, 1) and S (0, 1). 

Ans. 

2 

2 2 

3. Verify Green’s Theorem for ( x 2 xy) dx ( x y 3) dy around the boundary c of the region 

c 

y 2 = 8 x and x = 2. 

2 2 2 2 

4. Use Green’s Theorem in a plane to evaluate the integral 

[(2 x y ) dx ( x y ) dy] 

, 

c 

where c is the boundary in the xy-plane of the area enclosed by the x-axis and the semi-circle x 2 + y 2 =1 

in the upper half xy-plane. Ans. 4 3 

5. Apply Green’s Theoem to evaluate [( y sin x) dy cos xdy], 

c 

where c is the plane triangle enclosed 

 

2 x 

2 

8 

by the lines y = 0, x = and y . 

Ans. 

2 

 

4 

6. Either directly or by Green’s Therorem, evaluate the line integral 

e x (cos y dx sin ydy), 

c 

 

where c is the rectangle with vertices (0, 0), (, 0,), , and 0, . 

Ans. 2 (1 – e – ) 

2 2 

(AMIETE II Sem June 2010) 

2 

7. Verify the Green’s Theorem to evaluate the line integral (2 y dx 3 x dy), 

where c is the boundary 

c 

of the closed region bounded by y = x and y = x 2 . 

(U.P., I Semester, Dec. 20005, AMIETE Summer 2004, Winter 2001) Ans. 27 4 

y = x 

B (1, 1) 

y = — 1 x 

A (2, —) 

1 

2 

X

436 Vectors 

8. Evaluate F . nds ˆ. , 

s 

and s is the region of the plane 2x + 2y + z = 6 

 

where 2 

F xyiˆ x ˆ j ( x z) 

kˆ 

in the first octant. (A.M.I.E.T.E., Summer 2004, Winter 2001) Ans. 27 4 

9. Verify Green’s Theorem for 

2 2 

( xy y ) dx x dy 

 

C 

where C is the boundary by y = x and y = x 2 . 

(AMIETE, June 2010) 

5.38 STOKE’S THEOREM (Relation between Line Integral and Surface Integral) 

(Uttarakhand, I Sem. 2008, U.P., Ist Semester, Dec. 2006) 

Statement. Surface integral of the component of curl F along the normal to the surface S, 

taken over the surface S bounded by curve C is equal to the line integral of the vector point function 

F taken along the closed curve C. 

Mathematically 

 

 

F . d r = curl Fnds ˆ 

S 

where ˆn = cos î + cos ĵ + cos ˆk is a unit 

external normal to any surface ds, 

Proof. Let r = xiˆ 

yj ˆ 

zkˆ 

dr = idx ˆ ˆj dy kdz ˆ 

F = Fi ˆ 

ˆ 

1 F ˆ 2 j F3 k 

 

On putting the values of F, 

d r in the statement of the theorem 

 

c 

( Fi ˆ ˆ ˆ ˆ ˆ ˆ 

1 F2 j Fk 3 ) ( idx j dy kdz) 

= 

i j k 

S 

 

x y z 

( Fiˆ ˆ ˆ ˆ ˆ ˆ 

 

1 F 2 j F3 k). (cos i cos j cos k) 

ds 

( F1 dx 

F3 F2 1 3 

2 1 

F2 dy F3 

dz) 

= 

ˆ F F 

ˆ 

F F 

 

i j kˆ 

 

 

. 

S 

 

 

y z z x x y 

 

( iˆcos ˆj cos kˆ 

cos ) 

ds 

3 2 1 3 

2 1 

= F 

F F F 

F F 

 

cos cos cos ds 

S y z 

 

 

z x 

 

x y 

 

...(1) 

Let us first prove 

F c 

1 dx 

1 1 

= F 

F 

 

cos cos ds 

S 

 

 

 

z 

y 

 

...(2) 

Let the equation of the surface S be z = g (x, y). The projection of the surface on x – y plane 

is region R. 

F 1 ( x , y , z ) dx 

c 

= F 1 [ x , y , g ( x , y )] dx 

c 

 

= F1 ( x, y, g) 

dx dy [By Green’s Theorem] 

R y 

F1 F1 

g 

= dx dy 

R 

 

y z y 

 

...(3) 

The direction consines of the normal to the surface z = g(x, y) are given by 

cos 

= cos cos 

 

g 

g 

1 

x 

y

Vectors 437 

And dx dy = projection of ds on the xy-plane = ds cos 

Putting the values of ds in R.H.S. of (2) 

F 

z 

F 

y 

 

1 1 

cos cos ds 

S 

 

= 

= 

R 

 

 

 

 

z 

cos y 

 

 

 

 

F1 cos F 

1 dx dy 

= 

R 

F1 F1 

dx dy 

cos cos 

z 

y 

cos 

F1 g 

F1 

 

 

dx dy 

R 

z y y 

 

F1 F1 

g 

= dx dy 

R 

 

y z y 

 

...(4) 

From (3) and (4), we get 

F c 

1 = F1 F1 

 

cos cos ds 

S 

 

z 

y 

 

...(5) 

Similarly, F c 

2 2 2 

= F 

F 

 

cos cos ds 

S 

 

x 

z 

 

 

 

...(6) 

and F c 

3 3 3 

= F 

F 

 

cos cos ds 

S 

 

y 

x 

 

...(7) 

On adding (5), (6) and (7), we get 

( F1 dx F2 dy F3 

dz) 

c 

= 

 

S 

F1 F1 F2 F2 

cos cos cos cos 

z y x z 

F3 F3 

 

cos cos ds Proved. 

y 

x 

 

5.39 ANOTHER METHOD OF PROVING STOKE’S THEOREM 

The circulation of vector F around a closed curve C is equal to the flux of 

the curve of the vector through the surface S bounded by the curve C. 

 

F 

d r 

 

c = curl Fnds curl FdS 

S S 

Proof : The projection of any curved surface over xy-plane can be treated as kernal of the 

surface integral over actual surface 

Now, 

S 

 

 

( F) 

kˆ 

dS 

= ( ) ( ) 

 

= [( iˆ)( F ˆj) ( ˆj)( Fiˆ)] 

dx dy 

 

F i j dx dy 

[ kˆ iˆ 

ˆj] 

 

S 

 

 

F F dx dy 

 

= ( y) ( x) 

S 

S 

 

x 

y 

Fx 

dx Fy 

dy [By Green’s theorem] 

= [ ] 

= 

S 

[ ˆ ˆ ] ( ˆ ˆ 

iFx 

jFy 

idx j dy) 

S 

= F 

. dr 

 

c 

 

curl F ndS ˆ = . d r . 

S 

c F 

where, F = F iˆ F ˆj F kând 

dr dx iˆ dyj ˆ dz kˆ 

x y z 

 

 

Example 85. Evaluate by Strokes theorem ( yz dx zx dy xy dz) 

where C is the curve 

C 

x 2 + y 2 = 1, z = y 2 . (M.D.U., Dec 2009) 

Solution. Here we have 

yz dx zx dy xy dz 

= ( yziˆ zxj ˆ xykˆ 

).( idx ˆ ˆjdy kdz)

438 Vectors 

= Fdx . 

Curl F = 

iˆ 

ˆj kˆ 

 

x y z 

yz zx xy 

= curl F. 

ds 

= (x – x) î + (y – y) ĵ + (z – z) ˆk 

= 0 = 0 Ans. 

Example 86. Using Stoke’s theorem or otherwise, evaluate 

2 2 

[(2 x y) dx yz dy y z dz] 

 

c 

where c is the circle x 2 + y 2 = 1, corresponding to the surface of sphere of unit radius. 


2 2 

Solution. [(2 x y) dx yz dy y z dz] 

c 

2 2 ˆ ˆ 

= [(2 x y) iˆ yz ˆj y z k] ( iˆdx ˆjdy k dz) 

By Stoke’s theorem 

Curl F = 

 

c 

 

 

 

F 

d r = Curl F n ds 

S 

...(1) 

iˆ 

ˆj kˆ 

F 

= 

Putting the value of curl F in (1), we get 

= ˆ 

ˆ 

= kˆ nˆ 

ds 

 

x y z 

2 2 

2x y yz y z 

= (– 2 yz 2 yz) iˆ 

–(0–0) ˆj (0 1) 

kˆ kˆ 

dx dy 

dx dy 

k n = dx dy 

nk ˆ ˆ = Area of the circle = 

ds 

( nk ˆ ˆ 

 

 

) 

 

 

Example 87. Evaluate F . d r, where F ( x, 

y i xj z kand C is the curve of 

C 

intersection of the plane y + z = 2 and the cylinder x 2 + y 2 = 1. (Gujarat, I sem. Jan. 2009) 

 

2 2 

Solution. F. dr curl F . nds ˆ curl (– y iˆ 

x ˆj z kˆ 

 

) nds ˆ 

...(1) 

Normal vector = 

C S S 

2 2 ˆ 

F (x, y, z) = 

Curl F = 

F 

2 2 

, z) – y ˆ ˆ ˆ 

y iˆ xj ˆ z k 

(By Stoke’s Theorem) 

iˆ 

ˆj kˆ 

 

x y z 

2 2 

– y x z 

= iˆ(0 –0)– ˆj (0 –0) kˆ(12 y) (1 

2 y) 

kˆ 

 

= ˆ 

 

ˆ 

ˆ 

i j k ( y z – 2) ˆj kˆ 

 

x y z 

ˆj 

kˆ 

Unit normal vector ˆn = 

2 

dx dy 

ds = 

ˆ . kˆ 

O 

3y + z = 2 

1 

x 2 + y 2 = 1

Vectors 439 

On putting the values of curl F, 

nˆ 

and ds in (1), we get 

= 

= 

= 

 

F 

 

. dr 

 

ˆ ˆ 

= 

ˆ j k dx dy 

(1 2 ) . 

C y k 

S 

2 ˆj 

kˆ 

 

. kˆ 

2 

 

1 

2ydxdy 

(1 2 y) 

dxdy 2 

1 

 

2 1 = (1 2 r sin ) rd d r 

0 

0 

Y 

2 

 

 

2 

1 2 

 

( r 2r sin ) d d r 

0 0 

1 

2 3 

2 

2 

r 

2r 

1 2 

d sin sin d 

2 3 

 

2 3 

 

 

0 0 

 

0 

2 

 

2 2 2 

= – cos – – 0 

2 3 

 

0 3 3 

 

= Ans. 

Example 88. Apply Stoke’s Theorem to find the value of 

 

c 

( ydx z dy xdz) 

where c is the curve of intersection of x 2 + y 2 + z 2 = a 2 and x + z = a. (Nagpur, Summer 2001) 

 

Solution. ( ydx z dy xdz) 

c 

ˆ 

ˆ 

= ( ˆ ˆ ) ( ˆ ˆ 

yi zj xk idx j dy kdz) 

c 

= 

= curl ( yiˆ zj ˆ xkˆ 

) nds ˆ 

 

S 

 

C 

( yiˆ 

zj ˆ xkˆ 

) dr 

(By Stoke’s Theorem) 

ˆ 

ˆ 

= 

ˆ 

 

ˆ 

 

i j k ( yiˆ zj ˆ xk) 

nˆ 

ds 

S 

 

x y z 

= ˆ ˆ ˆ 

( i j k) 

nˆ 

ds 

S 

...(1) 

where S is the circle formed by the intersection of x 2 + y 2 + z 2 = a 2 and x + z = a. 

 

ˆ 

 

ˆ 

ˆ 

 

i j k ( x z a) 

ˆn = | = x y z 

| 

| | 

iˆ 

kˆ 

ˆn = 

2 2 

Putting the vlaue of ˆn in (1), we have 

ˆ ˆ 

= ( ˆ ˆ ˆ 

i k 

i j k) 

ds 

S 

 

2 2 

 

1 1 

= 

ds 

S 

 

2 2 

2 2 

2 2 

a a 

= ds 

2 

S 

 

2 2 2 

Example 89. Directly or by Stoke’s Theorem, evaluate 

= 

iˆ 

kˆ 

1 

1 

 

2 2 

2 2 2 2 a a 

Use 

r R p a 

 

2 2 

Ans. 

ˆ , ˆ ˆ ˆ 

curl vnds v iy jz kx, 

s is 

the surface of the paraboloid z = 1 – x 2 – y 2 , z 3 > 0 and ˆn is the unit vector normal to s. 

s 

 

 

O 

r ddr 

X

440 Vectors 

Solution. 

 

v = 

iˆ 

ˆj kˆ 

iˆ 

ˆj kˆ 

x y z 

y z x 

Obviously ˆn = k ˆ. 

Therefore 

 

( v) 

nˆ 

= (– iˆ 

– ˆj – kˆ). kˆ 

–1 

Hence 

( v) 

nds ˆ = ( 1) dx dy 

S 

= 

S 

 

S 

dx dy 

= – (1) 2 = – . (Area of circle = r 2 ) Ans. 

Example 90. Use Stoke’s Theorem to evaluate 

v , 

c dr 

2 

where v y iˆ xyj ˆ xzkˆ 

, and c 

is the bounding curve of the hemisphere x 2 + y 2 + z 2 = 9, z > 0, oriented in the positive 

direction. 

Solution. By Stoke’s theorem 

 

( v) 

nds ˆ = 

S 

 

v 

c 

 

dr = (curl v) nˆ ds ( v) 

nds 

ˆ 

S S 

iˆ 

ˆj kˆ 

ˆ ˆ 

ˆ 

(0 0) i ( z 0) j ( y 2 y) 

k 

v = 

x y z zj ˆ ykˆ 

2 

y xy xz 

2 2 2 

 

i j k ( x y z 9) 

ˆn = | = x y z 

| 

| | 

= 

2 xiˆ 2 yj ˆ 2 zkˆ xiˆ yj ˆ zkˆ xiˆ yj ˆ zkˆ 

 

 

2 2 2 2 2 2 

4x 4y 4 z x y z 3 

 

ˆ ˆ ˆ 

( v) 

nˆ 

2 

= ( ˆ ˆ xi yj zk yz yz yz 

zj yk) 

 

3 3 3 

ˆ ˆ ˆ 

ˆn 

kds ˆ = dx dy 

xi yj zk . k ˆ 

z 

dx = dx dy ds = dx dy 

3 

3 

ds = 3 dx dy 

z 

 

2yz 

3 

 

dx dy = 2 ydxdy 

3 z 

 

= 

= 2r sin r d dr 

3 

3 

2 r 

0 

0 

 

2 

3 

 

2 sin d r dr 

 

0 0 

= 2( cos ) 

= – 2 (– 1 + 1) 9 = 0 Ans. 

3 

Example 91. Evaluate the surface integral curl F . nˆ 

dS by transforming it into a line 

S 

integral, S being that part of the surface of the paraboloid z = 1 – x 2 – y 2 for which 

 

z0and 

F yiˆ 

zj ˆ xkˆ 

. (K. University, Dec. 2008) 

 

2

Vectors 441 

Solution. 

 

 

F = 

iˆ 

ˆj kˆ 

iˆ 

ˆj kˆ 

x y z 

y z x 

Obviously ˆn = k ˆ. 

Therefore 

 

( F) 

nˆ 

= (– iˆ 

– ˆj – kˆ). kˆ 

–1 

Hence 

( F) 

nˆ 

ds 

= ( 1) dx dy 

S 

 

= 

S 

 

S 

dx dy 

= – (1) 2 = – . (Area of circle = r 2 ) Ans. 

Example 92. Evaluate Fdr 

 

2 2 

by Stoke’s Theorem, where F y iˆ x ˆ j ( x z) 

kˆ 

and 

C 

C is the boundary of triangle with vertices at (0, 0, 0), (1, 0, 0) and (1, 1, 0). 


Solution. We have, curl F = 

= 

iˆ 

ˆj kˆ 

 

x y z 

2 2 

F 

0. iˆ ˆj 2( x y) kˆ 

. 

y x ( x z) 

We observe that z co-ordinate of each vertex of the triangle is zero. 

Therefore, the triangle lies in the xy-plane. 

ˆn = ˆk 

 

curl Fn ˆ [ ˆj 2( x yk ) ˆ]. kˆ 

2( x y). 

In the figure, only xy-plane is considered. 

The equation of the line OB is y = x 

By Stoke’s theorem, we have 

C 

 

Fdr 

= 

 

S 

 

 

(curl Fnˆ 

) ds 

1 x 

= 2( ) 

2 

1 

x y dx dy 

y 

x0 = 2 

y 0 xy 

dx 

0 

2 0 

2 

1 2 

= 2 x 

2 

x 

dx 

0 

2 

= 

1 x 

1 

2 dx = 2 

0 2 

x dx 

3 

x 

= 

0 

3 

x 

1 

0 

= 1 . 

3 

Example 93. Evaluate Fdr 

 

 

by Stoke’s Theorem, where 

2 2 

F ( x y ) iˆ 2 xy ˆj 

C and C 

is the boundary of the rectangle x = a, y = 0 and y = b. (U.P., I Semester, Winter 2002) 

Solution. Since the z co-ordinate of each vertex of the given rectangle is zero, hence the given 

rectangle must lie in the xy-plane. 

Here, the co-ordinates of A, B, C and D are (a, 0), (a, b), (– a, b) and (– a, 0) respectively. 

Curl F = 

iˆ 

ˆj kˆ 

 

= – 4 y k 

x y z 

2 2 

x y 2xy 

0 

Ans.

442 Vectors 

Here, 

nˆ kˆ 

, so by Stoke’s theorem, we’ve 

= 

S 

Fdr 

 

 

C 

= curl ˆ 

S 

( 4 ykˆ)() 

kˆ 

d xdy 

= 

a 

 

2 

y 

= 4 dx 

2 

= 

 

a 

b 

0 

F n ds 

a 

b 

4 

xa y 0 

a 

2 2 

 

2b dx 4ab 

a 

Example 94. Apply Stoke’s Theorem to calculate 

c 

 

ydxdy 

4ydx 2zdy 6ydz 

where c is the curve of intersection of x 2 + y 2 + z 2 = 6 z and z = x + 3. 

Solution. 

 

 

 

F dr = 4 ydx 2z dy 6 ydz 

c c 

= 

F = 

F 

= 

 

c 

(4 yiˆ 2zj ˆ 6 ykˆ) ( idx ˆ ˆjdy kdz ˆ ) 

4 yiˆ 

2 zj ˆ 6 

ykˆ 

iˆ 

ˆj kˆ 

(6 2) iˆ(0 0) ˆj (0 4) kˆ 

x y z 4iˆ 

4kˆ 

4y 2z 6 y 

Ans. 

S is the surface of the circle x 2 + y 2 + z 2 = 6z, z = x + 3, ˆn is normal to the plane x – z + 3 = 0 

 

ˆ ˆ ˆ 

i j k ( x z 3) 

 

ˆn = | = x y z 

| 

| | 

( F) 

nˆ 

= 

ˆ ˆ 

(4 ˆ 4 ˆ i k 

i k) 

= 4 4 = 4 2 

2 2 

= 

iˆkˆ 

iˆkˆ 

 

1 

1 2 

Fdr 

 

= (curl ) ˆ 

c F nds = 4 2 ( dx dz) 

S 

S 

= 4 2 (area of circle) 

Centre of the sphere x 2 + y 2 + (z – 3) 2 = 9, (0, 0, 3) lies on the plane z = x + 3. It means that 

the given circle is a great circle of sphere, where radius of the circle is equal to the radius of the 

sphere. 

Radius of circle = 3, Area = (3) 2 = 9 

( F) 

nˆ 

ds = 4 2(9 ) 36 2 Ans. 

S 

Example 95. Verify Stoke’s Theorem for the function 

F ziˆ x ˆ j ykˆ 

, where C is the unit 

2 2 

circle in xy-plane bounding the hemisphere z = ( 1x y ). (U.P., I Semester Comp. 2002) 

Solution. Here F = ziˆ xj ˆ ykˆ 

. 

...(1) 

Also, r = xiˆ 

yj ˆ zkˆ 

dr = dxiˆ dyj ˆ dz kˆ 

. 

 

F 

dr = z dx + x dy + y dz. 

 

F 

dr = ( zdx x dy ydz). 

C C 

...(2)

Vectors 443 

On the circle C, x 2 + y 2 = 1, z = 0 on the xy-plane. Hence on C, we 

have z = 0 so that dz = 0. Hence (2) reduces to 

F 

dr = xdy . 

C C 

...(3) 

Now the parametric equations of C, i.e., x 2 + y 2 = 1 are 

x = cos , y = sin . ...(4) 

Using (4), (3) reduces to F 

. dr 

 

C 

= 

2 

cos cos d 

= 

0 2 

 

2 

0 

1 cos 2 

2 

1 sin 2 

= 

2 

 

2 

= ...(5) 

0 

Let P(x, y, z) be any point on the surface of the hemisphere x 2 + y 2 + z 2 = 1, O origin is the 

centre of the sphere. 

Radius = OP = 

xiˆ 

yj ˆ zkˆ 

Normal = xiˆ 

yj ˆ zkˆ 

ˆn 

xiˆ 

yj ˆ zkˆ 

= 

xiˆ yj ˆ zkˆ 

2 2 2 

x y z 

(Radius is to tangent i.e. Radius is normal) 

x = sin cos , y = sin sin , z = cos ...(6) 

ˆn = sin cos î + sin sin ĵ + cos ˆk 

iˆ 

ˆj kˆ 

Also, Curl F = / x / y / 

z iˆ 

ˆj kˆ 

...(7) 

z x y 

 

= 

= 

d 

 

Curl Fn ˆ = ( iˆ ˆj kˆ).(sin cos iˆsin sin ˆj sin kˆ) 

= sin cos + sin sin + cos 

/2 2 

Curl Fn ˆ dS = ( ˆ ˆ ˆ 

i j k) 

0 

 

0 

s 

= 

 

. (sin cos î + sin sin ĵ + cos ˆk ) sin d d 

/2 2 

 

sin d (sin cos sin sin cos ) 

d 

0 0 

[ dS = Elementary area on hemisphere = sin d d] 

/2 

2 

sin d 

[sin sin sin ( cos ) cos ] 

0 

0 = 

/2 

(0 0 2 sin cos ) d = 

0 

= – (/2) [– 1 – 1] = . 

From (5) and (8), 

C 

 

 

Fdr = 

/2 

sin 2 d 

= 

0 

 

/2 

0 

 

 

 

sin d 

 

cos 2 

2 

 

 

 

/2 

curl F 

ndS ˆ , which verifies Stokes’s theorem. 

S 

Example 96. Verify Stoke’s theorem for the vector field F (2 x – y) iˆ 

– yz ˆj – y zk over 

0 

2 2 ˆ 

the upper half of the surface x 2 + y 2 + z 2 = 1 bounded by its projection on xy- plane. 


Solution. Let S be the upper half surface of the sphere x 2 + y 2 + z 2 = 1. The boundary C or S 

is a circle in the xy plane of radius unity and centre O. The equation of C are x 2 + y 2 = 1, 

z = 0 whose parametric form is 

x = cos t, y = sin t, z = 0, 0 < t < 2 

 

 

F . dr = 

C 

 

C 

2 2 

[(2 x – y) iˆ – yz ˆj – y zkˆ].[ iˆ dx ˆjdy kdz ˆ ]

444 Vectors 

2 2 

= [(2 x – y) dx – yz dy – y z dz] 

C 

= (2 x – y) dx, 

C 

since on C, z = 0 and 2z = 0 

2 

dx 2 

= (2cos t –sin t) dt (2cos t –sin t)(–sin t) 

dt 

0 dt 

0 

2 2 

2 1–cos 2t 

 

= (– sin 2t sin t) dt – sin 2t dt 

0 

0 

 

 

 

2 

2 

cos 2t t sin 2t 

1 1 

= – – 

2 2 4 

 

 

0 2 2 

iˆ 

ˆj kˆ 

...(1) 

 

Curl F = 

 

x y z = (– 2yz 2 yz) iˆ 

(0–0) ˆj (01) 

kˆ kˆ 

2 x – y 

2 

– yz 

2 

– y z 

 

Z 

Curl F . nˆ 

= kˆ. nˆ 

nk ˆ. 

ˆ 

 

Curl F. 

nds ˆ = ˆ. ˆ ˆ. ˆ dx dy 

. . 

S nkds nk 

S R ˆ ˆ 

Where R is the projection of S on xy-plane. 

O 

Y 

= 

2 

1 1– x 

dx dy = 

2 

–1 – 1– x 

 

1 2 1 2 

2 1– x dx 4 1– x dx 

–1 0 

1 

2 –1 

x 

1 1 

 

= 4 1– x sin x 4 . 

2 2 

 

0 2 2 

 

 

From (1) and (2), we have 

 

 

F . dr = Curl F . nds ˆ which is the Stoke's theorem. 

C 

 

Example 97. Verify Stoke’s Theorem for 2 ˆ ˆ 

2 

F ( x y 4) i 3 xyj (2 xz z ) kˆ 

over the surface of hemisphere x 2 + y 2 + z 2 = 16 above the xy-plane. 

 

Solution. Fdr , where c is the boundary of the circle x 2 + y 2 + z 2 = 16 

c 

(bounding the hemispherical surface) 

2 2 ˆ 

= [( x y 4) iˆ 3 xyj ˆ (2 xz z ) k]( idx ˆ ˆjdy) 

Putting 

 

 

c 

2 

= [( x y 4) dx 3 xy dy)] 

c 

x = 4 cos , y = 4 sin , dx = – 4 sin d , dy = 4 cos d 

= 

 

2 

2 2 

0 

 

[(16 cos 4sin 4) ( 4sin d ) (192 sin cos d )] 

2 2 2 2 

= 16 [ 4cos sin sin sin 12 sin cos ] d 

 

 

0 

2 

2 2 

= 16 (8 sin cos sin sin ) d 

= 

= 

0 

 

2 

16 sin d 

 

0 

2 

 

2 2 

= 

0 

16 4 sin d 

 

To evaluate surface integral 

F 

= 

1 

 

64 = – 16 . 

22 

iˆ 

ˆj kˆ 

 

x y z 

X 

 

 

 

 

 

2 2 

x y 4 3xy 2 xz z 

 

 

2 

0 

2 

0 

C 

...(2) 

Ans. 

n 

sin cos d0 

 

 

 

n 

cos sin d 

0

Vectors 445 

= (0 – 0) iˆ – (2 z – 0) ˆj (3 y – 1) kˆ – 2 zj ˆ (3 y – 1) kˆ 

 

ˆ ˆ ˆ 2 2 2 

 

i j k ( x y z 16) 

ˆn = | = x y z 

| 

| | 

2xiˆ 

2yj ˆ 2zkˆ 

xiˆ 

yj ˆ zkˆ 

xiˆ 

yj ˆ zkˆ 

= 

= 

= 

2 2 2 2 2 2 

4x 4y 4z 

x y z 4 

 

ˆ ˆ ˆ 

( F) 

nˆ 

= [– 2 ˆ (3 – 1) ˆ xi yj zk 

zj y k] 

 

2 yz (3y 1) z 

= 

4 

4 

ˆk n 

xiˆ 

yj ˆ zkˆ 

z 

ds = dx dy 

. k ds = dx dy ds = dx dy 

4 

4 

 

ds = 4 dx dy 

z 

2 (3 1) 4 

( F) 

nˆ 

ds yz y z dx dy 

 

 

4 z 

= [ 2 y (3 y 1)] dx dy = ( y 1) dxdy 

On putting x = r cos , y = r sin , dx dy = r d dr, we get 

= 

= 

= ( r sin 1) r d dr 

2 

3 2 

4 

 

 

2 

d ( r sin r) 

dr 

= r 

r 

d 

 

sin 

3 2 = 64 

d 

sin 8 

0 

 

3 

0 0 

 

2 

64 64 64 

= cos 8 

= 16 

= – 16 

3 3 3 

0 

The line integral is equal to the surface integral, hence Stoke’s Theorem is verified. Proved. 

 

2 2 

Example 98. Verify Stoke’s theorem for a vector field defined by F ( x – y ) iˆ 2xy ˆj 

in 

the rectangular in xy-plane bounded by lines x = 0, x = a, y = 0, y = b. 


Solution. Here we have to verify Stoke’s theorem F 

. dr 

 

= ( ). ˆ 

C F 

nds 

S 

Where ‘C’ be the boundary of rectangle (ABCD) and S be the surface enclosed by curve C. 

2 

F 2 2 

= ( x – y ) iˆ (2 xy) 

ˆj 

 

F . dr = 

2 2 

[( x – y ) iˆ 2 xyj ˆ].[ idx ˆ ˆj dy] 

 

F . dr = (x 

2 

+ y 2 ) dx + 2xy dy ...(1) 

Now, F 

. dr 

 

 

= F . dr F . dr F . dr F . dr 

C 

...(2) 

OA AB BC CO 

 

Along OA, put y = 0 so that k dy = 0 in (1) and F . dr = x 2 dx, 

Where x is from 0 to a. 

3 

a 

a 

2 

F 

. dr 

 

3 

x dx 

x a 

OA = 

0 3 3 

 

Along AB, put x = a so that dx = 0 in (1), we get F . 

Where y is from 0 to b. 

 

b 

0 

0 

 

d r 

= 2ay dy 

...(3) 

2 2 

F . dr 2aydy 

= [ ay ] b 

0 

ab 

...(4) 

AB

446 Vectors 

Along BC, put y = b and dy = 0 in (1) we get F . dr = (x 2 – b 2 ) dx, 

where x is from a to 0. 

F 

 

BC 

. dr = 

 

0 

3 

0 

3 

2 2 x 2 

– a 2 

Along CO, put x = 0 and dx = 0 in (1), we get F . dr 0 

( x – b ) dx – bx 

ba 

a 

3 3 

...(5) 

 

 

F 

. dr 

 

= 0 ...(6) 

CO 

Putting the values of integrals (3), (4), (5) and (6) in (2), 

we get 

 

 

F . dr = 

C 

3 3 

2 a 2 2 

a 

ab – ab 0 2ab 

...(7) 

3 3 

Now we have to evaluate R.H.S. of Stoke’s Theorem i.e. 

We have, 

F 

= 

iˆ 

ˆj kˆ 

 

x y z 

2 2 

x – y 2xy 

0 

 

S 

a 

 

( F). 

nds ˆ 

(2y 2 y) kˆ 

4ykˆ 

Also the unit vector normal to the surface S in outward direction is ˆn 

k 

(z-axis is normal to surface S) 

Also in xy-plane ds = dx dy 

 

( F). nˆ 

. ds = 4 ˆ. ˆ 4 . 

S yk kdxdy ydx dy 

R R 

Where R be the region of the surface S. 

Consider a strip parallel to y-axis. This strip starts on line y = 0 (i.e. x-axis) and end on the line 

y = b, We move this strip from x = 0 (y-axis) to x = a to cover complete region R. 

 

 

( F). nˆ 

. ds = 

S 

 

From (7) and (8), we get 

 

 

= 

 

 

ydy 

 

dx y dx 

 

a b 2 

4 

a [2 ] 

b 

0 0 0 

0 

 

a 2 2 a 2 

b dx b x 

0 

ab 

...(8) 

0 2 2 [ ] 2 

F . dr = ( ). ˆ 

C F nds and hence the Stoke’s theorem is verified. 

S 

Example 99. Verify Stoke’s Theorem for the function 

 

F = xi 

2ˆ – xyj ˆ 

integrated round the square in the plane z = 0 and bounded by the lines 

x = 0, y = 0, x = a, y = a. 

Solution. We have, F = xi 

2ˆ – xyj ˆ 

 

F = 

iˆ 

ˆj kˆ 

 

x y z 

2 

x = 0 

Y 

y = b 

C 

B 

(a, b) 

x = a 

x xy 0 

= (0 –0) iˆ 

–(0–0) ˆj (– y –0) kˆ – ykˆ 

( ˆn to xy plane i.e. ˆk ) 

O 

y = 0 

A 

X

Vectors 447 

( F) 

nˆ 

ds = ( yk) 

kdxdy 

S 

S 

 

= 

 

To obtain line integral 

a 

a 

dx 

ydy = 

0 0 

a 

a 

2 

y 

dx 

 

 

 

2 

= 

0 0 

2 

a a 

( x) 0 = 

2 

 

F dr 2 

= ˆ ˆ ˆ ˆ 

2 

( x i xyj) ( idx j dy) 

= ( x dx xydy) 

C 

C 

C 

where c is the path OABCO as shown in the figure. 

Also, 

 

F dr = 

C 

 

Fdr = 

OABCO 

Along OA, y = 0, dy = 0 

F 

dr 

 

= 

OA 

 

2 

( xdx 

xydy ) 

OA 

a 

3 

2 x 

= xdx = = 

0 

3 0 

Along AB, x = a, dx = 0 

Fdr 

 

2 

= ( x dx xyd y) 

AB AB 

= 

a 

aydy = 

Along BC, y = a, dy = 0 

 

 

0 

 

Fdr Fdr Fdr F 

dr 

OA AB BC CO 

3 

a 

...(1) 

2 

...(2) 

a 

3 

a 

3 

2 

y 

a 

 

 

2 

F dr 

2 

= ( xdx xydy) 

= 

BC 

BC 

Along CO, x = 0, dx = 0 

 

a 

0 

= 

3 

a 

 

2 

0 2 

xdx 

a 

= 

Fdr 

2 

= ( ) 

CO xdx xydy = 0 

CO 

Putting the values of these integrals in (2), we have 

Fdr 

 

= 

C 

From (1) and (3), 

3 3 3 

a a a 

0 = 

3 2 3 

( F) 

nˆ 

ds = 

Hence, Stoke’s Theorem is verified. 

S 

 

 

 

C 

 

3 

 

3 

x 

 

 

0 

3 a 

= 

3 

a 

 

3 

a 

...(3) 

2 

 

F dr 

Ans. 

Example 100. Verify Stoke’s Theorem for F 

 

= (x + y) î + (2x – z) ĵ + (y + z) ˆk for the 

surface of a triangular lamina with vertices (2, 0, 0), (0, 3, 0) and (0, 0, 6). 

(Nagpur University 2004, K. U. Dec. 2009, 2008, A.M.I.E.T.E., Summer 2000) 

Solution. Here the path of integration c consists of the straight lines AB, BC, CA where the 

co-ordinates of A, B, C and (2, 0, 0), (0, 3, 0) and (0, 0, 6) respectively. Let S be the plane 

surface of triangle ABC bounded by C. Let ˆn be unit normal vector to surface S. Then by 

Stoke’s Theorem, we must have 

Fdr 

 

= 

c 

curl Fnds ˆ 

...(1) 

s 

 

 

line Eq. of Lower Upper 

line limit limit 

OA y = 0 dy = 0 x = 0 x = a 

AB x = a dx = 0 y = 0 y = a 

BC y = a dy= 0 x = a x = 0 

CO x = 0 dx = 0 y = a y = 0

448 Vectors 

c 

L.H.S. of (1)= Fdr 

 

= Fdr F dr F dr 

ABC AB 

BC CA 

x y 

Along line AB, z = 0, equation of AB is = 1 2 3 

y = 3 3 

(2 x), 

dy = 

2 

2 dx 

At A, x = 2, At B, x = 0, r = xiˆ 

yj ˆ 

 

 

F dr = 

AB 

 

 

AB 

[( x y) iˆ 2 xj ˆ ykˆ 

]( idx ˆ ˆjdy) 

= ( x y) dx 2xdy 

= 

 

AB 

AB 

3x 

3 

x 3 dx 2x 

dx 

2 2 

2 

0 

7x 

7x 

 

= 3 dx 

3x 

2 

 

2 

4 

 

= (7 – 6) = + 1 

y z 

Along line BC, x = 0, Equation of BC is = 1 or z = 6 – 2y, dz = – 2dy 

3 6 

At B, y = 3, At C, y = 0, r = 

 

 

yj ˆ zkˆ 

F dr = [ ( ) ] ( ) 

BC 

yi zj y z k jdy kdz = ( ) 

BC zdy y z dz 

BC 

0 

= ( 6 2 y) dy ( y 6 2 y)( 2 dy) 

3 

0 2 0 

= (4y 18) dy (2y 18 y) 

3 

3 = 36 

x z 

Along line CA, y = 0, Eq. of CA, = 1 or z = 6 – 3x, dz = – 3dx 

2 6 

At C, x = 0, at A, x = 2, r = xiˆ 

zkˆ 

 

 

F dr = [ xiˆ (2 x z) ˆj zkˆ][ dxiˆ 

dzkˆ] 

CA = ( xdx zdz) 

CA 

= 

line Eq. of Lower Upper 

line limit limit 

AB 

x y 3 At A At B 

1 

dy – dx 

2 3 2 x 2 x 0 

z = 0 

BC 

y z 

At B At C 

1 

dz = – 2dy 

3 6 

y 3 y 0 

x = 0 

CA 

x z 

At C At A 

1 

dz = – 3dx 

2 6 

x 0 x 2 

y = 0 

CA 

2 

xdx (6 3 x )( 3 dx ) = 

0 

2 

0 

0 

2 

 

(10 x 18) dx 2 2 

= [5x 

18 x] 

0 = – 16

Vectors 449 

c 

L.H.S. of (1) = 

Fdr 

 

 

ABC 

= 

 

Fdr F dr F dr 

= 1 + 36 – 16 = 21 ...(2) 

AB BC CA 

 

Curl F = F = 

ˆ 

 

ˆ 

ˆ 

i j k [( x y) iˆ (2 x z) ˆj ( y z) k] 

x y z 

iˆ 

ˆj kˆ 

= 

 

x y z 

x y 2x z y z 

x y z 

Equation of the plane of ABC is = 1 

2 3 6 

Normal to the plane ABC is 

(1 1) iˆ(0 0) ˆj (2 1) kˆ 

2iˆ 

kˆ 

 

ˆ 

= 

ˆ 

 

ˆ 

x y z 

i j k 1 

= 

x y z2 3 6 

iˆ 

ˆj kˆ 

 

Unit Normal Vector = 

2 3 6 

1 1 1 

 

4 9 36 

1 

ˆn = (3iˆ 

2 ˆj kˆ 

) 

14 

i ˆ ˆ ˆ 

 

j 

k 

2 3 6 

 

R.H.S. of (1) = curl Fnds 

= ˆ ˆ 1 

ˆ ˆ ˆ dx dy 

(2 ) (3 2 ) 

s i k i j k 

s 

4 1 

(3iˆ 

2 ˆj kˆ). 

kˆ 

14 

(6 1) dx dy 

= = 7 

s 

14 1 dx dy = 7 Area of OAB 

14 

1 

 

= 7 

23 

= 21 ...(3) 

2 

 

with the help of (2) and (3) we find (1) is true and so Stoke’s Theorem is verified. 

Example 101. Verify Stoke’s Theorem for 

 

F 

= (y – z + 2) î + (yz + 4) ĵ – (xz) ˆk 

over the surface of a cube x = 0, y = 0, z = 0, x = 2, y = 2, z = 2 above the XOY plane 

(open the bottom). 

Solution. Consider the surface of the cube as shown in the figure. Bounding path is OABCO 

shown by arrows. 

 

Fdr = [( y z 2) iˆ ( yz 4) ˆj ( xz) kˆ] ( idx ˆ ˆjdy kdz ˆ ) 

 

 

= ( y z 2) dx ( yz 4) dy xzdz 

c 

 

 

Fdr = Fdr Fdr Fdr Fdr 

c 

OA AB BC CO 

(1) Along OA, y = 0, dy = 0, z = 0, dz = 0 

...(1)

450 Vectors 

Line Equ. Lower Upper Fdr . 

1 OA 

2 AB 

3 BC 

4 CO 

of line limit limit 

y 0 dy 0 

z 0 dz 0 

x = 0 x = 2 2 dx 

x 2 dx 0 

z 0 dz 0 

y = 0 y = 2 4 dy 

y 2 dy 0 

z 0 dz 0 

x = 2 x = 0 4 dx 

x 0 dx 0 

z 0 dz 0 

y = 2 y = 0 4 dy 

Fdr = 

OA 

(2) Along AB, x = 2, dx = 0, z = 0, dz = 0 

AB 

 

 

 

 

Fdr = 

(3) Along BC, y = 2, dy = 0, z = 0, dz = 0 

F dr = 

BC 

(4) Along CO, x = 0, dx = 0, z = 0, dz = 0 

CO 

 

 

 

 

2 

2 

2 dx [2 x] 

0 = 4 

0 

2 

2 

4dy 

4( y) 

0 = 8 

0 

2 

0 

(2 0 2) dx (4 x) 

2 = – 8 

0 

Fdr = ( y 0 2) 0 (0 4) dy 0 

0 

= 4 dy 4( y) 2 = – 8 

On putting the values of these integrals in (1), we get 

Fdr = 4 + 8 – 8 – 8 = – 4 

c 

 

 

To obtain surface integral 

iˆ 

ˆj kˆ 

F 

= 

 

x y z 

y z 2 yz 4 xz 

= (0 – y) î – (– z + 1) ĵ + (0 – 1) ˆk = – y î + (z – 1) ĵ – ˆk 

Here we have to integrate over the five surfaces, ABDE, OCGF, BCGD, OAEF, DEFG. 

Over the surface ABDE (x = 2), ˆn = i, ds = dy dz 

 

( F) 

nds ˆ = [ yi ( z 1) j k] 

idx dz ydydz 

 

2 

= 

 

R 

( , ) 

3 ( , , ) z f x y 

z f ( x, y) 

F x y z dx dy 

1

Vectors 451 

Surface Outward normal 

ds 

1 ABDE i dy dz x = 2 

2 OCGF – i dy dz x = 0 

3 BCGD j dx dz y = 2 

4 OAEF – j dx dz y = 0 

5 DEFG k dx dy z = 2 

= 

2 2 2 

y 

2 

ydydz [ z] 4 

0 

2 

0 0 0 

2 

Over the surface OCGF (x = 0), ˆn = – i, ds = dy dz 

 

( F) 

nds ˆ = [ ˆ ( 1) ˆ ˆ] ( ˆ 

yi z j k i) 

dy dz 

= 

2 2 2 

y 

 

ydydz y dy dz 2 

4 

2 

 

0 0 0 

2 

(3) Over the surface BCGD, (y = 2), ˆn = j, ds = dx dz 

 

( F) 

nds ˆ = [ yiˆ ( z 1) ˆj kˆ 

] ˆ 

j dx dz 

= 

= ( z 1) 

dxdz 

2 2 

dx ( z 1) dz = 

0 0 

( x) 

2 

0 

 

2 

z 

 

2 

2 

 

z 

 

 

0 

= 0 

(4) Over the surface OAEF, (y = 0), ˆn = – ĵ , ds = dx dz 

 

( F ) nˆ 

ds = ˆ 

 

[ yiˆ ( z 1) ˆj k]( ˆj) 

dx dz 

= ( z 1) 

dxdz = – 

2 2 

dx ( z 1) dz = 

0 0 

(5) Over the surface DEFG, (z = 2), ˆn = k, ds = dx dy 

( x) 

2 

0 

 

2 

z 

 

2 

2 

 

z 

 

 

0 

= 0 

 

( F) 

nds ˆ = [ yi ˆ ( z 1) ˆ j k ˆ] 

k ˆ 

dx dy = – dx dy 

= – 

2 2 

dx 

dy 2 2 

= [ x] [ y] 

= – 4 

0 0 

0 0 

Total surface integral = – 4 + 4 + 0 + 0 – 4 = – 4 

Thus 

curl F nds ˆ = 

S 

 

 

F dr = – 4 

c 

 

which verifies Stoke’s Theorem. 

 

Ans.

452 Vectors 

1. Use the Stoke’s Theorem to evaluate 

EXERCISE 5.14 

 

2 

ydx 

xydy xzdz, 

C 

where C is the bounding curve of the hemisphere x 2 + y 2 + z 2 = 1, z 0, oriented in the positive 

direction. Ans. 0 

2. Evaluate 

(curl F) ndA ˆ , using the Stoke’s Theorem, where F yiˆ 

zj ˆ xkˆ 

and s is the paraboloid 

s 

z = f (x, y) = 1 – x 2 – y 2 , z 0. 

Ans. 

 

2 2 2 

3. Evaluate the integral for ydx z dy xdz, where C is the triangular closed path joining the points 

C 

(0, 0, 0), (0, a, 0) and (0, 0, a) by transforming the integral to surface integral using Stoke’s Theorem. 

 

2 

4. Verify Stoke’s Theorem for A 3 yiˆ 

xzj ˆ yz kˆ 

, where S is the surface of the paraboloid 2z = x 

2 

+ y 2 

bounded by z = 2 and c is its boundary traversed in the clockwise direction. 

Ans. – 20 

5. Evaluate 

F 

 

d R where 

C 

Ans. 

3 

a 

. 

3 

 

ˆ 3 

F yi xz ˆ j zy 

3ˆ k, 

C is the circl x 2 + y2 = 4, z = 1.5 Ans. 19 2 

6. If S is the surface of the sphere x 2 + y 2 + z 2 

= 9. Prove that curl F ds = 0. 

S 

7. Verify Stoke’s Theorem for the vector field 

 

F (2 y z) iˆ 

( x – z) ˆj ( y – x) 

kˆ 

over the portion of the plane x + y + z = 1 cut off by the co-ordinate planes. 

8. Evaluate 

dr by Stoke’s Theorem for F yziˆ zx ˆ j xyk and C is the curve of intersection of 

c 

x 2 + y 2 = 1 and y = z 2 . Ans. 0 

9. 

 

If ˆ 3 ˆ 2 

F ( x – z) i ( x yz) j 3xy kˆ 

and S is the surface of the cone z = a – 2 2 

( x y ) above the 

xy-plane, show that 

 

curl FdS = 3 a 4 / 4. 

s 

 

10. If F 3yiˆ 

xyj ˆ yz2kˆ 

and S is the surface of the paraboloid 2z = x 2 + y 2 bounded by z = 2, show by 

 

using Stoke’s Theorem that ( F) 

dS = 20 . 

s 

 

2 2 2 2 2 2 2 2 2 

11. If F ( y z – x ) iˆ 

( z x – y ) ˆj ( x y – z ) kˆ 

, evaluate curl F nds ˆ integrated over 

the portion of the surface x 2 + y 2 – 2ax + az = 0 above the plane z = 0 and verify Stoke’s Theorem; where 

ˆn is unit vector normal to the surface. (A.M.I.E.T.E., Winter 20002) Ans. 2 a 3 

where C is the boundary of 

C 

12. Evaluate by using Stoke’s Theorem sin zdx cos x dy sin 

ydz 

rectangle 0 x, 0 y1, z 3 . (AMIETE, June 2010) 

5.40 GAUSS’S THEOREM OF DIVERGENCE 

(Relation between surface integral and volume integral) 

(U.P., Ist Semester, Jan., 2011, Dec, 2006) 

Statement. The surface integral of the normal component of a vector function F taken around 

a closed surface S is equal to the integral of the divergence of F taken over the volume V enclosed 

by the surface S. 

Mathematically 

 

S 

 

F. 

nˆ 

ds 

 

V 

 

div Fdw

Vectors 453 

 

Proof. Let F Fi ˆ ˆ ˆ 

1 F2 j F3 k. 

 

Putting the values of F, 

nˆ 

in the statement of the divergence theorem, we have 

Fi ˆ ˆ 

1 F ˆ 2 j F3 k 

nds ˆ 

S 

= ˆ 

 

ˆ k ˆ 

( ˆ 

V F 1 F 2 

x y z 

ˆ F ˆ 

3 ) . 

1 2 3 

= F 

F 

F 

 

dx dy dz 

V 

 

x y z 

 

We require to prove (1). 

...(1) 

F3 

Let us first evaluate dx dy dz . 

V z 

V 

 

z 

F3 

dx dy dz 

= 

z f2 ( x, y) 

F3 

 

R 

dz dx dy 

z 

f1( x, y) 

z 

 

 

 

[ F ( x, y, f ) F ( x, y, f )] dx dy 

...(2) 

= 

R 

3 2 3 1 

For the upper part of the surface i.e. S 2 

, we have 

dx dy = ds 2 

cos r 2 

= ˆn 2 

. ˆk ds 2 

Again for the lower part of the surface i.e. S 1 

, we have, 

R 

dx dy = – cos r 1 

, ds 1 

= ˆn 1 

. ˆk ds 1 

F3 ( x, y, f2) 

dx dy ˆ ˆ 

F n kds 

S 

= 3 2 2 

= 3 1 1 

and F3 ( x, y, f1) 

dx dy ˆ ˆ 

R F n kds 

S1 

Putting these values in (2), we have 

F3 

dv F nˆ 

kds ˆ F nˆ 

kˆ 

ds 

V z 

S2 S1 

Similarly, it can be shown that 

F2 

dv 

ˆ 

V 

 

ˆ 

y 

F n 

jds 

S 

...(4) 

= 2 

2 

= 3 2 2 3 1 1 = 3 

F1 

dv = 

ˆ 

V 

1 

ˆ 

x 

F ni 

ds 

S 

...(5) 

Adding (3), (4) & (5), we have 

 

V 

F1 F2 

F3 

 

dv 

x y z 

 

= ( Fi ˆ ˆ ˆ 

1 F2 j F3 k) 

nˆ 

ds 

 

( F) 

dv 

V 

S 

= ˆ 

F nds 

Proved. 

S 

Example 102. State Gauss’s Divergence theorem 

 

ˆ ˆ 

F n 

kds ...(3) 

S 

F . nds ˆ Div Fdv 

S 

where S is the 

 

surface of the sphere x 2 + y 2 + z 2 

= 16 and F 3xiˆ 

4y ˆj 5 zkˆ 

. 

(Nagpur University, Winter 2004) 

Solution. Statement of Gauss’s Divergence theorem is given in Art 24.8 on page 597. 

Thus by Gauss’s divergence theorem, 

 

= 

. Here F 3xiˆ 

4y ˆj 5zkˆ 

F . nds ˆ 

S 

v 

 

Fdv

454 Vectors 

. F 

= 

Putting the value of . F, we get 


 

 

ˆ 

 

ˆ 

ˆ 

i j k .(3xiˆ 4yj ˆ 5 zkˆ 

) 

x y z 

. F 

= 3 + 4 + 5 = 14 

= 14 . 

v 

F . nds ˆ 

S 

 

S 

dv 

where v is volume of a sphere 

= 14 v 

4 3 3584 

= 14 (4) 

 

3 3 

Ans. 

 

ˆ 2 

F . nds ˆ where F 4 xzi – y ˆj yz kˆ 

and S is the surface of the 

cube bounded by x = 0, x = 1, y = 0, y = 1, z = 0, z = 1. 

(U.P., Ist Semester, 2009, Nagpur University, Winter 2003) 

Solution. By Divergence theorem, 

 

 

F . nds ˆ = ( . F) 

dv 

S 

 

v 

= 

= 

 

v 

v 

 

 

 

ˆ 

 

ˆ 

ˆ 

ˆ 2 

i j k .(4 xzi – y ˆj yz kˆ 

 

) dv 

x y z 

2 

(4 xz) (– y ) ( yz) 

dxdydz 

x y z 

= (4 z –2 y y) 

dxdydz 

= 

= 

= 

v 

 

v 

2 

1 14z 

 

(4 z – y) dx dy dz – yz dx dy 

0 

0 

2 

 

1 1 2 1 

1 1 

0 0 

0 

0 0 

 

(2 z – yz) dxdy (2 – y) 

dx dy 

1 

2 

1 

 

1 

y 3 

2 y – dx dx 

 

2 2 

= 

0 0 

0 

Note: This question is directly solved as on example 14 on Page 574. 

1 

0 

3 1 3 3 

[ x ] 0 (1) Ans. 

2 2 2 

 

2 

Example 104. Find Fn ˆ ds, 

where F (2x 3) z iˆ 

– ( xz y) ˆj ( y 2 z) 

kˆ 

and S is 

the surface of the sphere having centre (3, – 1, 2) and radius 3. 

(AMIETE, Dec. 2010, U.P., I Semester, Winter 2005, 2000) 

Solution. Let V be the volume enclosed by the surface S. 

By Divergence theorem, we’ve 

 

 

 

 

Fnˆ 

ds = div Fdv. 

S V 

Now, div F 2 

= (2x 3) z [ ( xz y)] ( y 2 z) 

= 2 – 1 + 2 = 3 

x y z 

 

 

= 3 

Fnˆ 

ds 

S dv = 3 

V dv = 3V. 

V 

Again V is the volume of a sphere of radius 3. Therefore 

4 3 4 3 

V = r = (3) = 36 . 

3 3 

 

Fn ˆ ds = 3V = 3 × 36 = 108 Ans. 

S

Vectors 455 

Example 105. Use Divergence Theorem to evaluate 

A 

S ds , 

where 3 3 

A x iˆ 

y ˆj z 

3ˆ k and S is the surface of the sphere x2 + y2 + z2 = a2 . 

(AMIETE, Dec. 2009) 

Solution. 

= 

 

A 

 

S ds = div AdV 

v 

 

ˆ 

 

ˆ 

ˆ 3 ˆ 3ˆ 

3 

i j k ( x i y j z kˆ 

) dV 

x y z 

v 

2 2 2 

(3 x 3y 3 z ) dV = 

v 

2 2 2 

= 

3 ( x y z ) dV 

v 

On putting x = r sin cos , y = r sin sin , z = r cos , we get 

3 r ( r sin dr dd) 

= 3 × 8 

v 

= 

2 2 

 

 

2 2 

 

d sin d 

r dr 

0 0 

5 

a 

5 5 

 

= 24 ( ) 

2 

( cos ) 

2 

r a 

12a 

 

0 0 

 

= 24 ( 0 1) 

 

5 2 5 5 

Ans. 

 

 

 

0 

Example 106. Use divergence Theorem to show that 

2 2 2 

( x y z ) d 

s = 6 V 

S 

where S is any closed surface enclosing volume V. (U.P., I Semester, Winter 2002) 

 

Solution. Here (x 2 + y 2 + z 2 ˆ 2 2 2 

) = 

ˆ 

 

i ˆ 

 

j k ( x y z ) 

x y z 

= 2 xiˆ 2 yj ˆ 2 zkˆ 2 ( xiˆ yj ˆ zkˆ) 

 

2 2 2 

( x y z ) ds 

= 

S 

 

S 

2 2 2 

ˆ 

( x y z ) nds 

ˆn being outward drawn unit normal vector to S 

= 2( xiˆ yj ˆ zkˆ 

) nds ˆ 

 

S 

a 

0 

4 

= 2 ( ˆ ˆ ˆ 

div xi yj zk) 

dv 

...(1) 

V 

(By Divergence Theorem) 

(V being volume enclosed by S) 

Now, 

 

div. ( xiˆ 

yj ˆ zkˆ 

ˆ 

ˆ 

) = 

ˆ 

 

ˆ 

 

i j k ( xiˆ y ˆj zk) 

x y z 

x y z 

= 

 

 

 

x y z 

= 3 ...(2) 

From (1) & (2), we have 

2 2 2 

( y z ) dS dv dv = 6 V 

V 

Proved. 

= 2 3 = 6 

V 

Example 107. Evaluate ˆ ˆ 

( y z i z x j z y k ) n ˆ dS , where S is the part of the sphere 

S 

x 2 + y 2 + z 2 = 1 above the xy-plane and bounded by this plane. 

Solution. Let V be the volume enclosed by the surface S. Then by divergence Theorem, we 

have 

2 2 2 2 2 

( y z i ˆ z x ˆ j z y 2ˆ k ) n ˆ dS 

2 2 2 2 2 

S 

= div ( y z iˆ 

z x ˆj z yk 

2ˆ) 

dV 

= 

 

x y z 

2 2 2 2 2 2ˆ 

 

 

2 2 2 2 2 2 

( y z ) ( z x ) ( z y ) dV 

V 

 

 

2 2 2 

2 

V V 

V 

 

 

zy dV zy dV

456 Vectors 

Changing to spherical polar coordinates by putting 

x = r sin cos , y= r sin sin , z = r cos , dV = r 2 sin dr d d 

To cover V, the limits of r will be 0 to 1, those of will be 0 to 2 

and those of will be 0 to 

2. 

 

2 

2 

dV = 

V zy 

= 

= 

= 

2 /2 1 2 2 2 2 

 

2 ( r cos )( r sin sin ) r sin drd d 

0 0 0 

2 /2 1 5 3 2 

 

2 r sin cos sin 

drd d 

 

0 0 0 

 

6 

1 

2 2 

3 2 

r 

 

0 

 

0 

6 0 

2 sin cos sin 

d d 

2 2 

= 

2 

2 

sin 

6 0 4.2 d 

1 

12 

2 

2 

sin d 

= 

0 

12 

Example 108. Use Divergence Theorem to evaluate FdS 

2 

where F 4 xiˆ 

–2y ˆj z 

2ˆ k 

S 

and S is the surface bounding the region x 2 + y 2 = 4, z = 0 and z = 3. 

(A.M.I.E.T.E., Summer 2003, 2001) 

Solution. By Divergence Theorem, 

FdS 

 

= div FdV 

S V = 

 

 

V 

 

ˆ 

 

ˆ 

ˆ 

ˆ 2ˆ 

2 

i j k (4 xi 2 y j z kˆ 

) dV 

x y z 

= (4 4y 

2 z) 

dx dy dz 

= 

V 

3 

dx dy (4 4y 2 z) 

dz = 

= (12 12y 

9) 

dxdy 

Let us put x = r cos , y = r sin 

= (21 12r sin ) 

r d 

dr 

= 

0 

= (21 12 ) 

= 

2 

2 

2 

2 2 

21r 

3 

 

d 4r 

sin 

2 

0 

 

= 

0 0 

 

0 

 

 

2 2 

0 

 

 

2 3 

dx dy [4z 4 yz z ] 0 

y dxdy 

d (21r 12r sin ) 

dr 

 

 

d (42 32 sin ) (42 32 cos ) 

2 

0 

Ans. 

= 84 + 32 – 32 = 84 Ans. 

^ 

Example 109. Apply the Divergence Theorem to compute u nds, where s is the surface of 

the cylinder x 2 + y 2 = a 2 bounded by the planes z = 0, z = b and where u ix ˆ – ˆjy kz ˆ . 

Solution. By Gauss’s Divergence Theorem 

u nds 

 

ˆ = ( u) 

dv 

V 

 

ˆ 

ˆ 

= 

ˆ 

 

ˆ 

 

i j k ( ix ˆ ˆ 

 

jy kzdv ) 

V 

 

x y z 

= x y z 

dv 

V 

 

x y z 

= 111 

dv 

V 

= dv dx dy dz 

V = Volume of the cylinder = a 2 b Ans. 

= 

V

Vectors 457 

Example 110. Apply Divergence Theorem to evaluate 

F . ˆ , where 

V 

F 

4x 3 iˆ x 2 y ˆj x 2 zk ˆ and S is the surface of the cylinder x 2 + y 2 = a 2 bounded by the 

planes z = 0 and z = b. 


(U.P. Ist Semester, Dec. 2006) 

F 

4x 3 iˆ 

x 2 y ˆj x 2 zk ˆ 

 

ˆ 

3 2 2 ˆ 

div F = 

ˆ 

 

ˆ 

 

i j k (4 x iˆ x yj ˆ x zk) 

x y z 

3 2 2 

= (4 x ) ( x y) ( x z) 

x y z 

= 12x 2 – x 2 + x 2 = 12 x 2 

Now, 

V 

 

div FdV 

= 

2 2 

12 a a 

x b 

xay a 

2 

x 

2 

z 0 

x 

2 

dzdydx 

a a 

2 

x 

2 

2 b 

xa y a 

2 

x 

2 

= 

= 12 x ( z) 

0 dydx 

a 

a 

 

a 2 2 

2 a 

x 

a a 

2 

x 

2 

12 b x ( y) 

dx 

2 2 2 

2 2 2 

= 12 b x .2 a x dx = 24 b 

x a x dx 

= 

= 

 

a 

2 2 2 

48 b x a x dx 

 

0 

/2 2 2 

48 b a sin a cos a cos d 

 

0 

a 

a 

[Put x = a sin , dx = a cos d] 

3 3 

4 /2 

2 2 

= 48 ba sin cos 

d 

4 

= 48 ba 

2 2 

0 

23 

1 1 

 

4 2 2 

= 48 ba 

= 3 b a 4 Ans. 

22 

Example 111. Evaluate surface integral Fnds ˆ , 

 

 

where F = (x 2 + y 2 + z 2 ) iˆ 

ˆj kˆ 

( ), S 

is the surface of the tetrahedron x = 0, y = 0, z = 0, x + y + z = 2 and n is the unit normal in 

the outward direction to the closed surface S. 

Solution. By Divergence theorem 

Fnds 

 

ˆ = div Fdv 

S V 

where S is the surface of tetrahedron x = 0, y = 0, z = 0, x + y + z = 2 

= 

 

 

 

i ˆ 

 

ˆ j k ˆ 2 2 2 

( x y z )( i ˆ ˆ j k ˆ) 

dv 

V 

 

x y z 

= (2x 2y 2 z) 

dv 

V 

 

= 2 ( x y z) 

dx dy dz 

= 

= 

V 

2 2x 2 x y 

 

2 dx dy ( x y z) 

dz 

2 

 

0 0 0 

2 

2 x 

y 

2 2 x 

z 

dx dy 

xz 

yz 

2 

 

 

0 0 

0

458 Vectors 

= 

 

2 

2 2 x 

2 2 (2 x 

y) 

 

dx dy x x xy y xy y 

0 

 

 

0 

2 2 2 

 

3 3 

2 

2 2 2 y (2 x 

y) 

 

= 2 

dx 2xy x y xy y 

 

0 

 

3 6 

2 

2 

x 

3 3 

2 

2 2 2 (2 x) (2 x) 

 

= 2 

dx 2 x(2 x) x (2 x) x (2 x) (2 x) 

 

0 

 

3 6 

3 3 

2 

2 2 3 2 3 2 (2 x) (2 x) 

 

= 2 

4x2x 2x x 4x 4 x x (2 x) 

 

0 

 

 

3 6 

2 

 

3 4 3 4 3 4 4 

2 4 2 4 (2 ) (2 ) (2 ) 

= 2 2 x x 2 

x x x x x 

x x 

3 4 3 4 3 12 24 

 

3 4 4 

(2 x) (2 x) (2 x) 

8 16 16 

= 2 = 2 

3 12 24 3 12 24 

 

= 4 Ans. 

0 

Example 112. Use the Divergence Theorem to evaluate 

 

S 

( xdydz y dz dx zdxdy) 

where S is the portion of the plane x + 2 y + 3 z = 6 which lies in the first Octant. 


 

Solution. ( f1 dydz f2 dx dz f3 

dxdy) 

S 

f1 f2 

f3 

 

= 

dxdydz 

V 

 

x y z 

 

where S is a closed surface bounding a volume V. 

 

( xdydz y dz dx zdxdy) 

S 

 

= 

V 

x y z 

dx dy dz 

x y z 

= 3 V 2 

= (1 11) 

dx dy dz dx dy dz 

V 

= 3 (Volume of tetrahedron OABC) 

1 

= 3[( Area of the base OAB) height OC] 

3 

1 1 

 

= 3 

63 

2 

3 2 

= 18 Ans. 

 

Example 113. Use Divergence Theorem to evaluate : ( xdydz y dz dx zdxdy) 

Solution. 

over the surface of a sphere radius a. 

Here, we have 

(K. University, Dec. 2009) 

xdydz y dx dz zdxdy 

 

S 

 

 

f1 f2 

f3 

 

x y z 

dx dy dz 

V 

 

dx dy dz 

x y z 

 

 

V 

x y z 

(1 + 1 + 1) dx dy dz = 3 (volume of the sphere) 

V 

4 

3 

= 3 a 

 

3 = 4 a3 Ans. 

 

0 

 

0

Vectors 459 

Example 114. Using the divergence theorem, evaluate the surface integral 

( yz dy dz zxdz dx xy dy dx) 

where S : x 2 + y 2 + z 2 = 4. 

S 

 

Solution. ( f1 dydz f2 dx dz f3 

dxdy) 

S 

 

f1 f2 

f3 

 

= 

dx dy dz 

v 

 

x y z 

where S is closed surface bounding a volume V. 

 

( yz dy dz zx dx dz xy dx dy) 

S 

(AMIETE, Dec. 2010, UP, I Sem., Dec 2008) 

( ) ( ) ( ) 

= yz zx xy 

dx dy dz 

v 

 

x y z 

= (0 0 0) dx dy dz 

v 

= 0 Ans. 

 

2 2 3 2 

Example 115. Evaluate xz dy dz ( x y z ) dzdx (2 xy y z) 

dxdy 

S 

where S is the surface of hemispherical region bounded by 

z = 

2 2 2 

a x y and z = 0. 

= 

Solution. ( f1 dydz f2 dz dx f3 

dxdy) 

S 

where S is a closed surface bounding a volume V. 

 

 

= 

S 

 

2 2 3 2 

xz dy dz ( x y z ) dzdx (2 xy y z) 

dxdy 

 

 

V 

V 

f1 f2 

f3 

 

dx dy dz 

x y z 

 

2 2 3 

2 

( xz ) ( x y z ) (2 xy y z) 

dxdydz 

x y z 

 

(Here V is the volume of hemisphere) 

2 2 2 

= ( z x y ) dx dy dz 

V 

Let x = r sin cos , y = r sin sin , z = r cos 

= 

= 


2 2 

r ( r sin dr d d ) 

= 

5 

a 

2 /2 

r 

 

( ) 0 ( cos ) 

0 

 

5 

 

0 

= 

 

2 a 

2 sin 

0 0 0 

 

d d r dr 

5 

2 ( 0 1) 5 

a 

= 

2a 

5 

4 

5 

Ans. 

F . n ˆ ds over the entire surface of the region above the xy-plane 

S 

bounded by the cone z 2 = x 2 + y 2 2 

and the plane z = 4, if F = 4xz iˆ 

xyz ˆj 3 zkˆ 

. 

Solution. If V is the volume enclosed by S, then V is bounded by the surfaces z = 0, z = 4, 

z 2 = x 2 + y 2 . 

By divergence theorem, we have 

= 

F . n ˆ ds 

S 

= 

= 

 

 

 

V 

V 

V 

 

div Fdxdydz 

2 

(4 xz) ( xyz ) (3) z dxdydz 

x y z 

 

2 

(4z xz 3) dxdydz 

Limits of z are 

2 2 

x y and 4.

460 Vectors 

4 2 

x 

2 

y 

2 

(4z xz 3) dzdydx = 

 

 

3 

2 xz 

2z 3z 

dy dx 

3 

4 

x 

2 

y 

2 

 

64x 

2 2 2 2 3/2 2 2 

= 32 12 {2( x y ) xx ( y ) 3 x y } dydx 

3 

 

 

 

 

64x 

2 2 2 2 3/2 2 2 

= 44 2( x y ) xx ( y ) 3 

x y dydx 

3 

 

Putting x = r cos and y = r sin , we have 

64r 

cos 2 3 

= 44 2r r cos r 3r 

rddr 

3 

 

Limits of r are 0 to 4. 

and limits of are 0 to 2 

= 

= 

= 

= 

= 

 

 

 

 

 

2 

2 4 64r 

cos 3 5 2 

44 2 cos 3 

 

0 

0 

 

 

r r r r ddr 

3 

 

3 4 6 

2 

2 64 r cos r r 

3 

0 

2 

0 

2 

0 

2 

0 

 

22r cos r d 

 

9 2 6 

3 4 6 

2 64 (4) cos (4) (4) 

3 

22(4) cos (4) 

d 

 

9 2 6 

 

6 

64 64 (4) 

352 cos 128 cos 64 

d 

9 6 

 

 

6 

64 64 (4) 

160 cos d 

 

 

9 6 

 

 

 

2 

 

6 

64 64 (4) 

 

6 

64 64 (4) 

= 160 

sin 

9 6 = 160 (2 ) sin 2 

 

 

 

 

9 6 

0 

 

 

= 320 Ans. 

Example 117. The vector field 2 

F x iˆ 

zj ˆ yzkˆ 

is defined over the volume of the cuboid 

given by 0 x a, 0 y b, 0 z c, enclosing the surface S. Evaluate the surface integral 

 

 

F . ds 


S 

Solution. By Divergence Theorem, we have 

2 ˆ 

2 

( x iˆ zj ˆ yz k). ds div ( x iˆ zj ˆ yz kˆ) dv, 

 

S 

 

v 

where V is the volume of the cuboid enclosing the surface S. 

 

ˆ 

2 

ˆ 

= 

ˆ 

 

ˆ 

 

i j k .( x iˆ z ˆ 

 

j yzk) 

dv 

v 

 

x y z 

2 

= ( x ) ( z) ( yz) 

dx dy dz 

v 

x y z 

= 

a b c 

= 

(2 x 

y ) dx dy dz 

0 0 0 

x y z 

a b a b 

c 

= 0 

0 0 0 0 

 

dx [2 xz yz] dy dx (2 xc yc) 

dy 

4 

0 

a b c 

 

dx dy (2 x y ) dz 

0 0 0

Vectors 461 

= 

= 

a b a b 

2 a 

2 

y b 

cdx(2 x y) dy c2xy dx c 2bx 

dx 

2 

2 

 

0 0 0 0 0 

2 2 

a 

2 

2 

2 

bx b x ab b 

c c ab abc a 

 

2 2 2 2 

0 

Example 118. Verify the divergence Theorem for the function F = 2 x2 yi – y 2 j + 4 x z 2 k 

taken over the region in the first octant bounded by y 2 + z 2 = 9 and x = 2. 

Solution. 

 

FdV 

= 

V 

 

= (4 xy 2y 8 xz) 

dxdydz 

= 

= 

 

ˆ 

 

ˆ 

ˆ 2 ˆ 2ˆ 

2 

i j k (2x yi y j 4 xz k) 

dV 

x y z 

= 

 

 

2 3 9 y 

2 

 

dx dy (4 xy 2y 8 xz) 

dz 

0 0 0 

2 3 9 

2 

2 y 

dx (4 2 4 ) 

0 dy xyz yz xz 

0 

0 

2 3 2 2 2 

 

dx [4 xy 9 y 2y 9 y 4 x(9 y )] dy 

0 0 

3 

2 4x 

2 2 3/2 2 2 3/2 4xy 

 

= dx (9 y ) (9 y ) 36 

xy 

0 

2 3 3 3 0 

2 

2 

= (0 0 108 x 36 x 36 x 18) dx 

2 

= (108 x 18) dx 

 

2 

x 

0 = 108 18 x 

0 

2 0 

= 216 – 36 = 180 ...(1) 

Here Fnds 

 

ˆ = Fnˆ ds Fnˆ ds Fnˆ ds Fnˆ ds F 

nˆ 

ds 

S 

OABC OCE OADE ABD BDEC 

F nˆ 

2 2 2 

ds (2 x yiˆ 

y ˆj 4 xzkˆ 

). nds ˆ 

= 

BDEC 

 

BDEC 

Normal vector 

 

= = ˆ 

 

i ˆ 

 

j kˆ 

 

 

x y z (y2 + z 2 – 9) 

= 2 yj ˆ 2 zkˆ 

2 yj ˆ 2zkˆ 

yj ˆ zkˆ 

Unit normal vector = ˆn = 

= 

2 2 2 2 

4y 

4z 

y z 

yj ˆ zkˆ 

yj ˆ zkˆ 

= = 

9 3 

ˆ 

2 ˆ 2ˆ 

2 yj zk 1 

(2x yi y j 4 xzk) 

ds 

3 3 

= ( 4 ) 

3 

BDEC 

3 

y xz ds 

BDEC 

 

 

 

yj ˆ zkˆ 

 

( ˆ ) ˆ z dxdy 

dx dy ds nk ds k ds or ds 

 

3 3 z 

 

 

 

3 

3 

1 

3 3 dxdy 

2 3 

y 2 

 

= ( y 4 xz ) 

3 

= dx 4 xz dy 

z 

0 

 

0 

z 

BDEC 

 

 

3 

3 

2 

27 sin 

2 

2 

 

= dx 

4 x (9cos ) 

0 

0 

3cos 

 

3 

Ans. 

y 

3sin , 

 

 

z 

3cos

462 Vectors 

= 

= 

F nˆ 

ds = 

OABC 

 

 

= 

2 2 2 

dx 27 108 x 

0 

 

3 3 

= 

2 

 

2 

0 

( 18 72 x ) dx 

 

2 

18x 

36 x 

 

 

 

= 108 ...(2) 

0 

2 2 2 

(2 x yiˆ y ˆj 4 xz kˆ) ( kˆ) 

ds 

 

OABC 

 

2 

4 xz ds = 0 ...(3) because in OABC xy-plane, z = 0 

OABC 

F nˆ 

ds = 

OADE 

 

 

Fnds ˆ 

= 

OCE 

 

 

Fnds ˆ = 

ABD 

 

 

= 

(2 ˆ ˆ 4 ) ( ˆ 

x yi y j xzkj) 

ds = 

OADE 

2 2 2 ˆ 

(2 ˆ ˆ 4 ) ( ˆ 

x yi y j xzki) 

ds = 

OCE 

2 2 2 ˆ 

(2 x yiˆ y ˆj 4 xzk) () iˆ 

ds = 

2 

2 x y ds 

ABD 

2 

2 x y dy dz = 

2 2 2 ˆ 

9 z 

2 

 

2 

y ds = 0 ...(4) 

OADE 

because in OADE xz-plane, y = 0 

2 

2 x y ds = 0 ...(5) 

OCE 

 

because in OCE yz-plane, x = 0 

ABD 

3 9 

z 

2 

2 

dz 2(2) ydy 

0 

because in ABD plane, x = 2 

0 

2 

3 

= 8 y 

 

dz 

0 

2 

= 

3 

3 

2 

z 

4 dz (9 z ) = 49z 

 

0 

 

3 

0 

 

On adding (2), (3), (4), (5) and (6), we get 

 

 

3 

0 

= 4 [27 – 9] = 72 ...(6) 

Fnds ˆ = 108 + 0 + 0 + 0 + 72 = 180 ...(7) 

S 


V 

 

FdV 

Fnds 

 

 

S 

 

= 

ˆ 

Hence the theorem is verified. 

Example 119. Verify the Gauss divergence Theorem for 

= (x 2 – yz) î + (y 2 – zx) ĵ + (z 2 – xy) ˆk taken over the rectangular parallelopiped 

0 x a, 0 y b, 0 z c. (U.P., I Semester, Compartment 2002) 

Solution. We have 

div F = F 

ˆ 

2 2 2 ˆ 

= 

ˆ 

 

ˆ 

 

i j k [( x yz) iˆ( y zx) ˆj ( z xy) k] 

x y z 

2 2 2 

= ( x yz) ( y zx) ( z xy) 

= 2x + 2y + 2z 

x y z 

F 

Volume integral = 

= 

= 

 

FdV 

= 2( x y z) 

dV 

V 

V 

a b c 

= 

2 ( x y z ) dx dy dz 

2 

x 0 y 0 z 

0 

 

dx dy 

xz yz 

 

2 

a b z 

0 0 

c 

 

2 

 

= 

0 

b 

2 2 

= 2 a y c y 

dx cxyc 

 

0 

2 2 

 

 

= 2 

0 

2 

 

 

a b c 

 

2 dx dy ( x y z) 

dz 

0 0 0 

 

dx dy 

cx cy 

 

2 

a b c 

0 0 

a bc bc 

dx bcx 

0 

 

2 2 

2 2 

 

2

Vectors 463 

a 

 

2 2 2 

bc x b cx bc x 

= 2 = [a 

2 2 2 

2 bc + ab 2 c + abc 2 ] 

0 

= abc (a + b + c) ...(A) 

 

 

To evaluate Fnds ˆ , where S consists of six plane surfaces. 

S 

 

 

= ˆ ˆ ˆ 

OABC 

DEFG OAFG 

Fnds ˆ 

S 

Fnds 

OABC 

 

ˆ = 

 

 

F nds ˆ 

DEFG 

 

Fnds Fnds Fnds 

 

 

Fnds ˆ Fnds ˆ Fnds ˆ 

= 

= 

= 

= 

= 

 

 

BCDE ABEF OCDG 

OABC 

ab 

 

00 

ab 

00 

2 2 2 

{( x yzi ) ˆ ( y xz) ˆj ( z xy) kˆ}( kˆ) 

dxdy 

2 

( z xy) 

dxdy 

(0 xy) 

dx dy = 

 

DEFG 

ab 

ab 

2 

( z xy) 

dxdy = 

 

00 

00 

a 

2 

b a 

 

2 xy 

c y dx 

2 

= 

0 0 

0 

 

2 2 

2 x b 

= cbx 

 

4 

Fnds 

 

 

OAFG 

 

ˆ = 

= 

= 

 

 

a 

OAFG 

c 

0 0 

 

ˆ 

2 

 

a 

0 

= 

OAFG 

( y zx) 

dxdz 

dx 

(0 zx) 

dz = 

2 2 

a b 

4 

...(1) 

2 2 2 

{( x yz) iˆ 

( y xz) ˆj ( z xy) kˆ}( kˆ) 

dxdy 

2 

( c xy) 

dxdy 

 

2 xb 

cb 

 

2 

2 2 

2 

 

dx 

 

 

2 a b 

abc ...(2) 

4 

{( x yz) iˆ ( y zx) ˆj ( z xy) k}( ˆj) 

dxdz 

2 2 2 ˆ 

a 

2 

xz 

dx 

 

 

2 

= 

 

c 

0 0 

a xc 

2 

dx = 

2 

0 

x c 

 

4 

F nds 2 2 2 

= {( x yz ) i ˆ ( y zx ) ˆ j ( z xy ) k ˆ} 

ˆ jdxdz 

BCDE = 

Fnds 

 

 

ABEF 

= 

 

a c 

a 

2 

c 

 

2 

2 xz 

dx ( b xz) 

dz = 

 

b z 

2 

0 0 

0 0 

2 2 

a 

2 2 

2 x c 2 ac 

2 2 

dx = 

 

 

 

a 

0 

a 

 

0 

= 

2 2 

a c 

4 

BCDE 

 

2 xc 

 

b c 

2 

2 

...(3) 

( y xz) 

dxdz 

2 

 

 

dx 

 

 

= b cx = ab c ...(4) 

4 

4 

0 

2 2 2 

 

ˆ 

ˆ 

= {( ) ˆ ( ) ˆ ( ) } ˆ 

x yz i y xz j z x y k idydz 

ABEF 

= 

2 

( x yz) 

dydz = 

ABEF 

S.No. 

Surface Outward normal 

ds 

1 OABC – k dx dy z = 0 

2 DEFG k dx dy z = c 

3 OAFG – j dx dz y = 0 

4 BCDE j dx dz y = b 

5 ABEF i dy dz x = a 

6 OCDG – i dy dz x = 0 

b c 

b 

2 

c 

2 

 

2 yz 

dy ( a yz) 

d z = dy 

 

a z 

2 

0 0 

0 0

464 Vectors 

Fnds 

 

 

OCDG 

= 

 

ˆ = 

= 

b 

 

2 

2 yc 

 

ac 

dy 

2 

0 

= 

 

OCDG 

 

2 2 

2 yc 

acy 

 

4 

2 2 2 ˆ 

b 

0 

= 

2 2 

2 b c 

a bc ...(5) 

4 

{( x yz) iˆ ( y zx) ˆj ( z x y) k} ( iˆ 

) dydz 

b c 

x 2 yz dydz 

= 

0 0 ( ) 

2 

b yc 

2 2 

y c 

= dy = = 

0 2 4 0 

Adding (1), (2), (3), (4), (5) and (6), we get 

b 

 

b 

c 

 

= 

dy ( yz ) dz 

0 0 

2 2 

b c 

4 

2 

b yz 

dy 

 

 

0 

2 

c 

0 

...(6) 

Fnds 

 

ˆ 

2 2 2 2 2 2 2 2 

2 2 

= 

a b abc a b a c ab c 

a c 

4 4 4 4 

 

 

2 2 2 2 

b c 

2 b c 

a bc 

4 4 

 

= abc 2 + ab 2 c + a 2 bc 

= abc (a + b + c) ...(B) 

From (A) and (B), Gauss divergence Theorem is verified. 

Verified. 

 

2 

Example 120. Verify Divergence Theorem, given that F 4 xziˆ 

– y ˆj yz kˆ 

and S is the 

surface of the cube bounded by the planes x = 0, x = 1, y = 0, y = 1, z = 0, z = 1. 

Solution. 

Volume Integral = 

F 

ˆ 

2 ˆ 

= 

ˆ 

 

ˆ 

 

i j k (4 zxiˆ y ˆj yzk) 

x y z 

= 4 z – 2 y + y 

= 4 z – y 

 

Fdv 

 

= (4 z y) 

dx dy dz 

= 

1 1 1 

 

dx dy (4 z y ) dz 

0 0 0 

= 

= 

1 dx 1 dy 2 1 

(2 z yz ) 

0 0 

0 

= 

 

2 

1 y 

dx 

2 y 

0 2 = 

 

1 

0 

 

1 1 

 

dx dy (2 y) 

0 0 

 

1 1 

dx 2 

 

0 

 

2 

= 3 1 3 ( ) 1 

0 

0 

2 dx x = 3 2 2 

...(1) 

To evaluate Fnds ˆ , where S consists of six plane surfaces. 

S 

 

 

Over the face OABC , z = 0, dz = 0, ˆn = – ˆk , ds = dx dy 

 

 

1 1 2 

F. nds ˆ (– y ˆj) (– kˆ 

) dxdy 0 

 

0 0 

Over the face BCDE, y = 1, dy = 0

Vectors 465 

 

 

Fnds ˆ = 

1 1 

 

0 0 

(4 xziˆ ˆj zkˆ 

) ( ˆj) 

dx dz 

ˆn = ˆ, j ds dxdz = 

= 

1 1 

dx dz = 

0 0 

1 1 

 

0 0 

dxdz 

1 1 

0 z 0 

( x) ( ) = – (1) (1) = – 1 

Over the face DEFG, z = 1, dz = 0, ˆn = ˆk , ds = dx dy 

 

 

Fnds ˆ = 

= 

1 1 2 

 

0 0 

[4 x (1) y ˆj y(1) kˆ]() 

kˆ 

dx dy 

1 1 

ydxdy = 

0 0 

1 1 

dx ydy = ( x) 

0 0 

Over the face OCDG, x = 0, dx = 0, ˆn = – iˆ, 

 

 

1 

0 

ds = dy dz 

Fnds ˆ = 1 1 ˆ 2 ˆ ˆ ˆ 

(0 i y j yzk ) ( i ) dydz = 0 

0 0 

Over the face AOGF, y = 0, dy = 0, ˆn = – ĵ , 

 

 

Fnds ˆ = 

1 1 

(4 ˆ) ( ˆ 

xzi j) 

dxdz = 0 

0 0 

Over the face ABEF, x = 1, dx = 0, ˆn = î , 

 

 

Fnds ˆ = 

1 1 2 

0 0 

[(4 zi ˆ y ˆ j yzk ˆ)()] 

i ˆ dydz 

= 

1 dy 

1 

4 

0 0 

1 2 1 

dy (2 z ) 

0 

0 

1 

 

2 

y 

 

2 

 

0 

ds = dx dz 

ds = dy dz 

1 

0 

= 1 2 

1 1 

4 zdydz 

0 0 

= zdz = = 2 dy = 2( y) 2 

On adding we see that over the whole surface 

Fnds 

1 

ˆ = 01 0 0 

2 

2 = 3 2 


1. Use Divergence Theorem to evaluate 

V 

 

Fdv 

Fnds 

 

 

S 

 

= 

ˆ 

EXERCISE 5.15 

 

2 2 2 2 2 

( y z i ˆ z x ˆ j x y 2ˆ k ). ds , 

s 

1 

0 

...(2) 

Verified. 

where S is the upper part of the sphere x 2 + y 2 + z 2 = 9 above xy- plane. Ans. 243 

8 

 

 

2. Evaluate ( F). ds, 

where S is the surface of the paraboloid x 2 + y 2 + z = 4 above the xy-plane and 

 

S 

2 ˆ ˆ 

2 

F ( x y 4) i 3 xyj (2 xz z ) kˆ 

. 

Ans. – 4 

 

2 2 3 2 

3. Evaluate [ xz dy dz ( x y z ) dzdx (2 xy y z) dxdy], 

where S is the surface enclosing a 

s 

region bounded by hemisphere x 2 + y 2 + z 2 = 4 above XY-plane. 

4. Verify Divergence Theorem for 2 

F x iˆ zj ˆ yzkˆ 

, taken over the cube bounded by 

x = 0, x = 1, y = 0, y = 1, z = 0, z = 1. 

2 

5. Evaluate (2 ˆ ˆ ˆ 

xyi yz j xz k) 

ds 

over the surface of the region bounded by 

S 

x = 0, y = 0, y = 3, z = 0 and x + 2 z = 6 Ans. 351 

2

466 Vectors 

6. 

 

Verify Divergence Theorem for 2 

F ( x y ) iˆ 

– 2 xj ˆ 2 yzkˆ 

and the volume of a tetrahedron bounded 

by co-ordinate planes and the plane 2 x + y + 2 z = 6. 

(Nagpur, Winter 2000, A.M.I.E.T.E.. Winter 2000) 

7. Verify Divergence Theorem for the function 

x 2 + y 2 = 9, z = 0 and z = 2. 

8. Use the Divergence Theorem to evaluate 

 

s 

ˆ ˆ 2 ˆ over the region bounded by 

F yi xj z k 

3 2 2 

x dydz x ydzdx x zdxdy, 

where S is the surface of the region bounded by the closed cylinder 

x 2 + y 2 = a 2 , (0 z b) and z = 0, z = b. 

 

Ans. 

4 

5 ab 

4 

2 

9. Evaluate the integral ( z x) dy dz xy dx dz 3 zdxdy, 

where S is the surface of closed region 

s 

bounded by z = 4 – y 2 and planes x = 0, x = 3, z = 0 by transforming it with the help of Divergence 

Theorem to a triple integral. Ans. 16 

10. Evaluate 

 

s 

ds 

2 2 2 2 2 2 

a x b y c z 

over the closed surface of the ellipsoid ax 2 + by 2 + cz 2 = 1 by 

applying Divergence Theorem. Ans. 

11. Apply Divergence Theorem to evaluate 2 2 2 

( lx my nz ) ds 

 

4 

( abc) 

taken over the sphere (x – a) 2 + (y – b) 2 + (z – c) 2 = r 2 , l, m, n being the direction cosines of the external 

normal to the sphere. (AMIETE June 2010, 2009) Ans. 

8 ( ) 

3 

3 a b c r 

12. Show that ( uV u 

V ) dv 

V 

 

 

= . 

s 

 

uV ds 

13. If E = grad and 2 

= 4 , prove that E 

n ds = 4 dv 

S 

V 

where n is the outward unit normal vector, while dS and dV are respectively surface and volume 

elements. 

Pick up the correct option from the following: 

14. If F 

is the velocity of a fluid particle then F. 

dr represents. 

(a) Work done (b) Circulation 

C 

(c) Flux (d) Conservative field. 

(U.P. Ist Semester, Dec 2009) Ans. (b) 

15. If f = ax i by j cz k , a, b, c, constants, then f. 

dS where S is the surface of a unit sphere is 

 

(a) ( ) 

3 a b c (b) 4 ( a b c) 

(c) 2 ( a b c) 

(d) (a + b + c) 

3 

(U.P., Ist Semester, 2009) Ans. (b) 

16. A force field F is said to be conservative if 

 

(a) Curl F 0 (b) grad F 0 (c) Div F 0 (d) Curl (grad F ) = 0 

(AMIETE, Dec. 2006) Ans. (a) 

17. The line integral 

 

 

2 2 

x dx y dy, where C is the boundary of the region x 2 + y 2 < a 2 equals 

c 

(a) 0, (b) a (c) a 2 1 2 

(d) a 

2 

(AMIETE, Dec. 2006) Ans. (b)

vector

You also want an ePaper? Increase the reach of your titles

Delete template?

Save as template?